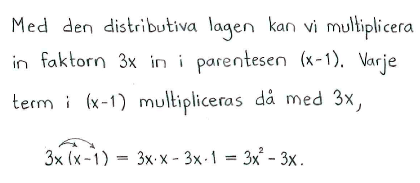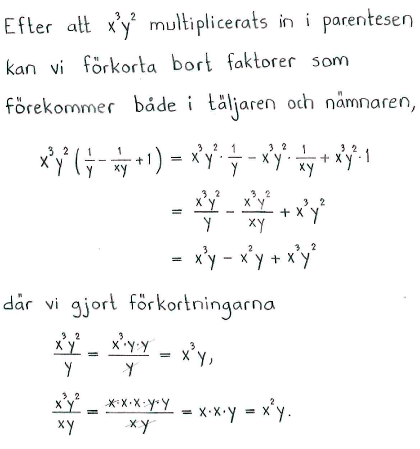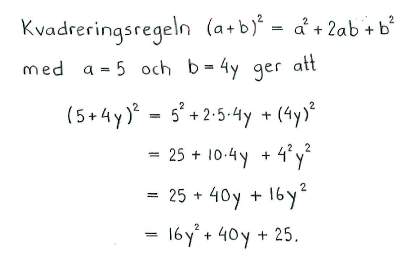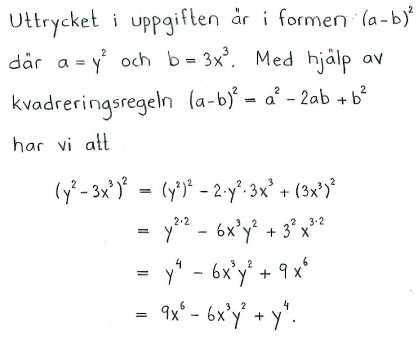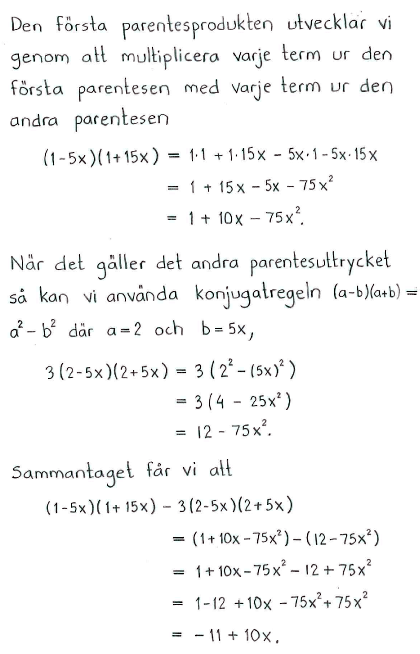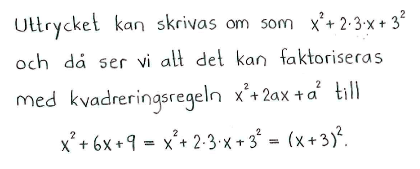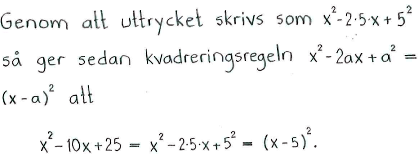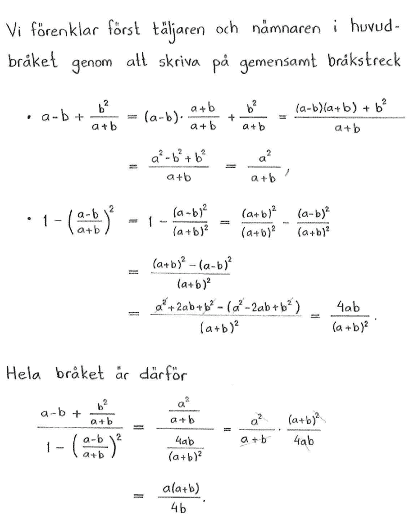2.1 Övningar
Sommarmatte 1
| Versionen från 27 april 2007 kl. 17.01 (redigera) Ossiang (Diskussion | bidrag) (→Övning 2.1:6) ← Gå till föregående ändring |
Versionen från 27 april 2007 kl. 17.08 (redigera) (ogör) Ossiang (Diskussion | bidrag) (→Övning 2.1:6) Gå till nästa ändring → |
||
| Rad 474: | Rad 474: | ||
| <div class=NavContent> | <div class=NavContent> | ||
| Facit till alla delfrågorna<br \> | Facit till alla delfrågorna<br \> | ||
| + | <table width="100%" cellspacing="10px"> | ||
| + | <tr align="left"> | ||
| + | <td class="ntext">a)</td> | ||
| + | <td class="ntext">2y</td> | ||
| + | <td class="ntext">b)</td> | ||
| + | <td class="ntext">\displaystyle-\frac{-x+12}{(x-2)(x+3)}</td> | ||
| + | </tr> | ||
| + | <tr align="left"> | ||
| + | <td class="ntext">c)</td> | ||
| + | <td class="ntext">\displaystyle\frac{b}{a(a-b)}</td> | ||
| + | <td class="ntext">d)</td> | ||
| + | <td class="ntext">\displaystyle\frac{a(a+b)}{4b}</td> | ||
| + | </tr> | ||
| + | </table> | ||
| </div> | </div> | ||
| </div> | </div> | ||
Versionen från 27 april 2007 kl. 17.08
Innehåll[göm] |
Övning 2.1:1
Utveckla
| a) | 3x(x-1) | b) | (1+x-x^2)xy | c) | -x^2(4-y^2) | d) | x^3y^2\left(\displaystyle \frac{1}{y} - \frac{1}{xy}+1\right) |
| e) | (x-7)^2 | f) | (5+4y)^2 | g) | (y^2-3x^3)^2 | h) | (5x^3+3x^5)^2 |
Facit till alla delfrågorna
| a) | 3x^2-3x | b) | xy+x^2y-x^3y | c) | -4x^2+x^2y^2 | d) | x^3y-x^2y+x^3y^2 |
| e) | x^2-14x+49 | f) | 16y^2+40y+25 | g) | 9x^6-6x^3y^2+y^4 | h) | 9x^{10}+30x^8+25x^6 |
Lösning till delfråga a
Lösning till delfråga b
Lösning till delfråga c
Lösning till delfråga d
Lösning till delfråga e
Lösning till delfråga f
Lösning till delfråga g
Lösning till delfråga h
Övning 2.2:2
Utveckla och förenkla så långt som möjligt
| a) | (x-4)(x-5)-3x(2x-3) | b) | (1-5x)(1+15x)-3(2-5x)(2+5x) |
| c) | (3x+4)^2-(3x-2)(3x-8) | d) | (3x^2+2)(3x^2-2)(9x^4+4) |
| e) | (a+b)^2+(a-b)^2 | ||
Facit till alla delfrågorna
| a) | -5^2+20 | b) | -11+10x |
| c) | 54x | d) | 81x^8-16 |
| e) | 2a^2+2b^2 | ||
Lösning till delfråga a 
Lösning till delfråga b 
Lösning till delfråga c 
Lösning till delfråga d 
Lösning till delfråga e 
Övning 2.1:3
Faktorisera så långt som möjligt
| \textrm{a) } | x^2-36 | \textrm{b) } | 5x^2-20 |
| \textrm{c) } | x^2+6x+9 | \textrm{d) } | x^2-10x+25 |
| \textrm{e) } | 18x-2x^3 | \textrm{f) } | 16x^2+8x+1 |
Facit till alla delfrågorna
| \textrm{a) } | (x+6)(x-6) | \textrm{b) } | 5(x+2)(x-2) |
| \textrm{c) } | (x+3)^2 | \textrm{d) } | (x-5)^2 |
| \textrm{e) } | -2x(x+3)(x-3) | \textrm{f) } | (4x+1)^2 |
Lösning till delfråga a
Lösning till delfråga b
Lösning till delfråga c
Lösning till delfråga d
Lösning till delfråga e
Lösning till delfråga f
Övning 2.1:4
Bestäm koefficienterna framför x och x^2 när följande uttryck utvecklas
| a) | (x+2)(3x^2-x+5) |
| b) | (1+x+x^2+x^3)(2-x+x^2+x^4) |
| c) | (x-x^3+x^5)(1+3x+5x^2)(2-7x^2-x^4) |
Facit till alla delfrågorna
| a) | 5 framför x^2, 3 framför x |
| b) | 2 framför x^2, 1 framför x |
| \textrm{c) } | 6 framför x^2, 2 framför x |
Lösning till delfråga 
Lösning till delfråga 
Lösning till delfråga 
Övning 2.1:5
Förenkla så långt som möjligt
| \textrm{a) } | \displaystyle \frac{1}{x-x^2}-\displaystyle \frac{1}{x} | \textrm{b) } | \displaystyle \frac{1}{y^2-2y}-\displaystyle \frac{2}{y^2-4} |
| \textrm{c) } | \displaystyle \frac{(3x^2-12)(x^2-1)}{(x+1)(x+2)} | \textrm{d) } | \displaystyle \frac{(y^2+4y+4)(2y-4)}{(y^2+4)(y^2-4)} |
Facit till alla delfrågorna
| a) | \displaystyle \frac{1}{1-x} | b) | -\displaystyle \frac{1}{y(y+2)} |
| c) | 3(x-2)(x-1) | d) | \displaystyle \frac{2(y+2)}{y^2+4} |
Lösning till delfråga a
Lösning till delfråga b
Lösning till delfråga c
Lösning till delfråga d
Övning 2.1:6
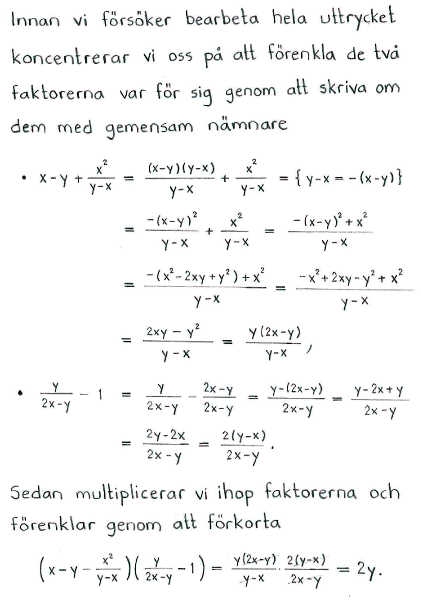
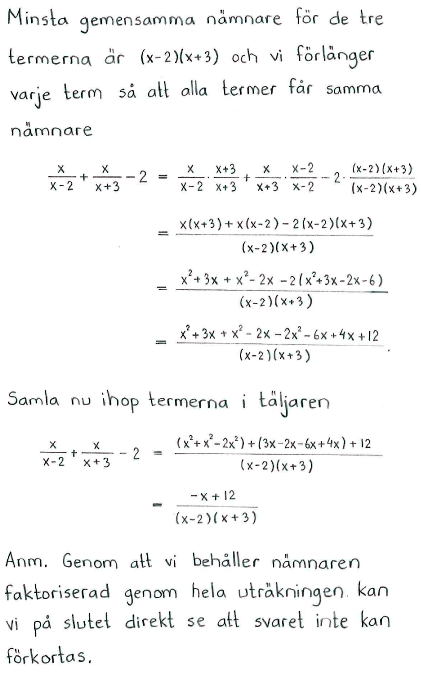
| a) | \left(x-y+\displaystyle\frac{x^2}{y-x}\right) \left(\displaystyle\frac{y}{2x-y}-1\right) | b) | \displaystyle \frac{x}{x-2}+\displaystyle \frac{x}{x+3}-2 |
| c) | \displaystyle \frac{2a+b}{a^2-ab}-\frac{2}{a-b} | d) | \displaystyle\frac{a-b+\displaystyle\frac{b^2}{a+b}}{1-\left(\displaystyle\frac{a-b}{a+b}\right)^2} |
Facit till alla delfrågorna
| a) | 2y | b) | \displaystyle-\frac{-x+12}{(x-2)(x+3)} |
| c) | \displaystyle\frac{b}{a(a-b)} | d) | \displaystyle\frac{a(a+b)}{4b} |
Lösning till delfråga a
Lösning till delfråga b
Lösning till delfråga c
Lösning till delfråga d
