Processing Math: Done
To print higher-resolution math symbols, click the
Hi-Res Fonts for Printing button on the jsMath control panel.
jsMath

Lösning 4.2:6

FörberedandeFysik

(Skillnad mellan versioner)
Hoppa till: navigering, sök
(Ny sida: Kirchhoffs spänningslag: <math>E-R_I\cdot I+E-R_I\cdot I+E-R_I\cdot I-U_L=0</math><br\> <math>3E-3R_I\cdot I-U_L=0</math><br\> <math>3E=5,0 V</math><br\> <math>U_L=3,9V,I_L=1,0A \Righta...)
Rad 3: Rad 3:
<math>3E=5,0 V</math><br\>
<math>3E=5,0 V</math><br\>
<math>U_L=3,9V,I_L=1,0A \Rightarrow 5,0-3R_I-3,9=0 \Rightarrow 3R_I=1,1\Omega </math><br\>
<math>U_L=3,9V,I_L=1,0A \Rightarrow 5,0-3R_I-3,9=0 \Rightarrow 3R_I=1,1\Omega </math><br\>
 +
Effektutvecklingen i batteriet är <math>3R_I\cdot I^2=1,1W </math><br\>
Effektutvecklingen i batteriet är <math>3R_I\cdot I^2=1,1W </math><br\>
Effektutvecklingen i lysdioden är <math>U_L\cdot I_L=3,9W</math>
Effektutvecklingen i lysdioden är <math>U_L\cdot I_L=3,9W</math>

Versionen från 22 januari 2010 kl. 12.21

Kirchhoffs spänningslag: E−RII+E−RII+E−RII−UL=0
3E−3RII−UL=0
3E=50V
UL=39VIL=10A50−3RI−39=03RI=11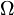

Effektutvecklingen i batteriet är 3RII2=11W
Effektutvecklingen i lysdioden är ULIL=39W

Den här artikeln är hämtad från http://wiki.math.se/wikis/forberedandefysik/index.php/L%C3%B6sning_4.2:6