Processing Math: Done
Lösning 4.1:6
FörberedandeFysik
(Skillnad mellan versioner)
| (3 mellanliggande versioner visas inte.) | |||
| Rad 1: | Rad 1: | ||
| - | <math>F = K\cdot \frac{Q_1\cdot Q_2}{r^2}<math> <br\> | + | <math>F = K\cdot \frac{Q_1\cdot Q_2}{r^2}</math> <br\> |
| - | där <math>Q_1=Q_2</math> och <math>r = 0,10 m</math>, <math>k = 9,0\cdot 10^9 Nm^2/C^2</math> och <math>F = 15 N</math> | + | där |
| + | <math>Q_1</math> = <math>Q_2</math> och <math>r = 0,10 \,\mathrm{m}</math>, <math>k = 9,0\cdot 10^9 \,\mathrm{Nm}^2/\,\mathrm{C}^2</math> och <math>F = 15 \,\mathrm{N}</math> | ||
| - | <math>Q_1 = Q_2= r\sqrt{\frac{F}{k}} = 4,1\mu C</math> | + | <math>Q_1 = Q_2= r\sqrt{\frac{F}{k}} = 4,1 \,\mathrm{\mu C}</math> |
Nuvarande version
 r2Q1
r2Q1 Q2
Q2
där
 10m
10m 0
0 109Nm2
109Nm2 C2
C2
 kF=4
kF=4 1
1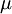 C
C
