Lösning 1.6:6
FörberedandeFysik
| (En mellanliggande version visas inte.) | |||
| Rad 4: | Rad 4: | ||
där<br\> | där<br\> | ||
| - | <math>p</math> är trycket i <math>Pa</math><br\> | + | <math>p</math> är trycket i <math>\mathrm{Pa}</math><br\> |
| - | <math>V</math> är volymen i <math>m^3</math><br\> | + | <math>V</math> är volymen i <math>\mathrm m^3</math><br\> |
| - | <math>n</math> är antal <math>kmol</math><br\> | + | <math>n</math> är antal <math>\mathrm{kmol}</math><br\> |
| - | <math>R</math> är allmänna gaskonstanten <math>8314 J/(kmol\cdot K)</math><br\> | + | <math>R</math> är allmänna gaskonstanten <math>8314 \,\mathrm{J/(kmol\cdot K)}</math><br\> |
| - | <math>T</math> är absoluta temperaturen i <math>K</math> | + | <math>T</math> är absoluta temperaturen i <math>\mathrm K</math> |
och | och | ||
| Rad 22: | Rad 22: | ||
<math>m=\frac{p\cdot V\cdot M}{R\cdot T}</math> | <math>m=\frac{p\cdot V\cdot M}{R\cdot T}</math> | ||
| - | <math>m=\frac{100\cdot 10^3(Pa)\cdot 10\cdot 10^{-3}(m^3)\cdot 4(kg/kmol)}{8314(J/K\cdot kmol)\cdot 293K}=0,00164kg</math> | + | <math>m=\mathrm{\frac{100\cdot 10^3(Pa)\cdot 10\cdot 10^{-3}(m^3)\cdot 4(kg/kmol)}{8314(J/K\cdot kmol)\cdot 293K}=0,00164kg}</math> |
<math>m</math> är heliumets massa<br\> | <math>m</math> är heliumets massa<br\> | ||
<math>M</math> är heliums molekylvikt<br\> | <math>M</math> är heliums molekylvikt<br\> | ||
| - | <math>M</math> för helium är <math> | + | <math>M</math> för helium är <math>4\,\mathrm{kg/kmol}</math><br\> |
| - | <math>T</math> för <math>20^\circ C</math> är <math> | + | <math>T</math> för <math>20^\circ \mathrm C</math> är <math>293 \,\mathrm K</math><br\> |
| - | <math>p</math> är <math>100\cdot 10^3 Pa</math><br\> | + | <math>p</math> är <math>100\cdot 10^3 \,\mathrm{Pa}</math><br\> |
| - | <math>V</math> är <math>10\cdot 10^{-3}m^3</math> (<math>10</math> liter) | + | <math>V</math> är <math>10\cdot 10^{-3}\,\mathrm m^3</math> (<math>10</math> liter) |
| - | <math> | + | <math>\rho=m_{\mathrm{luft}}/V</math><br\> |
| - | <math>m_{luft}= | + | <math>m_{\mathrm{luft}}=\rho\cdot V</math><br\> |
| - | <math>m_{luft}=1,19·10·10-3=0, | + | <math>m_{\mathrm{luft}}=1,19·10·10-3=0,0119 \,\mathrm{kg}</math><br\> |
| - | <math> | + | <math>\rho_{\mathrm{luft}}=1,1910^{-4} V</math> är <math>10\cdot 10^{-3}\,\mathrm m^3</math> (<math>10</math> liter) |
| - | Ballongen väger <math>2,4 g</math> vilket tillsammans med helium blir blir <math>4,04 g</math>. Tyngden för ballongen är <math>m\cdot g=0,040 N</math>. | + | Ballongen väger <math>2,4 \,\mathrm g</math> vilket tillsammans med helium blir blir <math>4,04 \,\mathrm g</math>. Tyngden för ballongen är <math>m\cdot g=0,040 \,\mathrm N</math>. |
| - | Den undanträngda luftmassan är <math>11,9 g</math> ger lyftkraften <math>m_{luft}\cdot g=0, | + | Den undanträngda luftmassan är <math>11,9 \,\mathrm g</math> ger lyftkraften <math>m_{\mathrm{luft}}\cdot g=0,117 \,\mathrm N</math> |
Lyftkraften minus ballongens tyngd ger den resulterande kraften. | Lyftkraften minus ballongens tyngd ger den resulterande kraften. | ||
| - | <math>F=m_{luft}\cdot g-m\cdot g = 0,117-0,040=0, | + | <math>F=m_{\mathrm{luft}}\cdot g-m\cdot g = 0,117-0,040=0,077 \,\mathrm N</math> |
Nuvarande version
Ideala gaslagen används.
 V=nRT
V=nRT
där
 (kmol
(kmol K)
K)
och
 M
M
det vill säga
 V=(m
V=(m M)RT
M)RT
vilket kan skrivas som
 Tp
Tp V
V M
M
 K
K kmol)
kmol) 293K100
293K100 103(Pa)
103(Pa) 10
10 10−3(m3)
10−3(m3) 4(kg
4(kg kmol)=0
kmol)=0 00164kg
00164kg
 kmol
kmol
 C
C
 103Pa
103Pa
 10−3m3
10−3m3
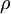 =mluft
=mluft V
V
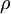
 V
V
 19·10·10−3=0
19·10·10−3=0 0119kg
0119kg
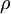 luft=1
luft=1 1910−4V
1910−4V 10−3m3
10−3m3
Ballongen väger \displaystyle 2,4 \,\mathrm g vilket tillsammans med helium blir blir \displaystyle 4,04 \,\mathrm g. Tyngden för ballongen är \displaystyle m\cdot g=0,040 \,\mathrm N. Den undanträngda luftmassan är \displaystyle 11,9 \,\mathrm g ger lyftkraften \displaystyle m_{\mathrm{luft}}\cdot g=0,117 \,\mathrm N
Lyftkraften minus ballongens tyngd ger den resulterande kraften.
\displaystyle F=m_{\mathrm{luft}}\cdot g-m\cdot g = 0,117-0,040=0,077 \,\mathrm N
