Lösning 5.5:3
FörberedandeFysik
(Skillnad mellan versioner)
(Ny sida: a) <math>\mathrm{Q = (M_{2H} + M_{3H} –\, M_{He} + m_n)c^2}</math> <math>\mathrm{(M_{2H})c^2 = (M_H + m_n)c^2 – 2\cdot 1{,}16\, MeV}</math> <math>\mathrm{(M_{3H})c^2 = (M_H + 2m_n)c^2...) |
(Ny sida: a) <math>\mathrm{Q = (M_{2H} + M_{3H} –\, M_{He} + m_n)c^2}</math> <math>\mathrm{(M_{2H})c^2 = (M_H + m_n)c^2 – 2\cdot 1{,}16\, MeV}</math> <math>\mathrm{(M_{3H})c^2 = (M_H + 2m_n)c^2...) |
Nuvarande version
a) \displaystyle \mathrm{Q = (M_{2H} + M_{3H} –\, M_{He} + m_n)c^2}
\displaystyle \mathrm{(M_{2H})c^2 = (M_H + m_n)c^2 – 2\cdot 1{,}16\, MeV}
\displaystyle \mathrm{(M_{3H})c^2 = (M_H + 2m_n)c^2 – 3\cdot 2{,}83\, MeV}
\displaystyle \mathrm{(M_{He})c^2 = (2M_H + 2m_n)c^2 – 4\cdot 7{,}07\, MeV}
vilket ger \displaystyle \textrm{Q} = -2\cdot 1{,}16 -3\cdot 2{,}83 - (-7\cdot 7{,}07) = 17{,}47 \textrm{ MeV}
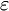b) \displaystyle \mathrm{U = e^2/4\pi \varepsilon_0(R_{2H} + R_{3H}) = (R = R_0\, A^{1/3},\, R_0 = 1,4\, fm) = 380\, keV}
