Processing Math: Done
Lösning 5.4:5
FörberedandeFysik
(Skillnad mellan versioner)
Louwah (Diskussion | bidrag)
(Ny sida: a) <math>\displaystyle \frac{1}{\lambda} = R\,\left( \displaystyle \frac{1}{n^2} - \displaystyle \frac{1}{k^2} \right)\,\,\lambda </math> är våglängden, <math>n</math> och <math>k</math>...)
Gå till nästa ändring →
Versionen från 13 december 2017 kl. 13.49
a) 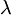 1=R
1=R 1n2−1k2
1n2−1k2
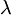
elektronhoppen sker.R är Rydbergs konstant,1 .097
107m−1
b) I Balmerserien gäller att  4
4 5
5




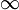
Våglängden fås ur 
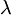 =R
=R 4
4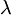 =4
=4 R=4
R=4 1
1 097
097 107=364nm
107=364nm
c) Längsta våglängden fås då 
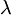 =R
=R 22–R
22–R 32
32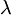 =36
=36 5R=36
5R=36 (5
(5 1
1 097
097 107)=656nm
107)=656nm
d) Om
