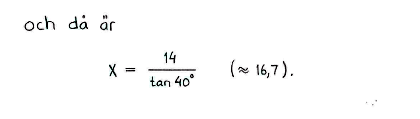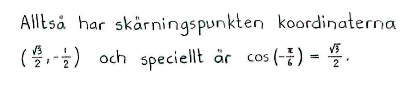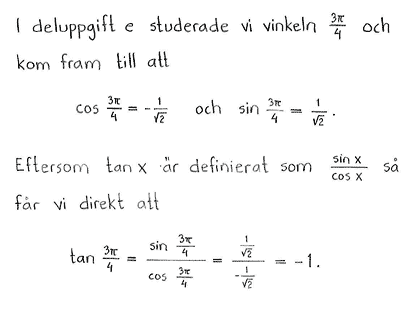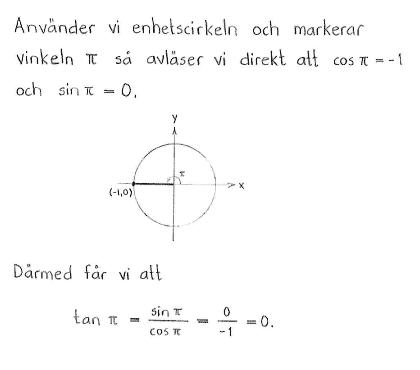4.2 Övningar
Sommarmatte 1
Innehåll |
Övning 4.2:1
Bestäm längden av sidan som är markerad med $x$ uttryckt med hjälp av de trigonometriska funktionerna.
| a) | BILD | b) | BILD | c) | BILD | d) | BILD | e) | BILD | f) | BILD |
Facit till alla delfrågorna
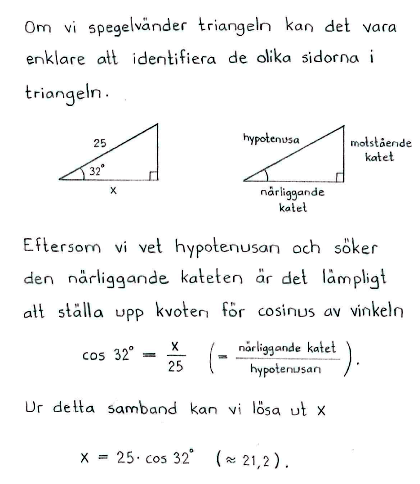
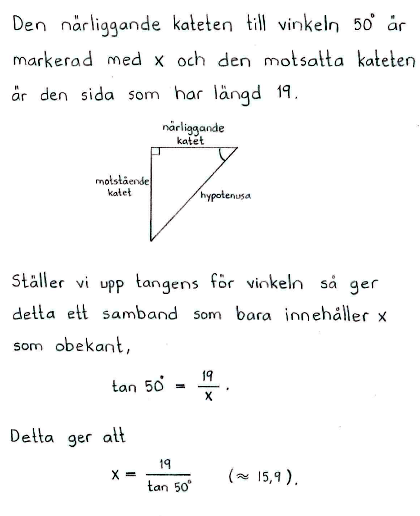
| a) | $x=13\cdot\tan {27 ^\circ} \approx 6,62$ | b) | $x=25\cdot\cos {32 ^\circ} \approx 21,2$ | c) | $x=\displaystyle\frac{14}{\tan {40 ^\circ}} \approx 16,7$ |
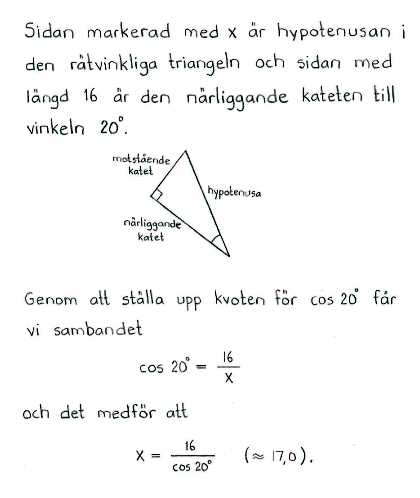
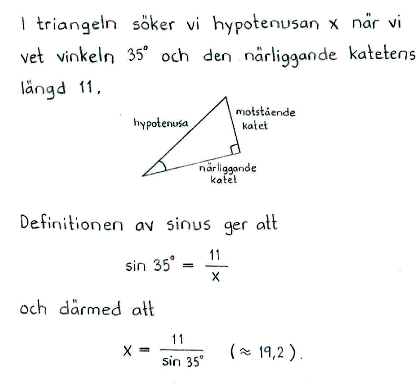
| d) | $x=\displaystyle\frac{16}{\cos {20 ^\circ}} \approx 17,0$ | e) | $x=\displaystyle\frac{11}{\sin {35 ^\circ}} \approx 19,2$ | f) | $x=\displaystyle\frac{19}{\tan {50 ^\circ}} \approx 15,9$ |
Lösning till delfråga a
Lösning till delfråga b
Lösning till delfråga c
Lösning till delfråga d
Lösning till delfråga e
Lösning till delfråga f
Övning 4.2:2
Bestäm en trigonometrisk ekvation dom vinkeln $v$ uppfyller.
| a) | BILD | b) | BILD | c) | BILD | d) | BILD | e) | BILD | f) | BILD |
Facit till alla delfrågorna
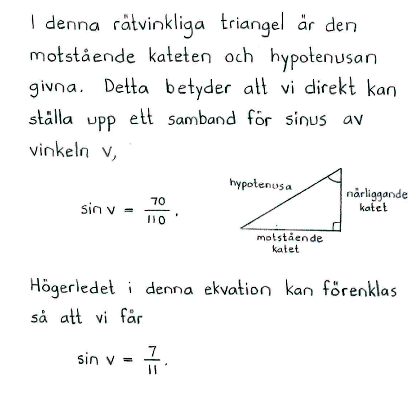
| a) | $\tan v=\displaystyle\frac{2}{5}$ | b) | $\sin v=\displaystyle\frac{7}{11}$ |
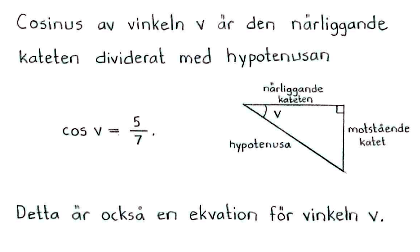
| c) | $\cos v=\displaystyle\frac{5}{7}$ | d) | $\sin v=\displaystyle\frac{3}{5}$ |
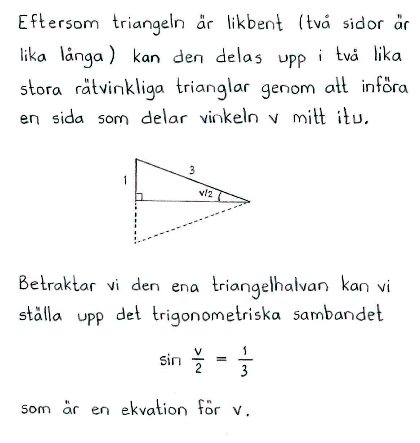
| e) | $v=30 ^\circ$ | f) | $\sin \displaystyle\frac{v}{2}=\displaystyle\frac{1}{3}$ |
Lösning till delfråga a
Lösning till delfråga b
Lösning till delfråga c
Lösning till delfråga d
Lösning till delfråga e
Lösning till delfråga f
Övning 4.2:3
Bestäm
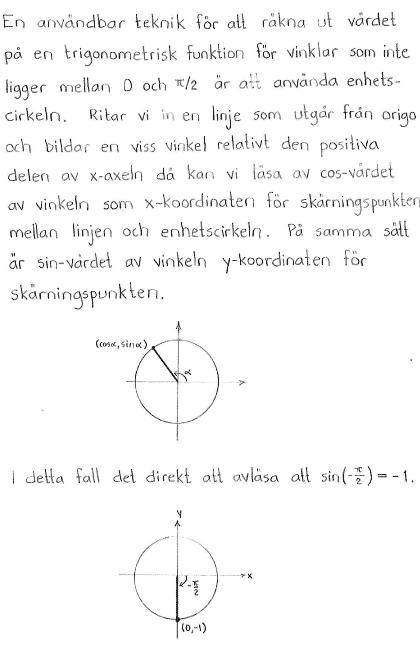
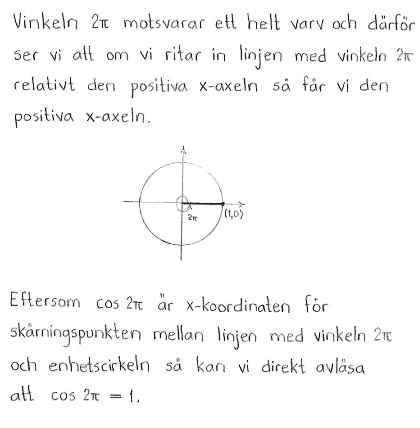
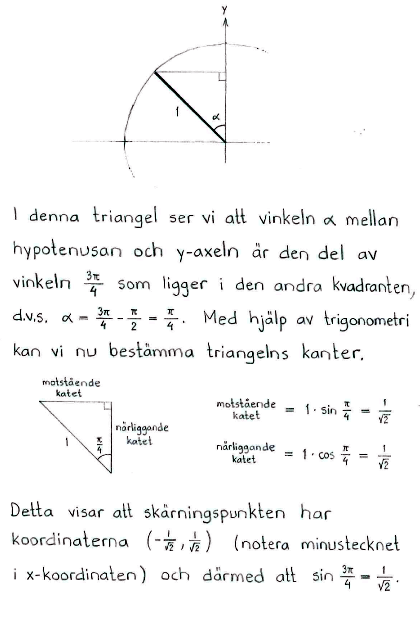
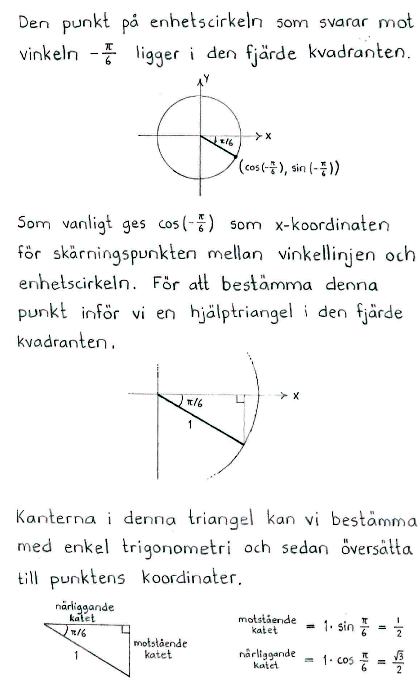
| $\textrm{a) }$ | $\sin{\left(-\displaystyle \frac{\pi}{2}\right)}$ | $\textrm{b) }$ | $\cos{2\pi}$ | $\textrm{c) }$ | $\sin{9\pi}$ |
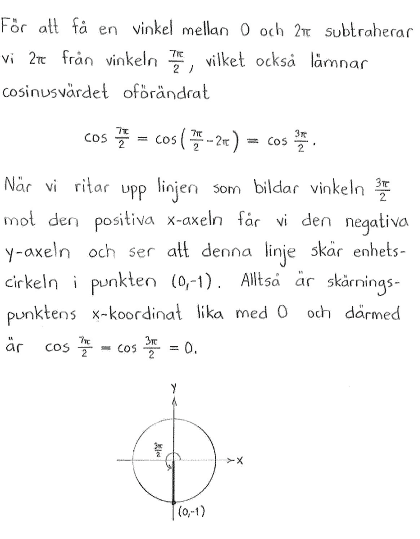
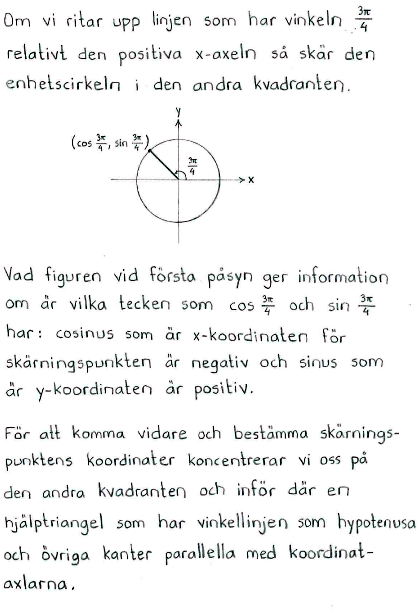
| $\textrm{d) }$ | $\cos{\displaystyle \frac{7\pi}{2}}$ | $\textrm{e) }$ | $\sin{\displaystyle \frac{3\pi}{4}}$ | $\textrm{f) }$ | $\cos{\left(-\displaystyle \frac{\pi}{6}\right)}$ |
Facit till alla delfrågorna
| $\textrm{a) }$ | $-1$ | $\textrm{b) }$ | $1$ | $\textrm{c) }$ | $0$ |
| $\textrm{d) }$ | $0$ | $\textrm{e) }$ | $\displaystyle \frac{1}{\sqrt{2}}$ | $\textrm{f) }$ | $\displaystyle \frac{\sqrt{3}}{2}$ |
Lösning till delfråga a
Lösning till delfråga b
Lösning till delfråga c
Lösning till delfråga d
Lösning till delfråga e
Lösning till delfråga f
Övning 4.2:4
Bestäm
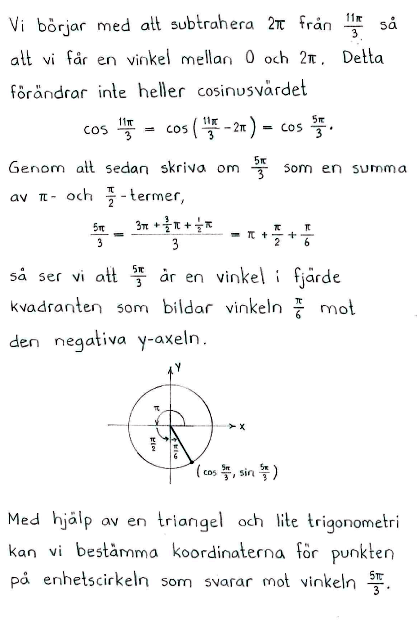
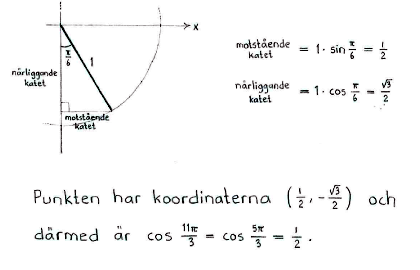
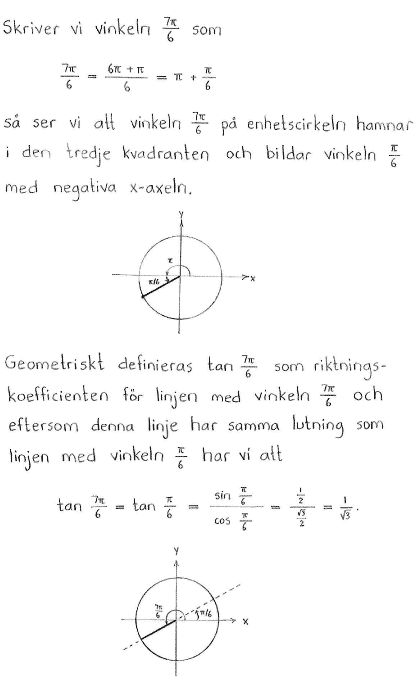
| a) | $\cos{\displaystyle \frac{11\pi}{6}}$ | b) | $\cos{\displaystyle \frac{11\pi}{3}}$ | c) | $\tan{\displaystyle \frac{3\pi}{4}}$ |
| d) | $\tan{\pi}$ | e) | $\tan{\displaystyle \frac{7\pi}{6}}$ | f) | $\tan{\left(-\displaystyle \frac{5\pi}{3}\right)}$ |
Facit till alla delfrågorna
| a) | $\displaystyle \frac{\sqrt{3}}{2}$ | b) | $\displaystyle \frac{1}{2}$ | c) | $-1$ |
| d) | $0$ | e) | $\displaystyle \frac{1}{\sqrt{3}}$ | f) | $\sqrt{3}$ |
Lösning till delfråga a
Lösning till delfråga b
Bild:4 2 4b.gif
Lösning till delfråga c
Lösning till delfråga d
Lösning till delfråga e
Lösning till delfråga f
