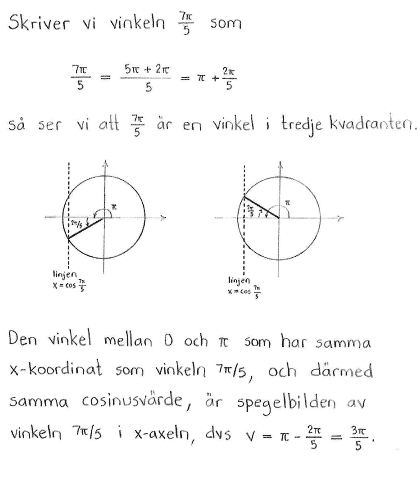
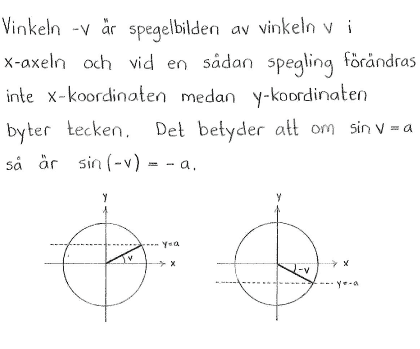
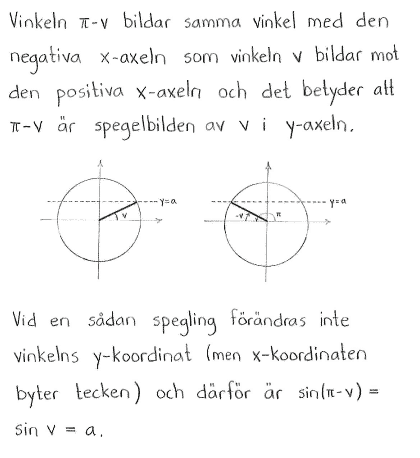
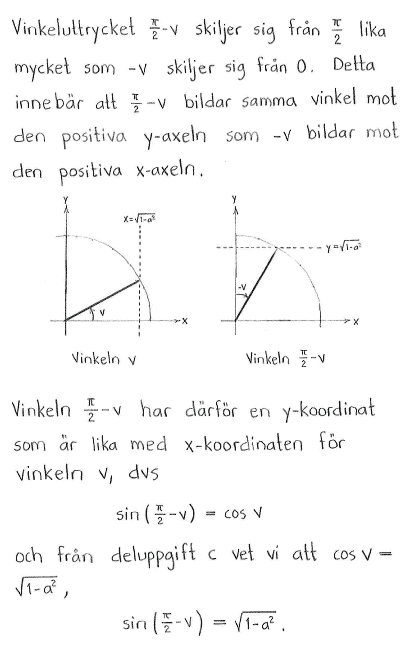
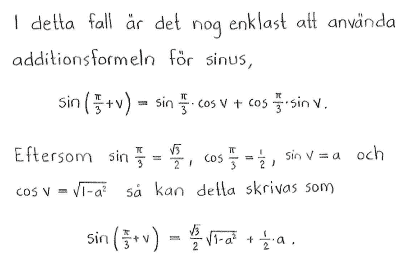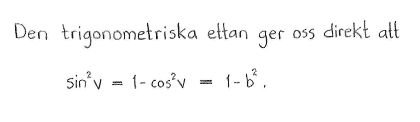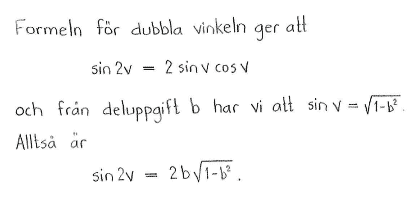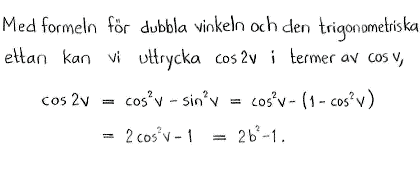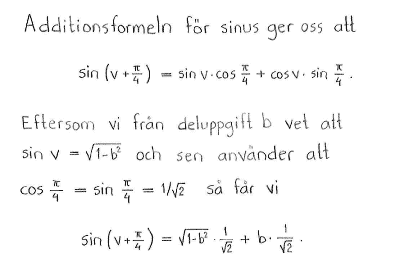4.3 Övningar
Sommarmatte 1
Innehåll[göm] |
Övning 4.3:1
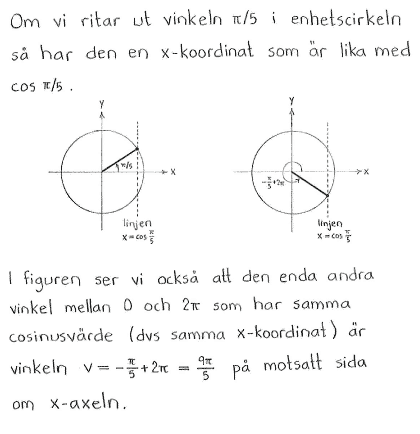
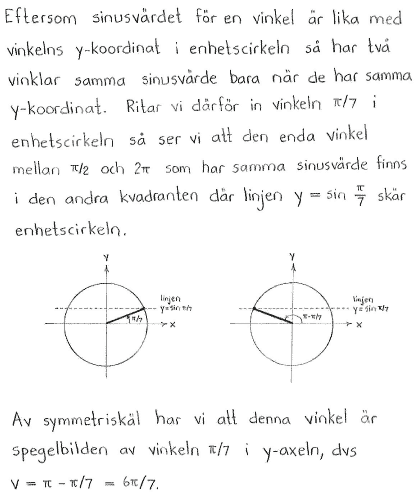
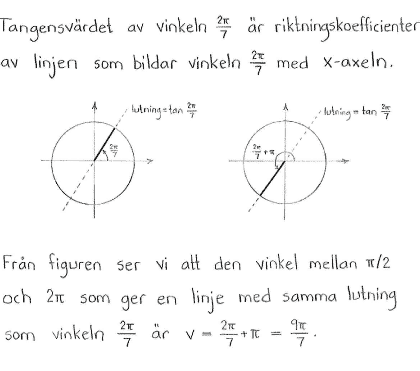
Bestäm de vinklar v mellan \displaystyle \frac{\pi}{2} och 2\pi som uppfyller
| \textrm{a) } | \cos{v}=\cos{\displaystyle \frac{\pi}{5}} | \textrm{b) } | \sin{v}=\sin{\displaystyle \frac{\pi}{7}} | \textrm{c) } | \tan{v}=\tan{\displaystyle \frac{2\pi}{7}} |
Facit till alla delfrågorna
| \textrm{a) } | v = \displaystyle \frac{9\pi}{5} | \textrm{b) } | v = \displaystyle \frac{6\pi}{7} | \textrm{c) } | v = \displaystyle \frac{9\pi}{7} |
Lösning till delfråga a
Lösning till delfråga b
Lösning till delfråga c
Övning 4.3:2
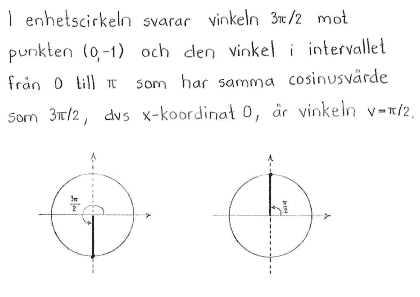
Bestäm de vinklar v mellan 0 och \pi som uppfyller
| \textrm{a) } | \cos{v} = \cos{\displaystyle \frac{3\pi}{2}} | \textrm{b) } | \cos{v} = \cos{ \displaystyle \frac{7\pi}{5}} |
Facit till alla delfrågorna
| \textrm{a) } | v=\displaystyle \frac{\pi}{2} | \textrm{b) } | v=\displaystyle \frac{3\pi}{5} |
Lösning till delfråga a
Lösning till delfråga b
Övning 4.3:3
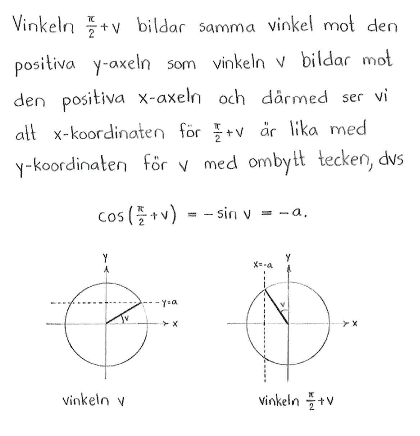
Antag att -\displaystyle \frac{\pi}{2} \leq v \leq \displaystyle \frac{\pi}{2} och att \sin{v} = a. Uttryck med hjälp av a
| \textrm{a) } | \sin{(-v)} | \textrm{b) } | \sin{(\pi-v)} | \textrm{c) } | \cos{v} |
| \textrm{d) } | \sin{\left(\displaystyle \frac{\pi}{2}-v\right)} | \textrm{e) } | \cos{\left( \displaystyle \frac{\pi}{2} + v\right)} | \textrm{f) } | \sin{\left( \displaystyle \frac{\pi}{3} + v \right)} |
Facit till alla delfrågorna
| \textrm{a) } | -a | \textrm{b) } | a | \textrm{c) } | \sqrt{1-a^2} |
| \textrm{d) } | \sqrt{1-a^2} | \textrm{e) } | -a | \textrm{f) } | \displaystyle \frac{\sqrt{3}}{2}\sqrt{1-a^2}+\displaystyle \frac{1}{2}\cdot a |
Lösning till delfråga a
Lösning till delfråga b
Lösning till delfråga c
Lösning till delfråga d
Lösning till delfråga e
Lösning till delfråga f
Övning 4.3:4
Antag att 0 \leq v \leq \pi och att \cos{v}=b. Uttryck med hjälp av b
| \textrm{a) } | \sin^2{v} | \textrm{b) } | \sin{v} | \textrm{c) } | \sin{2v} |
| \textrm{d) } | \cos{2v} | \textrm{e) } | \sin{\left( v+\displaystyle \frac{\pi}{4} \right)} | \textrm{f) } | \cos{\left( v-\displaystyle \frac{\pi}{3} \right)} |
Facit till alla delfrågorna
| \textrm{a) } | 1-b^2 | \textrm{b) } | \sqrt{1-b^2} | \textrm{c) } | 2b\sqrt{1-b^2} |
| \textrm{d) } | 2b^2-1 | \textrm{e) } | \sqrt{1-b^2}\cdot\displaystyle \frac{1}{\sqrt{2}} + b\cdot \displaystyle \frac{1}{\sqrt{2}} | \textrm{f) } | b\cdot\displaystyle \frac{1}{2}+\sqrt{1-b^2}\cdot\displaystyle \frac{\sqrt{3}}{2} |
Lösning till delfråga a
Lösning till delfråga b
Lösning till delfråga c
Lösning till delfråga d
Lösning till delfråga e
Lösning till delfråga f
Övning 4.3:5
För en spetsig vinkel v i en triangel gäller att \sin{v}=\displaystyle \frac{5}{7}. Bestäm \cos{v} och \tan{v}.
| \cos{v}=\displaystyle \frac{2\sqrt{6}}{7} | \tan{v}=\displaystyle \frac{5}{2\sqrt{6}} |
Övning 4.3:6
| \textrm{a) } | Bestäm \sin{v} och \tan{v} om \cos{v}=\displaystyle \frac{3}{4} och \displaystyle \frac{3\pi}{2} \leq v \leq 2\pi. |
| \textrm{b) } | Bestäm \cos{v} och \tan{v} om \sin{v}=\displaystyle \frac{3}{10} och v ligger i den andra kvadranten. |
| \textrm{c) } | Bestäm \sin{v} och \cos{v} om \tan{v}=3 och \pi \leq v \leq \displaystyle \frac{3\pi}{2} |
Facit till alla delfrågorna
| \textrm{a) } | \sin{v}=-\displaystyle \frac{\sqrt{7}}{4} och \tan{v}=-\displaystyle \frac{\sqrt{7}}{3} |
| \textrm{b) } | \cos{v}=-\displaystyle \frac{\sqrt{91}}{10} och \tan{v}=-\displaystyle \frac{3}{\sqrt{91}} |
| \textrm{c) } | \sin{v}=-\displaystyle \frac{3}{\sqrt{10}} och \cos{v}=-\displaystyle \frac{1}{\sqrt{10}} |
Lösning till delfråga a
Bild:4 3 6a.gif
Lösning till delfråga b
Bild:4 3 6b.gif
Lösning till delfråga c
Bild:4 3 6c.gif
