From Förberedande kurs i matematik 1

Revision as of 14:55, 31 March 2008 (edit) Lina (Talk | contribs) (Ny sida: {{NAVCONTENT_START}} <center> Bild:2_2_6e-1(2).gif </center> {{NAVCONTENT_STOP}} {{NAVCONTENT_START}} <center> Bild:2_2_6e-2(2).gif </center> {{NAVCONTENT_STOP}}) ← Previous diff		Current revision (13:30, 24 September 2008) (edit) (undo) Tek (Talk | contribs) m
(4 intermediate revisions not shown.)
Line 1:		Line 1:
-	{{NAVCONTENT_START}}	+	The lines have a point of intersection at that point which simultaneously satisfies the equations of both lines
-	<center> [[Bild:2_2_6e-1(2).gif]] </center>	+
-	{{NAVCONTENT_STOP}}	+	{{Displayed math||<math>2x+y-1=0\qquad\text{and}\qquad y-2x-2=0\,\textrm{.}</math>}}
-	{{NAVCONTENT_START}}	+
-	<center> [[Bild:2_2_6e-2(2).gif]] </center>	+	If we make ''y'' the subject of the second equation <math>y=2x+2</math> and substitute it into the first equation, we obtain an equation which only contains ''x'',
-	{{NAVCONTENT_STOP}}	+
		+	{{Displayed math||<math>2x+(2x+2)-1=0\quad\Leftrightarrow\quad 4x+1=0\,,</math>}}
		+
		+	which gives that <math>x=-1/4\,</math>. Then, from the relation <math>y=2x+2</math>, we obtain <math>y = 2\cdot(-1/4)+2 = 3/2\,</math>.
		+
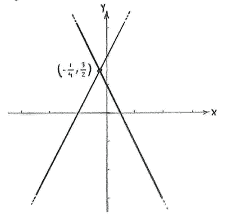
		+	The point of intersection is
		+	<math>\bigl(-\tfrac{1}{4},\tfrac{3}{2}\bigr)</math>.
		+
		+
		+	<center>[[Image:2_2_6_e.gif|center]]</center>
		+
		+
		+	We check for safety's sake that <math>\bigl(-\tfrac{1}{4},\tfrac{3}{2}\bigr)</math>
		+	really satisfies both equations:
		+
		+	:*2''x''&nbsp;+&nbsp;''y''&nbsp;-&nbsp;1&nbsp;=&nbsp;0: <math>\quad\textrm{LHS} = 2\cdot\bigl(-\tfrac{1}{4}\bigr) + \tfrac{3}{2} - 1 = -\tfrac{1}{2} + \tfrac{3}{2} - \tfrac{2}{2} = 0 = \textrm{RHS.}</math>
		+
		+	:*''y''&nbsp;-&nbsp;2''x''&nbsp;-&nbsp;2&nbsp;=&nbsp;0: <math>\quad\textrm{LHS} = \tfrac{3}{2}-2\cdot\bigl(-\tfrac{1}{4}\bigr)-2 = \tfrac{3}{2} + \tfrac{1}{2} - \tfrac{4}{2} = 0 = \textrm{RHS.}</math>

---

Current revision

The lines have a point of intersection at that point which simultaneously satisfies the equations of both lines

\displaystyle 2x+y-1=0\qquad\text{and}\qquad y-2x-2=0\,\textrm{.}

If we make y the subject of the second equation \displaystyle y=2x+2 and substitute it into the first equation, we obtain an equation which only contains x,

\displaystyle 2x+(2x+2)-1=0\quad\Leftrightarrow\quad 4x+1=0\,,

which gives that \displaystyle x=-1/4\,. Then, from the relation \displaystyle y=2x+2, we obtain \displaystyle y = 2\cdot(-1/4)+2 = 3/2\,.

The point of intersection is \displaystyle \bigl(-\tfrac{1}{4},\tfrac{3}{2}\bigr).

We check for safety's sake that \displaystyle \bigl(-\tfrac{1}{4},\tfrac{3}{2}\bigr) really satisfies both equations:

• 2x + y - 1 = 0: \displaystyle \quad\textrm{LHS} = 2\cdot\bigl(-\tfrac{1}{4}\bigr) + \tfrac{3}{2} - 1 = -\tfrac{1}{2} + \tfrac{3}{2} - \tfrac{2}{2} = 0 = \textrm{RHS.}

• y - 2x - 2 = 0: \displaystyle \quad\textrm{LHS} = \tfrac{3}{2}-2\cdot\bigl(-\tfrac{1}{4}\bigr)-2 = \tfrac{3}{2} + \tfrac{1}{2} - \tfrac{4}{2} = 0 = \textrm{RHS.}