Processing Math: Done
To print higher-resolution math symbols, click the
Hi-Res Fonts for Printing button on the jsMath control panel.
jsMath

Solution 4.4:3b

From Förberedande kurs i matematik 1

(Difference between revisions)
Jump to: navigation, search
m (Lösning 4.4:3b moved to Solution 4.4:3b: Robot: moved page)
Line 1: Line 1:
-
{{NAVCONTENT_START}}
+
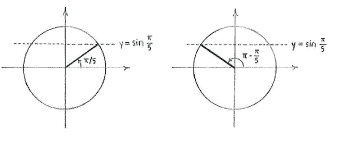
We see directly that
-
<center> [[Image:4_4_3b.gif]] </center>
+
<math>x=\frac{\pi }{5}</math>
-
{{NAVCONTENT_STOP}}
+
is a solution to the equation, and using the unit circle we can also draw the conclusion that
 +
<math>x=\pi -\frac{\pi }{5}=\frac{4\pi }{5}</math>
 +
is the only other solution between
 +
<math>0</math>
 +
and
 +
<math>\text{2}\pi </math>.
 +
 
[[Image:4_4_3_b.gif|center]]
[[Image:4_4_3_b.gif|center]]
 +
 +
We obtain all solutions to the equation when we add integer multiples of
 +
<math>\text{2}\pi </math>,
 +
 +
 +
<math>x=\frac{\pi }{5}+2n\pi </math>
 +
and
 +
<math>x=\frac{4\pi }{5}+2n\pi </math>
 +
 +
 +
where
 +
<math>n</math>
 +
is an arbitrary integer.

Revision as of 09:43, 1 October 2008

We see directly that x=5 is a solution to the equation, and using the unit circle we can also draw the conclusion that x=−5=54 is the only other solution between 0 and 2.


We obtain all solutions to the equation when we add integer multiples of 2,


x=5+2n and x=54+2n


where n is an arbitrary integer.

Retrieved from "http://wiki.math.se/wikis/2008/bridgecourse1-ImperialCollege/index.php/Solution_4.4:3b"