Processing Math: Done

From Förberedande kurs i matematik 1

Revision as of 12:37, 3 April 2008 (edit) Oskar (Talk | contribs) (Ny sida: {{NAVCONTENT_START}} <center> Bild:4_4_5c-1(2).gif </center> {{NAVCONTENT_STOP}} {{NAVCONTENT_START}} <center> Bild:4_4_5c-2(2).gif </center> {{NAVCONTENT_STOP}}) ← Previous diff		Current revision (14:09, 13 October 2008) (edit) (undo) Tek (Talk | contribs) m
(4 intermediate revisions not shown.)
Line 1:		Line 1:
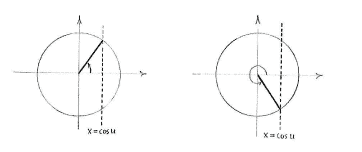
-	{{NAVCONTENT_START}}	+	For a fixed value of ''u'', an equality of the form
-	<center> [[Bild:4_4_5c-1(2).gif]] </center>	+
-	{{NAVCONTENT_STOP}}	+	{{Displayed math||<math>\cos u=\cos v</math>}}
-	{{NAVCONTENT_START}}	+
-	<center> [[Bild:4_4_5c-2(2).gif]] </center>	+	is satisfied by two angles ''v'' in the unit circle,
-	{{NAVCONTENT_STOP}}	+
		+	{{Displayed math||<math>v=u\qquad\text{and}\qquad v=-u\,\textrm{.}</math>}}
		+
		+	[[Image:4_4_5_c.gif|center]]
		+
		+	This means that all angles ''v'' which satisfy the equality are
		+
		+	{{Displayed math||<math>v=u+2n\pi\qquad\text{and}\qquad v=-u+2n\pi\,,</math>}}
		+
		+	where ''n'' is an arbitrary integer.
		+
		+	Therefore, the equation
		+
		+	{{Displayed math||<math>\cos 5x=\cos (x+\pi/5)</math>}}
		+
		+	has the solutions
		+
		+	{{Displayed math||<math>\left\{\begin{align} 5x&=x+\frac{\pi}{5}+2n\pi\quad\text{or}\\[5pt] 5x &= -x-\frac{\pi}{5}+2n\pi\,\textrm{.}\end{align}\right.</math>}}
		+
		+	If we collect ''x'' onto one side, we end up with
		+
		+	{{Displayed math||<math>\left\{\begin{align}
		+	x &= \frac{\pi}{20} + \frac{n\pi}{2}\,,\\[5pt]
		+	x &= -\frac{\pi }{30}+\frac{n\pi}{3}\,,
		+	\end{align}\right.</math>}}
		+
		+	where ''n'' is an arbitrary integer.

---

Current revision

For a fixed value of u, an equality of the form

cosu=cosv

is satisfied by two angles v in the unit circle,

v=uandv=−u.

This means that all angles v which satisfy the equality are

v=u+2nandv=−u+2n

where n is an arbitrary integer.

Therefore, the equation

cos5x=cos(x+5)

has the solutions

5x5x=x+5+2nor=−x−5+2n.

If we collect x onto one side, we end up with

xx=20+2n=−30+3n

where n is an arbitrary integer.