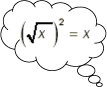3.1 Roots

From Förberedande kurs i matematik 1

(Difference between revisions)
Jump to: navigation, search
(Ny sida: __NOTOC__ {{Info| '''Innehåll:''' *Kvadratrot och ''n'':te rot *Rotlagar }} {{Info| '''Lärandemål:''' Efter detta avsnitt ska du ha lärt dig att: *Skriva om ett rotuttryck i potensfor...)
Current revision (19:32, 26 October 2008) (edit) (undo)
 
(43 intermediate revisions not shown.)
Line 1: Line 1:
__NOTOC__
__NOTOC__
 +
{| border="0" cellspacing="0" cellpadding="0" height="30" width="100%"
 +
| style="border-bottom:1px solid #797979" width="5px" |  
 +
{{Selected tab|[[3.1 Roots|Theory]]}}
 +
{{Not selected tab|[[3.1 Exercises|Exercises]]}}
 +
| style="border-bottom:1px solid #797979" width="100%"|  
 +
|}
 +
{{Info|
{{Info|
-
'''Innehåll:'''
+
'''Contents:'''
-
*Kvadratrot och ''n'':te rot
+
*Square roots and ''n'''th roots
-
*Rotlagar
+
*Manipulating roots
}}
}}
{{Info|
{{Info|
-
'''Lärandemål:'''
+
'''Learning outcomes:'''
-
Efter detta avsnitt ska du ha lärt dig att:
+
After this section, you will have learned:
-
*Skriva om ett rotuttryck i potensform.
+
*How to calculate the square root of some simple integers.
-
*Beräkna kvadratroten ur några enkla heltal.
+
*That the square root of a negative number is not defined.
-
*Kvadratroten ur ett negativt tal inte är definierat.
+
*That the square root of a number denotes the positive root.
-
*Kvadratroten ur ett tal betecknar den positiva roten.
+
*How to manipulate roots in the simplification of expressions.
-
*Hantera rotlagarna i förenkling av rotuttryck.
+
*To recognise when the methods of manipulating roots are valid.
-
*Veta när rotlagarna är giltiga (icke-negativa radikander).
+
*How to simplify expressions containing quadratic roots in the denominator.
-
*Förenkla rotuttryck med kvadratrötter i nämnaren.
+
*When the ''n'''th root of a negative number is defined (''n'' odd).
-
*Veta när ''n'':te roten ur ett negativt tal är definierat (''n'' udda).
+
}}
}}
-
== Kvadratrötter ==
+
== Square roots ==
-
[[Bild:rotbubbla.gif|right]]
+
[[Image:rotbubbla.gif|right]]
-
Symbolen <math>\sqrt{a}</math>, kvadratroten ur <math>a</math>, används som bekant för att beteckna det tal som multiplicerat med sig självt blir <math>a</math>. Man måste dock vara lite mer exakt när man definierar denna symbol.
+
The well-known symbol <math>\sqrt{a}</math>, the square root of <math>a</math>, is used to describe the number that when multiplied by itself gives <math>a</math>. However, one has to be a little more precise in defining this symbol.
-
Ekvationen <math>x^2 = 4</math> har två lösningar <math>x = 2</math> och <math>x = -2</math>, eftersom såväl <math>2\cdot 2 = 4</math> som <math>(-2)\cdot(-2) = 4</math>. Man skulle då kunna tro att <math>\sqrt{4}</math> kan vara vilket som helst av <math>-2</math> och <math>2</math>, dvs. <math>\sqrt{4}= \pm 2</math>, men <math>\sqrt{4}</math> betecknar '''bara''' det positiva talet <math>2</math>.
+
The equation <math>x^2 = 4</math> has two solutions <math>x = 2</math> and <math>x = -2</math>, since both <math>2\cdot 2 = 4</math> and <math>(-2)\cdot(-2) = 4</math>. It would then be logical to suppose that <math>\sqrt{4}</math> can be either <math>-2</math> or <math>2</math>, i.e. <math>\sqrt{4}= \pm 2</math>, but <math>\sqrt{4}</math> only denotes the positive number <math>2</math>.
<div class="regel">
<div class="regel">
-
Kvadratroten <math>\sqrt{a}</math> betecknar det '''icke-negativa tal''' som multiplicerat med sig självt blir <math>a,</math> dvs. den icke-negativa lösningen till ekvationen <math>x^2 = a</math>.
+
The square root <math>\sqrt{a}</math> means '''the non-negative number''' that multiplied by
 +
itself gives <math>a,</math> i.e. the non-negative solution to the equation <math>x^2 = a</math>.
-
Kvadratroten ur <math>a</math> kan även skrivas <math>a^{1/2}</math>.
+
The square root of <math>a</math> can also be written as <math>a^{1/2}</math>.
</div>
</div>
-
Det är därför fel att påstå att <math>\sqrt{4}= \pm 2,</math> men korrekt att säga att ekvationen <math>x^2 = 4</math> har lösningarna <math>x = \pm 2</math>.
+
It is therefore wrong to state that <math>\sqrt{4}= \pm 2,</math> but correct to state that the equation <math>x^2 = 4</math> has the solution <math>x = \pm 2</math>.
<div class="exempel">
<div class="exempel">
-
'''Exempel 1'''
+
''' Example 1'''
<ol type="a">
<ol type="a">
-
<li><math>\sqrt{0}=0 \quad</math> eftersom <math>0^2 = 0 \cdot 0
+
<li><math>\sqrt{0}=0 \quad</math> because <math>0^2 = 0 \cdot 0
-
= 0</math> och <math>0</math> är inte negativ.</li>
+
= 0</math> and <math>0</math> is not negative. </li>
-
<li><math>\sqrt{100}=10 \quad</math> eftersom <math> 10^2 = 10 \cdot 10
+
<li><math>\sqrt{100}=10 \quad</math> since <math> 10^2 = 10 \cdot 10
-
= 100 </math> och <math>10</math> är ett positivt tal.</li>
+
= 100 </math> and <math>10</math> is a positive number. </li>
-
<li> <math>\sqrt{0{,}25}=0{,}5 \quad</math> eftersom <math>0{,}5^2
+
<li> <math>\sqrt{0_.25}=0_.5 \quad</math> since <math>0_.5^2
-
= 0{,}5 \cdot 0{,}5 = 0{,}25 </math> och <math>0{,}5</math> är positiv.</li>
+
= 0_.5 \cdot 0_.5 = 0_.25 </math> and <math>0{_.}5</math> is positive. </li>
-
<li><math>\sqrt{2} \approx 1{,}4142 \quad</math> eftersom <math>1{,}4142
+
<li><math>\sqrt{2} \approx 1{_.}4142 \quad</math> since <math>1{_.}4142
-
\cdot 1{,}4142 \approx 2</math> och <math>1{,}4142</math> är positiv.</li>
+
\cdot 1{_.}4142 \approx 2</math> and <math>1{_.}4142</math> is positive. </li>
-
<li>Ekvationen <math>x^2=2</math> har lösningarna <math>x=\sqrt{2}
+
<li> The equation <math>x^2=2</math> has the solutions <math>x=\sqrt{2}
-
\approx 1{,}414</math> och <math>x = -\sqrt{2} \approx -1{,}414</math>.</li>
+
\approx 1{_.}414</math> and <math>x = -\sqrt{2} \approx -1{_.}414</math>.</li>
-
<li><math>\sqrt{-4}\quad</math> är inte definierat, eftersom det inte finns något reellt tal <math>x</math> som uppfyller <math>x^2=-4</math>.</li>
+
<li><math>\sqrt{-4}\quad</math> is not defined, since there is no real number <math>x</math> that satisfies <math>x^2=-4</math>.</li>
-
<li><math>\sqrt{(-7)^2} = 7 \quad</math> eftersom <math> \sqrt{(-7)^2}
+
<li><math>\sqrt{(-7)^2} = 7 \quad</math> because <math> \sqrt{(-7)^2}
= \sqrt{(-7) \cdot (-7)} = \sqrt{49} = \sqrt{ 7 \cdot 7} = 7</math>.</li>
= \sqrt{(-7) \cdot (-7)} = \sqrt{49} = \sqrt{ 7 \cdot 7} = 7</math>.</li>
</ol>
</ol>
</div>
</div>
-
När man räknar med kvadratrötter kan det vara bra att känna till några räkneregler. Eftersom <math>\sqrt{a} = a^{1/2}</math> kan vi överföra potenslagarna till "rotlagar". Vi har t.ex. att
+
It is useful to know how square roots behave for calculations. As <math>\sqrt{a} = a^{1/2}</math> we can handle expressions involving roots as we would expressions involving exponents. For example, we have
-
{{Fristående formel||<math>\sqrt{9\cdot 4}
+
{{Displayed math||<math>\sqrt{9\cdot 4}
= (9\cdot 4)^{1/2}
= (9\cdot 4)^{1/2}
= 9^{1/2}\cdot 4^{1/2}
= 9^{1/2}\cdot 4^{1/2}
= \sqrt{9}\cdot \sqrt{4}\mbox{.}</math>}}
= \sqrt{9}\cdot \sqrt{4}\mbox{.}</math>}}
-
På detta sätt kan vi få fram följande räkneregler för kvadratrötter,
+
In this way we obtain the following rules for quadratic roots.
-
som gäller för alla reella tal <math> a, b \ge 0:</math>
+
<div class="regel">
<div class="regel">
-
{{Fristående formel||<math>\begin{align*}
+
For all real numbers <math> a, b \ge 0:</math>
 +
{{Displayed math||<math> \begin{align*}
\sqrt{ab} &= \sqrt{\vphantom{b}a}\cdot \sqrt{b}\\[4pt]
\sqrt{ab} &= \sqrt{\vphantom{b}a}\cdot \sqrt{b}\\[4pt]
-
\sqrt{\frac{a}{b}} &= \frac{\sqrt{a}}{\sqrt{b}}\\[4pt]
+
\sqrt{\frac{a}{b}} &= \frac{\sqrt{a}}{\sqrt{b}}, \quad\ \mathrm{for}\ b\neq0\\[4pt]
a\sqrt{b} &= \sqrt{a^2b}
a\sqrt{b} &= \sqrt{a^2b}
\end{align*}</math>}}
\end{align*}</math>}}
</div>
</div>
-
(Vi måste dock vid divisionen ovan som vanligt förutsätta att ''b'' inte är 0.)
 
<div class="exempel">
<div class="exempel">
-
'''Exempel 2'''
+
''' Example 2'''
<ol type="a">
<ol type="a">
Line 90: Line 96:
</div>
</div>
-
Observera att räknereglerna ovan förutsätter att <math>a</math> och <math>b \ge 0</math>. Om <math>a</math> och <math>b</math> är negativa (<&nbsp;0) så är inte <math>\sqrt{a}</math> och <math>\sqrt{b}</math> definierade som reella tal. Man skulle t.ex. kunna frestas att skriva
+
Note that the above calculations assume that <math>a</math> and <math>b \ge 0</math>. If <math>a</math> and <math>b</math> are negative (<&nbsp;0) then <math>\sqrt{a}</math> and <math>\sqrt{b}</math> are not defined as real numbers. It is tempting to write , for example,
-
{{Fristående formel||<math>-1 = \sqrt{-1} \cdot \sqrt{-1} = \sqrt{ (-1) \cdot (-1) } = \sqrt{1} = 1</math>}}
+
{{Displayed math||<math>-1 = \sqrt{-1} \cdot \sqrt{-1} = \sqrt{ (-1) \cdot (-1) } = \sqrt{1} = 1</math>}}
-
men ser då att något inte stämmer. Anledningen är att <math> \sqrt{-1} </math> inte är ett reellt tal, vilket alltså gör att räknereglerna ovan inte får användas.
+
but something here cannot be right. The explanation is that <math> \sqrt{-1} </math> is not a real number, which means the laws of roots discussed above may not be used.
-
== Högre ordningars rötter ==
+
== Higher order roots ==
-
Kubikroten ur ett tal <math>a</math> definieras som det tal som multiplicerat med sig själv tre gånger ger <math>a</math>, och betecknas <math>\sqrt[\scriptstyle 3]{a}</math>.
+
The cube root of a number <math>a</math> is defined as the number that multiplied by itself three times gives <math>a</math>, and is denoted as <math>\sqrt[\scriptstyle 3]{a}</math>.
<div class="exempel">
<div class="exempel">
-
'''Exempel 3'''
+
''' Example 3'''
<ol type="a">
<ol type="a">
-
<li><math>\sqrt[\scriptstyle 3]{8} = 2 \quad</math> eftersom <math>2
+
<li><math>\sqrt[\scriptstyle 3]{8} = 2 \quad</math> as <math>2
\cdot 2 \cdot 2=8</math>.</li>
\cdot 2 \cdot 2=8</math>.</li>
-
<li><math>\sqrt[\scriptstyle 3]{0{,}027} = 0{,}3
+
<li><math>\sqrt[\scriptstyle 3]{0{_.}027} = 0{_.}3
-
\quad</math> eftersom <math>0{,}3 \cdot 0{,}3 \cdot 0{,}3
+
\quad</math> since <math>0{_.}3 \cdot 0{_.}3 \cdot 0{_.}3
-
= 0{,}027</math>.</li>
+
= 0{_.}027</math>.</li>
-
<li><math>\sqrt[\scriptstyle 3]{-8} = -2 \quad</math> eftersom <math>(-2)
+
<li><math>\sqrt[\scriptstyle 3]{-8} = -2 \quad</math> because <math>(-2)
\cdot (-2) \cdot (-2)= -8</math>.
\cdot (-2) \cdot (-2)= -8</math>.
</ol>
</ol>
</div>
</div>
-
Notera att, till skillnad från kvadratrötter, är kubikrötter även definierade för negativa tal.
+
Note that, unlike square roots, cube roots are also defined for negative numbers.
-
Det går sedan att för postiva heltal <math>n</math> definiera n:te roten ur ett tal <math>a</math> som
+
For any positive integer <math>n</math> one can define the <math>n</math>'th root of a number <math>a</math>:
-
* om <math>n</math> är jämn och <math>a\ge0</math> är <math>\sqrt[\scriptstyle n]{a}</math> det icke-negativa tal som multiplicerat med sig själv <math>n</math> gånger blir <math>a</math>,
+
* if <math>n</math> is even and <math>a\ge0</math> then <math>\sqrt[\scriptstyle n]{a}</math> is the non-negative number that when multiplied by itself <math>n</math> times gives <math>a</math>,
-
* om <math>n</math> är udda så är <math>\sqrt[\scriptstyle n]{a}</math> det tal som multiplicerat med sig självt <math>n</math> gånger blir <math>a</math>.
+
* if <math>n</math> is odd, <math>\sqrt[\scriptstyle n]{a}</math> is the number that when multiplied by itself <math>n</math> times gives <math>a</math>.
-
Roten <math>\sqrt[\scriptstyle n]{a}</math> kan även skrivas som <math>a^{1/n}</math>.
+
The root <math>\sqrt[\scriptstyle n]{a}</math> can also be written as <math>a^{1/n}</math>.
<div class="exempel">
<div class="exempel">
-
'''Exempel 4'''
+
''' Example 4'''
<ol type="a">
<ol type="a">
-
<li><math>\sqrt[\scriptstyle 4]{625} = 5\quad</math> eftersom <math>5
+
<li><math>\sqrt[\scriptstyle 4]{625} = 5\quad</math> since <math>5
\cdot 5 \cdot 5 \cdot 5 = 625</math>.</li>
\cdot 5 \cdot 5 \cdot 5 = 625</math>.</li>
-
<li><math>\sqrt[\scriptstyle 5]{-243} = -3\quad</math> eftersom <math>(-3)
+
<li><math>\sqrt[\scriptstyle 5]{-243} = -3\quad</math> because <math>(-3)
\cdot (-3) \cdot (-3) \cdot (-3) \cdot (-3) = -243</math>.</li>
\cdot (-3) \cdot (-3) \cdot (-3) \cdot (-3) = -243</math>.</li>
-
<li><math>\sqrt[\scriptstyle 6]{-17}\quad</math> är inte definierat eftersom <math>6</math> är jämn och <math>-17</math> är ett negativt tal.</li>
+
<li><math>\sqrt[\scriptstyle 6]{-17}\quad</math> is not defined as <math>6</math> is even and <math>-17</math> is a negative number. </li>
</ol>
</ol>
</div>
</div>
-
För <math>n</math>:te rötter gäller samma räkneregler som för kvadratrötter om <math>a, \, b \ge 0</math>. Observera att om <math>n</math> är udda gäller de även för negativa <math>a</math> och <math>b</math>, dvs. för alla reella tal <math>a</math> och <math>b</math>.
+
For <math>n</math>'th roots the same rules apply as for quadratic roots if <math>a, \, b \ge 0</math>. Note that if <math>n</math> is odd these methods apply even for negative <math>a</math> and <math>b</math>, that is, for all real numbers <math>a</math> and <math>b</math>.
<div class="regel">
<div class="regel">
-
{{Fristående formel||<math>\begin{align*}
+
{{Displayed math||<math>\begin{align*}
\sqrt[\scriptstyle n]{ab}
\sqrt[\scriptstyle n]{ab}
&= \sqrt[\scriptstyle n]{\vphantom{b}a}\cdot
&= \sqrt[\scriptstyle n]{\vphantom{b}a}\cdot
\sqrt[\scriptstyle n]{b}\\[4pt]
\sqrt[\scriptstyle n]{b}\\[4pt]
\sqrt[\scriptstyle n]{\frac{a}{b}}
\sqrt[\scriptstyle n]{\frac{a}{b}}
-
&= \frac{\sqrt[\scriptstyle n]{a}}{\sqrt[\scriptstyle n]{b}}\\[4pt]
+
&= \frac{\sqrt[\scriptstyle n]{a}}{\sqrt[\scriptstyle n]{b}}, \quad\ \mathrm{for}\ b\neq0\\[4pt]
a\,\sqrt[\scriptstyle n]{b}
a\,\sqrt[\scriptstyle n]{b}
&= \sqrt[\scriptstyle n]{a^nb}
&= \sqrt[\scriptstyle n]{a^nb}
Line 151: Line 157:
-
== Förenkling av rotuttryck ==
+
== Simplification of expressions containing roots ==
-
Ofta kan man genom att använda räknereglerna för rötter förenkla rotuttryck väsentligt. Liksom vid potensräkning handlar det ofta om att bryta ner uttryck i så "små" rötter som möjligt. Exempelvis gör man gärna omskrivningen
+
Often one can significantly simplify expressions containing roots by using the rules described above. As is also the case when dealing with exponents, it is desirable to reduce expressions into "small" roots. For example, it is a good idea to do the following
-
{{Fristående formel||<math>\sqrt{8}
+
{{Displayed math||<math>\sqrt{8}
= \sqrt{4\cdot2}
= \sqrt{4\cdot2}
= \sqrt{4} \cdot \sqrt{2}
= \sqrt{4} \cdot \sqrt{2}
= 2\sqrt{2}</math>}}
= 2\sqrt{2}</math>}}
-
eftersom man då kan förenkla t.ex.
+
because it helps simplification as we see here
-
{{Fristående formel||<math>\frac{\sqrt{8}}{2}
+
{{Displayed math||<math>\frac{\sqrt{8}}{2}
= \frac{2 \sqrt{2}}{2}
= \frac{2 \sqrt{2}}{2}
= \sqrt{2}\mbox{.}</math>}}
= \sqrt{2}\mbox{.}</math>}}
-
Genom att skriva rotuttryck i termer av "små" rötter kan man också addera rötter av "samma sort", t.ex.
+
By rewriting expressions containing roots in terms of "small" roots one can also sum roots of "the same kind", e.g.
-
{{Fristående formel||<math>\sqrt{8} + \sqrt{2}
+
{{Displayed math||<math>\sqrt{8} + \sqrt{2}
= 2\sqrt{2} + \sqrt{2}
= 2\sqrt{2} + \sqrt{2}
= (2+1)\sqrt{2}
= (2+1)\sqrt{2}
Line 174: Line 180:
<div class="exempel">
<div class="exempel">
-
'''Exempel 5'''
+
''' Example 5'''
<ol type="a">
<ol type="a">
Line 228: Line 234:
<li><math>(\sqrt{3} + \sqrt{2}\,)(\sqrt{3} - \sqrt{2}\,)
<li><math>(\sqrt{3} + \sqrt{2}\,)(\sqrt{3} - \sqrt{2}\,)
= (\sqrt{3}\,)^2-(\sqrt{2}\,)^2 = 3-2 = 1</math>
= (\sqrt{3}\,)^2-(\sqrt{2}\,)^2 = 3-2 = 1</math>
-
:där vi använt konjugatregeln <math>(a+b)(a-b) = a^2 - b^2</math> med <math>a=\sqrt{3}</math> och <math>b=\sqrt{2}</math>.</li>
+
where we have used the difference of two squares <math>(a+b)(a-b) = a^2 - b^2</math> with <math>a=\sqrt{3}</math> and <math>b=\sqrt{2}</math>.</li>
</ol>
</ol>
</div>
</div>
-
== Rationella rotuttryck ==
+
== Rational root expressions ==
-
När rötter förekommer i ett rationellt uttryck vill man ofta undvika rötter i nämnaren (eftersom det är svårt vid handräkning att dividera med irrationella tal). Genom att förlänga med <math> \sqrt{2} </math> kan man exempelvis göra omskrivningen
+
When roots appear in a rational expression it is often useful to write the expression in a form which does not contain roots in the denominator. This is because it is difficult to divide by irrational numbers in calculations by hand. In the example below, multiplying by <math> 1=\frac{\sqrt{2}}{\sqrt{2}} </math>, one obtains
-
{{Fristående formel||<math>\frac{1}{\sqrt{2}}
+
{{Displayed math||<math>\frac{1}{\sqrt{2}}
= \frac{1\cdot\sqrt{2}}{\sqrt{2}\cdot\sqrt{2}}
= \frac{1\cdot\sqrt{2}}{\sqrt{2}\cdot\sqrt{2}}
= \frac{\sqrt{2}}{2}</math>}}
= \frac{\sqrt{2}}{2}</math>}}
-
vilket oftast är att föredra.
+
which usually is preferable.
-
I andra fall kan man utnyttja konjugatregeln, <math>(a+b)(a-b) = a^2 - b^2</math>, och förlänga med nämnarens s.k. ''konjugerade uttryck''. På så sätt försvinner rottecknen från nämnaren genom kvadreringen, t.ex.
+
In other cases, we can use the difference of two squares i.e <math>(a+b)(a-b) = a^2 – b^2</math> to eliminate the root from the denominator. Indeed, if we multiply both the numerator and denominator by the denominator´s “conjugate” expression, then the root in the denominator gets squared and hence is removed. For example,
-
{{Fristående formel||<math>\begin{align*}
+
{{Displayed math||<math>\begin{align*}
\frac{\sqrt{3}}{\sqrt{2}+1}
\frac{\sqrt{3}}{\sqrt{2}+1}
&= \frac{\sqrt{3}}{\sqrt{2}+1} \cdot \frac{\sqrt{2}-1}{\sqrt{2}-1}
&= \frac{\sqrt{3}}{\sqrt{2}+1} \cdot \frac{\sqrt{2}-1}{\sqrt{2}-1}
Line 256: Line 262:
<div class="exempel">
<div class="exempel">
-
'''Exempel 6'''
+
''' Example 6'''
<ol type="a">
<ol type="a">
Line 285: Line 291:
-
[[3.1 Övningar|Övningar]]
+
[[3.1 Exercises|Exercises]]
-
 
+
-
 
+
-
<div class="inforuta">
+
-
'''Råd för inläsning'''
+
-
'''Grund- och slutprov'''
 
-
Efter att du har läst texten och arbetat med övningarna ska du göra grund- och slutprovet för att bli godkänd på detta avsnitt. Du hittar länken till proven i din student lounge.
+
<div class="inforuta" style="width:580px;">
 +
'''Study advice'''
 +
'''Basic and final tests'''
-
'''Tänk på att:'''
+
After you have read the text and worked through the exercises, you should do the basic and final tests to pass this section. You can find the link to the tests in your student lounge.
-
Kvadratroten ur ett tal är alltid icke-negativ (dvs. positiv eller lika med noll)!
+
'''Keep in mind that... '''
-
Rotlagarna är egentligen specialfall av potenslagarna.
+
The square root of a number is always non-negative (that is, positive or zero)!
-
Exempelvis: <math>\sqrt{x}=x^{1/2}</math>
+
Rules for roots are actually a special case of laws of exponents, since, for example, <math>\sqrt{x} = x^{1/2}</math>.
-
'''Lästips'''
+
'''Reviews'''
-
För dig som vill fördjupa dig ytterligare eller behöver en längre förklaring
+
For those of you who want to deepen your understanding or need more detailed explanations consider the following references
-
[http://en.wikipedia.org/wiki/Root_(mathematics) Läs mer om kvadratrötter i engelska Wikipedia]
+
[http://en.wikipedia.org/wiki/Root_(mathematics) Learn more about square roots from Wikipedia ]
-
[http://www.mathacademy.com/pr/prime/articles/irr2/ Hur vet man att roten ur 2 inte är ett bråktal?]
+
[http://www.mathacademy.com/pr/prime/articles/irr2/ How do we know that the square root of 2 is not a fraction?]
-
'''Länktips'''
+
'''Useful web sites'''
-
[http://mathforum.org/dr.math/faq/faq.sqrt.by.hand.html Hur man finner roten ur ett tal, utan hjälp av miniräknare?]
+
[http://mathforum.org/dr.math/faq/faq.sqrt.by.hand.html How to find the root of a number, without the help of calculators?]
</div>
</div>

Current revision

       Theory          Exercises      

Contents:

  • Square roots and n'th roots
  • Manipulating roots

Learning outcomes:

After this section, you will have learned:

  • How to calculate the square root of some simple integers.
  • That the square root of a negative number is not defined.
  • That the square root of a number denotes the positive root.
  • How to manipulate roots in the simplification of expressions.
  • To recognise when the methods of manipulating roots are valid.
  • How to simplify expressions containing quadratic roots in the denominator.
  • When the n'th root of a negative number is defined (n odd).

Square roots

The well-known symbol \displaystyle \sqrt{a}, the square root of \displaystyle a, is used to describe the number that when multiplied by itself gives \displaystyle a. However, one has to be a little more precise in defining this symbol.

The equation \displaystyle x^2 = 4 has two solutions \displaystyle x = 2 and \displaystyle x = -2, since both \displaystyle 2\cdot 2 = 4 and \displaystyle (-2)\cdot(-2) = 4. It would then be logical to suppose that \displaystyle \sqrt{4} can be either \displaystyle -2 or \displaystyle 2, i.e. \displaystyle \sqrt{4}= \pm 2, but \displaystyle \sqrt{4} only denotes the positive number \displaystyle 2.


The square root \displaystyle \sqrt{a} means the non-negative number that multiplied by itself gives \displaystyle a, i.e. the non-negative solution to the equation \displaystyle x^2 = a.

The square root of \displaystyle a can also be written as \displaystyle a^{1/2}.

It is therefore wrong to state that \displaystyle \sqrt{4}= \pm 2, but correct to state that the equation \displaystyle x^2 = 4 has the solution \displaystyle x = \pm 2.

Example 1

  1. \displaystyle \sqrt{0}=0 \quad because \displaystyle 0^2 = 0 \cdot 0 = 0 and \displaystyle 0 is not negative.
  2. \displaystyle \sqrt{100}=10 \quad since \displaystyle 10^2 = 10 \cdot 10 = 100 and \displaystyle 10 is a positive number.
  3. \displaystyle \sqrt{0_.25}=0_.5 \quad since \displaystyle 0_.5^2 = 0_.5 \cdot 0_.5 = 0_.25 and \displaystyle 0{_.}5 is positive.
  4. \displaystyle \sqrt{2} \approx 1{_.}4142 \quad since \displaystyle 1{_.}4142 \cdot 1{_.}4142 \approx 2 and \displaystyle 1{_.}4142 is positive.
  5. The equation \displaystyle x^2=2 has the solutions \displaystyle x=\sqrt{2} \approx 1{_.}414 and \displaystyle x = -\sqrt{2} \approx -1{_.}414.
  6. \displaystyle \sqrt{-4}\quad is not defined, since there is no real number \displaystyle x that satisfies \displaystyle x^2=-4.
  7. \displaystyle \sqrt{(-7)^2} = 7 \quad because \displaystyle \sqrt{(-7)^2} = \sqrt{(-7) \cdot (-7)} = \sqrt{49} = \sqrt{ 7 \cdot 7} = 7.

It is useful to know how square roots behave for calculations. As \displaystyle \sqrt{a} = a^{1/2} we can handle expressions involving roots as we would expressions involving exponents. For example, we have

\displaystyle \sqrt{9\cdot 4} 
 = (9\cdot 4)^{1/2}
 = 9^{1/2}\cdot 4^{1/2}
 = \sqrt{9}\cdot \sqrt{4}\mbox{.}

In this way we obtain the following rules for quadratic roots.

For all real numbers \displaystyle a, b \ge 0:

\displaystyle \begin{align*} 
   \sqrt{ab} &= \sqrt{\vphantom{b}a}\cdot \sqrt{b}\\[4pt]
   \sqrt{\frac{a}{b}} &= \frac{\sqrt{a}}{\sqrt{b}}, \quad\ \mathrm{for}\ b\neq0\\[4pt]
   a\sqrt{b} &= \sqrt{a^2b}
 \end{align*}

Example 2

  1. \displaystyle \sqrt{64\cdot 81} = \sqrt{64}\cdot \sqrt{81} = 8\cdot 9 = 72
  2. \displaystyle \sqrt{\frac{9}{25}} = \frac{\sqrt{9}}{\sqrt{25}} = \frac{3}{5}
  3. \displaystyle \sqrt{18} \cdot \sqrt{2} = \sqrt{18 \cdot 2} = \sqrt{36} = 6
  4. \displaystyle \frac{\sqrt{75}}{\sqrt{3}} = \sqrt{\frac{75}{3}} = \sqrt{25} = 5
  5. \displaystyle \sqrt{12} = \sqrt{ 4 \cdot 3 } = \sqrt{4} \cdot \sqrt{3} = 2\sqrt{3}

Note that the above calculations assume that \displaystyle a and \displaystyle b \ge 0. If \displaystyle a and \displaystyle b are negative (< 0) then \displaystyle \sqrt{a} and \displaystyle \sqrt{b} are not defined as real numbers. It is tempting to write , for example,

\displaystyle -1 = \sqrt{-1} \cdot \sqrt{-1} = \sqrt{ (-1) \cdot (-1) } = \sqrt{1} = 1

but something here cannot be right. The explanation is that \displaystyle \sqrt{-1} is not a real number, which means the laws of roots discussed above may not be used.


Higher order roots

The cube root of a number \displaystyle a is defined as the number that multiplied by itself three times gives \displaystyle a, and is denoted as \displaystyle \sqrt[\scriptstyle 3]{a}.

Example 3

  1. \displaystyle \sqrt[\scriptstyle 3]{8} = 2 \quad as \displaystyle 2 \cdot 2 \cdot 2=8.
  2. \displaystyle \sqrt[\scriptstyle 3]{0{_.}027} = 0{_.}3 \quad since \displaystyle 0{_.}3 \cdot 0{_.}3 \cdot 0{_.}3 = 0{_.}027.
  3. \displaystyle \sqrt[\scriptstyle 3]{-8} = -2 \quad because \displaystyle (-2) \cdot (-2) \cdot (-2)= -8.

Note that, unlike square roots, cube roots are also defined for negative numbers.

For any positive integer \displaystyle n one can define the \displaystyle n'th root of a number \displaystyle a:

  • if \displaystyle n is even and \displaystyle a\ge0 then \displaystyle \sqrt[\scriptstyle n]{a} is the non-negative number that when multiplied by itself \displaystyle n times gives \displaystyle a,
  • if \displaystyle n is odd, \displaystyle \sqrt[\scriptstyle n]{a} is the number that when multiplied by itself \displaystyle n times gives \displaystyle a.

The root \displaystyle \sqrt[\scriptstyle n]{a} can also be written as \displaystyle a^{1/n}.

Example 4

  1. \displaystyle \sqrt[\scriptstyle 4]{625} = 5\quad since \displaystyle 5 \cdot 5 \cdot 5 \cdot 5 = 625.
  2. \displaystyle \sqrt[\scriptstyle 5]{-243} = -3\quad because \displaystyle (-3) \cdot (-3) \cdot (-3) \cdot (-3) \cdot (-3) = -243.
  3. \displaystyle \sqrt[\scriptstyle 6]{-17}\quad is not defined as \displaystyle 6 is even and \displaystyle -17 is a negative number.

For \displaystyle n'th roots the same rules apply as for quadratic roots if \displaystyle a, \, b \ge 0. Note that if \displaystyle n is odd these methods apply even for negative \displaystyle a and \displaystyle b, that is, for all real numbers \displaystyle a and \displaystyle b.

\displaystyle \begin{align*} 
   \sqrt[\scriptstyle n]{ab}
     &= \sqrt[\scriptstyle n]{\vphantom{b}a}\cdot
          \sqrt[\scriptstyle n]{b}\\[4pt]
   \sqrt[\scriptstyle n]{\frac{a}{b}}
     &= \frac{\sqrt[\scriptstyle n]{a}}{\sqrt[\scriptstyle n]{b}}, \quad\ \mathrm{for}\ b\neq0\\[4pt]
   a\,\sqrt[\scriptstyle n]{b}
     &= \sqrt[\scriptstyle n]{a^nb}
 \end{align*}


Simplification of expressions containing roots

Often one can significantly simplify expressions containing roots by using the rules described above. As is also the case when dealing with exponents, it is desirable to reduce expressions into "small" roots. For example, it is a good idea to do the following

\displaystyle \sqrt{8} 
 = \sqrt{4\cdot2}
 = \sqrt{4} \cdot \sqrt{2}
 = 2\sqrt{2}

because it helps simplification as we see here

\displaystyle \frac{\sqrt{8}}{2} 
 = \frac{2 \sqrt{2}}{2}
 = \sqrt{2}\mbox{.}

By rewriting expressions containing roots in terms of "small" roots one can also sum roots of "the same kind", e.g.

\displaystyle \sqrt{8} + \sqrt{2} 
 = 2\sqrt{2} + \sqrt{2}
 = (2+1)\sqrt{2}
 = 3\sqrt{2}\mbox{.}

Example 5

  1. \displaystyle \frac{\sqrt{8}}{\sqrt{18}} = \frac{\sqrt{2 \cdot 4}}{\sqrt{2 \cdot 9}} = \frac{\sqrt{2 \cdot 2 \cdot 2}}{\sqrt{2 \cdot 3 \cdot 3}} = \frac{\sqrt{2 \cdot 2^2}}{\sqrt{2 \cdot 3^2}} = \frac{2\sqrt{2}}{3\sqrt{2}} = \frac{2}{3}
  2. \displaystyle \frac{\sqrt{72}}{6} = \frac{\sqrt{8 \cdot 9}}{ 2 \cdot 3} = \frac{\sqrt{2 \cdot 2 \cdot 2 \cdot 3 \cdot 3}}{ 2 \cdot 3} = \frac{\sqrt{2^2 \cdot 3^2 \cdot 2}}{ 2 \cdot 3} = \frac{2 \cdot 3\sqrt{2}}{2 \cdot 3} = \sqrt{2}
  3. \displaystyle \sqrt{45} + \sqrt{20} = \sqrt{9\cdot5} + \sqrt{4\cdot5} = \sqrt{3^2\cdot5} + \sqrt{2^2\cdot5} = 3\sqrt{5} + 2\sqrt{5}\vphantom{\bigl(}
    \displaystyle \phantom{\sqrt{45} + \sqrt{20}\vphantom{\bigl(}}{} = (3+2)\sqrt{5} = 5\sqrt{5}
  4. \displaystyle \sqrt{50} + 2\sqrt{3} -\sqrt{32} + \sqrt{27}\vphantom{\Bigl(} = \sqrt{5 \cdot 10} + 2\sqrt{3} -\sqrt{2 \cdot 16} + \sqrt{3 \cdot 9}
    \displaystyle \phantom{\sqrt{50} + 2\sqrt{3} -\sqrt{32} + \sqrt{27}\vphantom{\Bigl(}}{} = \sqrt{5 \cdot 2 \cdot 5} + 2\sqrt{3} -\sqrt{2 \cdot 4 \cdot 4} + \sqrt{3 \cdot 3 \cdot 3}\vphantom{a^{b^c}}
    \displaystyle \phantom{\sqrt{50} + 2\sqrt{3} -\sqrt{32} + \sqrt{27}\vphantom{\Bigl(}}{} = \sqrt{5^2 \cdot 2 } + 2\sqrt{3} -\sqrt{2^2 \cdot 2^2 \cdot 2} + \sqrt{3 \cdot 3^2}\vphantom{a^{\textstyle b^{\textstyle c}}}
    \displaystyle \phantom{\sqrt{50} + 2\sqrt{3} -\sqrt{32} + \sqrt{27}\vphantom{\Bigl(}}{} = 5\sqrt{2} +2\sqrt{3} - 2 \cdot 2\sqrt{2} + 3\sqrt{3}\vphantom{a^{\textstyle b^{\textstyle c}}}
    \displaystyle \phantom{\sqrt{50} + 2\sqrt{3} -\sqrt{32} + \sqrt{27}\vphantom{\Bigl(}}{} = (5-4)\sqrt{2} + (2+3)\sqrt{3}\vphantom{a^{\textstyle b^{\textstyle c}}}
    \displaystyle \phantom{\sqrt{50} + 2\sqrt{3} -\sqrt{32} + \sqrt{27}\vphantom{\Bigl(}}{} = \sqrt{2} + 5\sqrt{3}\vphantom{a^{\textstyle b^{\textstyle c}}}
  5. \displaystyle \frac{ 2\cdot\sqrt[\scriptstyle3]{3} }{ \sqrt[\scriptstyle3]{12} } = \frac{ 2\cdot\sqrt[\scriptstyle3]{3} }{ \sqrt[\scriptstyle3]{3 \cdot 4} } = \frac{ 2\cdot\sqrt[\scriptstyle3]{3} }{ \sqrt[\scriptstyle3]{3} \cdot \sqrt[\scriptstyle3]{4} } = \frac{ 2 }{ \sqrt[\scriptstyle3]{4} } = \frac{ 2 }{ \sqrt[\scriptstyle3]{2 \cdot 2} } = \frac{ 2 }{ \sqrt[\scriptstyle3]{2} \cdot \sqrt[\scriptstyle3]{2} } \cdot \displaystyle \frac{\sqrt[\scriptstyle3]{2}}{ \sqrt[\scriptstyle3]{2}} = \frac{ 2\cdot\sqrt[\scriptstyle3]{2} }{ 2 } = \sqrt[\scriptstyle3]{2}
  6. \displaystyle (\sqrt{3} + \sqrt{2}\,)(\sqrt{3} - \sqrt{2}\,) = (\sqrt{3}\,)^2-(\sqrt{2}\,)^2 = 3-2 = 1 where we have used the difference of two squares \displaystyle (a+b)(a-b) = a^2 - b^2 with \displaystyle a=\sqrt{3} and \displaystyle b=\sqrt{2}.


Rational root expressions

When roots appear in a rational expression it is often useful to write the expression in a form which does not contain roots in the denominator. This is because it is difficult to divide by irrational numbers in calculations by hand. In the example below, multiplying by \displaystyle 1=\frac{\sqrt{2}}{\sqrt{2}} , one obtains

\displaystyle \frac{1}{\sqrt{2}} 
 = \frac{1\cdot\sqrt{2}}{\sqrt{2}\cdot\sqrt{2}}
 = \frac{\sqrt{2}}{2}

which usually is preferable.

In other cases, we can use the difference of two squares i.e \displaystyle (a+b)(a-b) = a^2 – b^2 to eliminate the root from the denominator. Indeed, if we multiply both the numerator and denominator by the denominator´s “conjugate” expression, then the root in the denominator gets squared and hence is removed. For example,

\displaystyle \begin{align*} 
   \frac{\sqrt{3}}{\sqrt{2}+1}
     &= \frac{\sqrt{3}}{\sqrt{2}+1} \cdot \frac{\sqrt{2}-1}{\sqrt{2}-1}
      = \frac{\sqrt{3}\,(\sqrt{2}-1)}{(\sqrt{2}+1)(\sqrt{2}-1)}\\[4pt]
     &= \frac{\sqrt{3}\cdot\sqrt{2} - \sqrt{3}\cdot1}{(\sqrt{2}\,)^2 - 1^2 }
      = \frac{\sqrt{3 \cdot 2} - \sqrt{3}}{ 2 - 1 }
      = \frac{\sqrt{6} - \sqrt{3}}{ 1 }
      = \sqrt{6} - \sqrt{3}\mbox{.}
 \end{align*}

Example 6

  1. \displaystyle \frac{10\sqrt{3}}{\sqrt{5}} = \frac{10\sqrt{3}\cdot\sqrt{5}}{\sqrt{5}\cdot\sqrt{5}} = \frac{10\sqrt{15}}{5} = 2\sqrt{15}
  2. \displaystyle \frac{1+\sqrt{3}}{\sqrt{2}} = \frac{(1+\sqrt{3})\cdot\sqrt{2}}{\sqrt{2}\cdot\sqrt{2}} = \frac{\sqrt{2}+\sqrt{6}}{2}
  3. \displaystyle \frac{3}{\sqrt{2}-2} = \frac{3(\sqrt{2}+2)}{(\sqrt{2}-2)(\sqrt{2}+2)} = \frac{3\sqrt{2}+6}{(\sqrt{2}\,)^2-2^2} = \frac{3\sqrt{2}+6}{2-4} = -\frac{3\sqrt{2}+6}{2}
  4. \displaystyle \frac{\sqrt{2}}{\sqrt{6}+\sqrt{3}} = \frac{\sqrt{2}\,(\sqrt{6}-\sqrt{3}\,)}{(\sqrt{6}+\sqrt{3}\,) (\sqrt{6}-\sqrt{3}\,)} = \frac{\sqrt{2}\,\sqrt{6}-\sqrt{2}\,\sqrt{3}}{(\sqrt{6}\,)^2 -(\sqrt{3}\,)^2}\vphantom{\Biggl(}
    \displaystyle \phantom{\frac{\sqrt{2}}{\sqrt{6}+\sqrt{3}}\vphantom{\Biggl(}}{} = \frac{\sqrt{2}\,\sqrt{2\cdot 3}-\sqrt{2}\,\sqrt{3}}{6-3} = \frac{2\sqrt{3}-\sqrt{2}\,\sqrt{3}}{3} = \frac{(2-\sqrt{2}\,)\sqrt{3}}{3} \vphantom{\displaystyle\frac{a^{\textstyle b^{\textstyle c}}}{b}}


Exercises


Study advice

Basic and final tests

After you have read the text and worked through the exercises, you should do the basic and final tests to pass this section. You can find the link to the tests in your student lounge.

Keep in mind that...

The square root of a number is always non-negative (that is, positive or zero)!

Rules for roots are actually a special case of laws of exponents, since, for example, \displaystyle \sqrt{x} = x^{1/2}.


Reviews

For those of you who want to deepen your understanding or need more detailed explanations consider the following references

Learn more about square roots from Wikipedia

How do we know that the square root of 2 is not a fraction?


Useful web sites

How to find the root of a number, without the help of calculators?

Retrieved from "http://wiki.math.se/wikis/2008/bridgecourse1-ImperialCollege/index.php/3.1_Roots"