2.1 Exercises
From Förberedande kurs i matematik 1
| (23 intermediate revisions not shown.) | |||
| Line 2: | Line 2: | ||
{| border="0" cellspacing="0" cellpadding="0" height="30" width="100%" | {| border="0" cellspacing="0" cellpadding="0" height="30" width="100%" | ||
| style="border-bottom:1px solid #000" width="5px" | | | style="border-bottom:1px solid #000" width="5px" | | ||
| - | {{ | + | {{Not selected tab|[[2.1 Algebraic expressions|Theory]]}} |
| - | {{ | + | {{Selected tab|[[2.1 Exercises|Exercises]]}} |
| style="border-bottom:1px solid #000" width="100%"| | | style="border-bottom:1px solid #000" width="100%"| | ||
|} | |} | ||
| - | === | + | ===Exercise 2.1:1=== |
<div class="ovning"> | <div class="ovning"> | ||
| - | + | Expand | |
{| width="100%" cellspacing="10px" | {| width="100%" cellspacing="10px" | ||
|a) | |a) | ||
| Line 31: | Line 31: | ||
||<math> (5x^3+3x^5)^2</math> | ||<math> (5x^3+3x^5)^2</math> | ||
|} | |} | ||
| - | </div>{{#NAVCONTENT: | + | </div>{{#NAVCONTENT:Answer|Answer 2.1:1|Solution a|Solution 2.1:1a|Solution b|Solution 2.1:1b|Solution c|Solution 2.1:1c|Solution d|Solution 2.1:1d|Solution e|Solution 2.1:1e|Solution f|Solution 2.1:1f|Solution g|Solution 2.1:1g|Solution h|Solution 2.1:1h}} |
| - | === | + | ===Exercise 2.1:2=== |
<div class="ovning"> | <div class="ovning"> | ||
| - | + | Expand | |
{| width="100%" cellspacing="10px" | {| width="100%" cellspacing="10px" | ||
|a) | |a) | ||
| Line 51: | Line 51: | ||
||<math> (a+b)^2+(a-b)^2</math> | ||<math> (a+b)^2+(a-b)^2</math> | ||
|} | |} | ||
| - | </div>{{#NAVCONTENT: | + | </div>{{#NAVCONTENT:Answer|Answer 2.1:2|Solution a|Solution 2.1:2a|Solution b|Solution 2.1:2b|Solution c|Solution 2.1:2c|Solution d|Solution 2.1:2d|Solution e|Solution 2.1:2e}} |
| - | === | + | ===Exercise 2.1:3=== |
<div class="ovning"> | <div class="ovning"> | ||
| - | + | Factorise and simplify as much as possible | |
{| width="100%" cellspacing="10px" | {| width="100%" cellspacing="10px" | ||
|a) | |a) | ||
| Line 71: | Line 71: | ||
||<math> 16x^2+8x+1</math> | ||<math> 16x^2+8x+1</math> | ||
|} | |} | ||
| - | </div>{{#NAVCONTENT: | + | </div>{{#NAVCONTENT:Answer|Answer 2.1:3|Solution a|Solution 2.1:3a|Solution b|Solution 2.1:3b|Solution c|Solution 2.1:3c|Solution d|Solution 2.1:3d|Solution e|Solution 2.1:3e|Solution f|Solution 2.1:3f}} |
| - | === | + | ===Exercise 2.1:4=== |
<div class="ovning"> | <div class="ovning"> | ||
| - | + | Determine the coefficients in front of <math>\,x\,</math> and <math>\,x^2\</math> when the following expressions are expanded out. | |
{| width="100%" cellspacing="10px" | {| width="100%" cellspacing="10px" | ||
|a) | |a) | ||
| Line 87: | Line 87: | ||
|- | |- | ||
|} | |} | ||
| - | </div>{{#NAVCONTENT: | + | </div>{{#NAVCONTENT:Answer|Answer 2.1:4|Solution a|Solution 2.1:4a|Solution b|Solution 2.1:4b|Solution c|Solution 2.1:4c}} |
| - | === | + | ===Exercise 2.1:5=== |
<div class="ovning"> | <div class="ovning"> | ||
| - | + | Simplify as much as possible | |
{| width="100%" cellspacing="10px" | {| width="100%" cellspacing="10px" | ||
|a) | |a) | ||
| Line 103: | Line 103: | ||
|| <math>\displaystyle \frac{(y^2+4y+4)(2y-4)}{(y^2+4)(y^2-4)}</math> | || <math>\displaystyle \frac{(y^2+4y+4)(2y-4)}{(y^2+4)(y^2-4)}</math> | ||
|} | |} | ||
| - | </div>{{#NAVCONTENT: | + | </div>{{#NAVCONTENT:Answer|Answer 2.1:5|Solution a|Solution 2.1:5a|Solution b|Solution 2.1:5b|Solution c|Solution 2.1:5c|Solution d|Solution 2.1:5d}} |
| - | === | + | ===Exercise 2.1:6=== |
<div class="ovning"> | <div class="ovning"> | ||
| - | + | Simplify as much as possible | |
{| width="100%" cellspacing="10px" | {| width="100%" cellspacing="10px" | ||
|a) | |a) | ||
| Line 119: | Line 119: | ||
|| <math>\displaystyle\frac{a-b+\displaystyle\frac{b^2}{a+b}}{1-\left(\displaystyle\frac{a-b}{a+b}\right)^2}</math> | || <math>\displaystyle\frac{a-b+\displaystyle\frac{b^2}{a+b}}{1-\left(\displaystyle\frac{a-b}{a+b}\right)^2}</math> | ||
|} | |} | ||
| - | </div>{{#NAVCONTENT: | + | </div>{{#NAVCONTENT:Answer|Answer 2.1:6|Solution a|Solution 2.1:6a|Solution b|Solution 2.1:6b|Solution c|Solution 2.1:6c|Solution d|Solution 2.1:6d}} |
| - | === | + | ===Exercise 2.1:7=== |
<div class="ovning"> | <div class="ovning"> | ||
| - | + | Simplify the following by writing them as a single ordinary fraction | |
| - | {| width="100" cellspacing="10px" | + | {| width="100%" cellspacing="10px" |
| + | |a) | ||
| + | |width="33%" | <math>\displaystyle \frac{2}{x+3}-\frac{2}{x+5}</math> | ||
| + | |b) | ||
| + | |width="33%" | <math>x+\displaystyle \frac{1}{x-1}+\displaystyle \frac{1}{x^2}</math> | ||
| + | |c) | ||
| + | |width="33%" | <math>\displaystyle \frac{ax}{a+1}-\displaystyle \frac{ax^2}{(a+1)^2}</math> | ||
| + | |} | ||
| + | </div>{{#NAVCONTENT:Answer|Answer 2.1:7|Solution a|Solution 2.1:7a|Solution b|Solution 2.1:7b|Solution c|Solution 2.1:7c}} | ||
| + | |||
| + | ===Exercise 2.1:8=== | ||
| + | <div class="ovning"> | ||
| + | Simplify the following fractions by writing them as a single ordinary | ||
| + | {| width="100%" cellspacing="10px" | ||
|a) | |a) | ||
| - | |width="33" | <math>\displaystyle \frac{ | + | |width="33%" | <math>\displaystyle \frac{\displaystyle\ \frac{x}{x+1}\ }{\ 3+x\ }</math> |
|b) | |b) | ||
| - | || <math> | + | |width="33%" | <math>\displaystyle \frac{\displaystyle \frac{3}{x}-\displaystyle \frac{1}{x}}{\displaystyle \frac{1}{x-3}}</math> |
|c) | |c) | ||
| - | || <math>\displaystyle \frac{ | + | |width="33%" | <math>\displaystyle \frac{1}{1+\displaystyle \frac{1}{1+\displaystyle \frac{1}{1+x}}}</math> |
|} | |} | ||
| - | </div>{{#NAVCONTENT: | + | </div>{{#NAVCONTENT:Answer|Answer 2.1:8|Solution a|Solution 2.1:8a|Solution b|Solution 2.1:8b|Solution c|Solution 2.1:8c}} |
Current revision
| Theory | Exercises |
Exercise 2.1:1
Expand
| a) | | b) | | c) | |
| d) |  y1−1xy+1 y1−1xy+1 | e) | f) | ||
| g) | h) |
Answer | Solution a | Solution b | Solution c | Solution d | Solution e | Solution f | Solution g | Solution h
Exercise 2.1:2
Expand
| a) | | b) | |
| c) | | d) | |
| e) |
Answer | Solution a | Solution b | Solution c | Solution d | Solution e
Exercise 2.1:3
Factorise and simplify as much as possible
| a) | | b) | | c) | |
| d) | | e) | f) |
Answer | Solution a | Solution b | Solution c | Solution d | Solution e | Solution f
Exercise 2.1:4
Determine the coefficients in front of
| a) | |
| b) | |
| c) | |
Answer | Solution a | Solution b | Solution c
Exercise 2.1:5
Simplify as much as possible
| a) | | b) | |
| c) | | d) | |
Answer | Solution a | Solution b | Solution c | Solution d
Exercise 2.1:6
Simplify as much as possible
| a) | 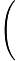 x−y+x2y−x x−y+x2y−x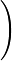  y2x−y−1 y2x−y−1 | b) | |
| c) | | d) | \displaystyle \displaystyle\frac{a-b+\displaystyle\frac{b^2}{a+b}}{1-\left(\displaystyle\frac{a-b}{a+b}\right)^2} |
Answer | Solution a | Solution b | Solution c | Solution d
Exercise 2.1:7
Simplify the following by writing them as a single ordinary fraction
| a) | \displaystyle \displaystyle \frac{2}{x+3}-\frac{2}{x+5} | b) | \displaystyle x+\displaystyle \frac{1}{x-1}+\displaystyle \frac{1}{x^2} | c) | \displaystyle \displaystyle \frac{ax}{a+1}-\displaystyle \frac{ax^2}{(a+1)^2} |
Answer | Solution a | Solution b | Solution c
Exercise 2.1:8
Simplify the following fractions by writing them as a single ordinary
| a) | \displaystyle \displaystyle \frac{\displaystyle\ \frac{x}{x+1}\ }{\ 3+x\ } | b) | \displaystyle \displaystyle \frac{\displaystyle \frac{3}{x}-\displaystyle \frac{1}{x}}{\displaystyle \frac{1}{x-3}} | c) | \displaystyle \displaystyle \frac{1}{1+\displaystyle \frac{1}{1+\displaystyle \frac{1}{1+x}}} |
Answer | Solution a | Solution b | Solution c
 Loading...
Loading...