From Förberedande kurs i matematik 1

Revision as of 10:16, 19 August 2008 (edit) Madde (Talk | contribs) ← Previous diff		Current revision (11:31, 10 October 2008) (edit) (undo) Tek (Talk | contribs) m
(3 intermediate revisions not shown.)
Line 1:		Line 1:
-	{{NAVCONTENT_START}}	+	Using the formula for double angles on <math>\sin 160^{\circ}</math> gives
-	<center> [[Bild:4_3_9-1(2).gif]] </center>	+
-	{{NAVCONTENT_STOP}}	+
-	{{NAVCONTENT_START}}	+
-	<center> [[Bild:4_3_9-2(2).gif]] </center>	+
-	{{NAVCONTENT_STOP}}	+
-	[[Bild:4_3_9.gif|center]]	+	{{Displayed math||<math>\sin 160^{\circ} = 2\cos 80^{\circ}\sin 80^{\circ}\,\textrm{.}</math>}}
		+
		+	On the right-hand side, we see that the factor <math>\cos 80^{\circ}</math> has appeared, and if we use the formula for double angles on the second factor (<math>\sin 80^{\circ}</math>),
		+
		+	{{Displayed math||<math>2\cos 80^{\circ}\sin 80^{\circ} = 2\cos 80^{\circ}\cdot 2\cos 40^{\circ}\sin 40^{\circ}\,,</math>}}
		+
		+	we obtain a further factor <math>\cos 40^{\circ}</math>. A final application of the formula for double angles on <math>\sin 40^{\circ }</math> gives us all three cosine factors,
		+
		+	{{Displayed math||<math>2\cos 80^{\circ}\cdot 2\cos 40^{\circ}\cdot\sin 40^{\circ} = 2\cos 80^{\circ}\cdot 2\cos 40^{\circ}\cdot 2\cos 20^{\circ}\sin 20^{\circ}\,\textrm{·}</math>}}
		+
		+	We have thus succeeded in showing that
		+
		+	{{Displayed math||<math>\sin 160^{\circ} = 8\cos 80^{\circ}\cdot \cos 40^{\circ}\cdot \cos 20^{\circ}\cdot\sin 20^{\circ}</math>}}
		+
		+	which can also be written as
		+
		+	{{Displayed math||<math>\cos 80^{\circ}\cdot\cos 40^{\circ}\cdot \cos 20^{\circ} = \frac{\sin 160^{\circ}}{8\sin 20^{\circ}}\,\textrm{.}</math>}}
		+
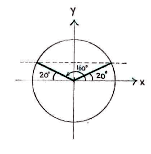
		+	[[Image:4_3_9.gif||right]]
		+	If we draw the unit circle, we see that <math>160^{\circ}</math> makes an angle of
		+	<math>20^{\circ}</math> with the negative ''x''-axis, and therefore the angles
		+	<math>20^{\circ}</math> and <math>160^{\circ}</math> have the same ''y''-coordinate in the unit circle, i.e.
		+
		+	<center><math>\sin 20^{\circ} = \sin 160^{\circ}\,\textrm{.}</math></center>
		+
		+	This shows that
		+
		+	<center><math>\cos 80^{\circ} \cos 40^{\circ} \cos 20^{\circ} = \frac{\sin 160^{\circ}}{8\sin 20^{\circ}} = \frac{1}{8}\,\textrm{.}</math></center>

---

Current revision

Using the formula for double angles on \displaystyle \sin 160^{\circ} gives

\displaystyle \sin 160^{\circ} = 2\cos 80^{\circ}\sin 80^{\circ}\,\textrm{.}

On the right-hand side, we see that the factor \displaystyle \cos 80^{\circ} has appeared, and if we use the formula for double angles on the second factor (\displaystyle \sin 80^{\circ}),

\displaystyle 2\cos 80^{\circ}\sin 80^{\circ} = 2\cos 80^{\circ}\cdot 2\cos 40^{\circ}\sin 40^{\circ}\,,

we obtain a further factor \displaystyle \cos 40^{\circ}. A final application of the formula for double angles on \displaystyle \sin 40^{\circ } gives us all three cosine factors,

\displaystyle 2\cos 80^{\circ}\cdot 2\cos 40^{\circ}\cdot\sin 40^{\circ} = 2\cos 80^{\circ}\cdot 2\cos 40^{\circ}\cdot 2\cos 20^{\circ}\sin 20^{\circ}\,\textrm{·}

We have thus succeeded in showing that

\displaystyle \sin 160^{\circ} = 8\cos 80^{\circ}\cdot \cos 40^{\circ}\cdot \cos 20^{\circ}\cdot\sin 20^{\circ}

which can also be written as

\displaystyle \cos 80^{\circ}\cdot\cos 40^{\circ}\cdot \cos 20^{\circ} = \frac{\sin 160^{\circ}}{8\sin 20^{\circ}}\,\textrm{.}

If we draw the unit circle, we see that \displaystyle 160^{\circ} makes an angle of \displaystyle 20^{\circ} with the negative x-axis, and therefore the angles \displaystyle 20^{\circ} and \displaystyle 160^{\circ} have the same y-coordinate in the unit circle, i.e.

\displaystyle \sin 20^{\circ} = \sin 160^{\circ}\,\textrm{.}

This shows that

\displaystyle \cos 80^{\circ} \cos 40^{\circ} \cos 20^{\circ} = \frac{\sin 160^{\circ}}{8\sin 20^{\circ}} = \frac{1}{8}\,\textrm{.}