Solution 4.2:9
From Förberedande kurs i matematik 1
m (Robot: Automated text replacement (-[[Bild: +[[Image:)) |
m |
||
| (2 intermediate revisions not shown.) | |||
| Line 1: | Line 1: | ||
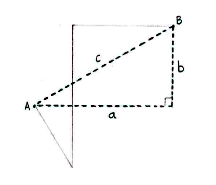
| - | + | If we introduce the dashed triangle below, the distance as the crow flies between A and B is equal to the triangle's hypotenuse, ''c''. | |
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
[[Image:4_2_9_1.gif|center]] | [[Image:4_2_9_1.gif|center]] | ||
| + | |||
| + | One way to determine the hypotenuse is to know the triangle's opposite and adjacent sides, since the Pythagorean theorem then gives | ||
| + | |||
| + | {{Displayed math||<math>c^2 = a^2 + b^2\,\textrm{.}</math>}} | ||
| + | |||
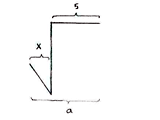
| + | In turn, we can determine the opposite and adjacent by introducing another triangle APR, where R is the point on the line PQ which the dashed triangle's side of length ''a'' cuts the line. | ||
| + | |||
[[Image:4_2_9_2.gif|center]] | [[Image:4_2_9_2.gif|center]] | ||
| - | [[Image:4_2_9_3.gif|center]] | + | |
| + | Because we know that <math>\text{AP}=4</math> and the angle at P, simple trigonometry shows that ''x'' and ''y'' are given by | ||
| + | |||
| + | {{Displayed math||<math>\begin{align} | ||
| + | x &= 4\sin 30^{\circ } = 4\cdot \frac{1}{2} = 2,\\[5pt] | ||
| + | y &= 4\cos 30^{\circ } = 4\cdot \frac{\sqrt{3}}{2} = 2\sqrt{3}\,\textrm{.} | ||
| + | \end{align}</math>}} | ||
| + | |||
| + | We can now start to look for the solution. Since ''x'' and ''y'' have been calculated, we can determine ''a'' and ''b'' by considering the horizontal and vertical distances in the figure. | ||
| + | |||
| + | {| align="center" | ||
| + | | align="center" |[[Image:4_2_9_3-1.gif]] | ||
| + | | width="20px"| | ||
| + | | align="center" |[[Image:4_2_9_3-2.gif]] | ||
| + | |- | ||
| + | | align="center" valign="top"|<math>\begin{align}a &= x+5\\ &= 2+5 = 7\end{align}</math> | ||
| + | || | ||
| + | | align="center" valign="top"|<math>\begin{align}b &= 12-y\\ &= 12-2\sqrt{3}\end{align}</math> | ||
| + | |} | ||
| + | |||
| + | With ''a'' and ''b'' given, the Pythagorean theorem leads to | ||
| + | |||
| + | {{Displayed math||<math>\begin{align} | ||
| + | c &= \sqrt{a^2+b^2}\\[5pt] | ||
| + | &= \sqrt{7^2+(12-2\sqrt{3})^2}\\[5pt] | ||
| + | &= \sqrt{49+(12^2-2\cdot 12\cdot 2\sqrt{3}+(2\sqrt{3})^2)}\\[5pt] | ||
| + | &= \sqrt{205-38\sqrt{3}}\\[5pt] | ||
| + | &\approx 11\textrm{.}0\ \text{km}\textrm{.} | ||
| + | \end{align}</math>}} | ||
Current revision
If we introduce the dashed triangle below, the distance as the crow flies between A and B is equal to the triangle's hypotenuse, c.
One way to determine the hypotenuse is to know the triangle's opposite and adjacent sides, since the Pythagorean theorem then gives
In turn, we can determine the opposite and adjacent by introducing another triangle APR, where R is the point on the line PQ which the dashed triangle's side of length a cuts the line.
Because we know that
 =4 =4 21=2 21=2 =4cos30 =4cos30 =4 =4 2 2 3=2 3=2 3. 3. |
We can now start to look for the solution. Since x and y have been calculated, we can determine a and b by considering the horizontal and vertical distances in the figure.
| 
| |
 3 3 |
With a and b given, the Pythagorean theorem leads to
 a2+b2= a2+b2= 72+(12−2 72+(12−2 3)2= 3)2= 49+(122−2 49+(122−2 12 12 2 2 3+(2 3+(2 3)2)= 3)2)= 205−38 205−38 3 3 11.0 km. 11.0 km. |