Solution 2.2:9a

From Förberedande kurs i matematik 1

(Difference between revisions)
Jump to: navigation, search
Current revision (13:58, 24 September 2008) (edit) (undo)
m
 
Line 1: Line 1:
-
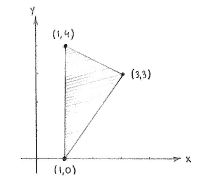
We can start by drawing the points
+
We can start by drawing the points (1,4), (3,3) and (1,0) in a coordinate system and draw lines between them, so that we get a picture of how the triangle looks like.
-
<math>\left( 1 \right.,\left. 4 \right)</math>,
+
 
-
<math>\left( 3 \right.,\left. 3 \right)</math>
+
-
and
+
-
<math>\left( 1 \right.,\left. 0 \right)</math>
+
-
in a coordinate system and draw lines between them, so that we get a picture of how thetriangle looks.
+
-
{{NAVCONTENT_START}}
 
[[Image:2_2_9_a-1(2).gif|center]]
[[Image:2_2_9_a-1(2).gif|center]]
-
{{NAVCONTENT_STOP}}
 
If we now think of how we should use the fact that the area of a triangle is given by the formula
If we now think of how we should use the fact that the area of a triangle is given by the formula
-
Area=
+
{{Displayed math||<math>\text{Area} = \frac{1}{2}\cdot\text{(base)}\cdot\text{(height),}</math>}}
-
<math>\frac{1}{2}</math>
+
-
(base)∙(height),
+
-
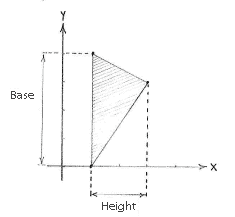
it is clear that it is most appropriate to use the edge from
+
it is clear that it is most appropriate to use the edge from (1,0) to (1,4) as the base of the triangle. The base is then parallel with the ''y''-axis and we can read off its length as the difference in the ''y''-coordinate between the corner points (1,0) and (1,4), i.e.
-
<math>\left( 1 \right.,\left. 0 \right)</math>
+
-
to
+
-
<math>\left( 1 \right.,\left. 4 \right)</math>
+
-
as the base of the triangle.
+
-
The base is then parallel with the y-axis and we can read off its length as the difference in the
+
-
<math>y</math>
+
{{Displayed math||<math>\text{base} = 4-0 = 4\,\textrm{.}</math>}}
-
-coordinate between the corner points
+
-
<math>\left( 1 \right.,\left. 0 \right)</math>
+
-
and
+
-
<math>\left( 1 \right.,\left. 4 \right)</math>, i.e.
+
-
base
+
In addition, the triangle's height is the horizontal distance from the third corner point (3,3) to the base and we can read that off as the difference in the ''x''-direction between (3,3) and the line <math>x=1</math>, i.e.
-
<math>=4-0=0</math>.
+
-
In addition, the triangle's height is the horizontal distance from the third corner point
+
-
<math>\left( 3 \right.,\left. 3 \right)</math>
+
-
to the base and we can read that off as the difference in the
+
-
<math>x</math>
+
-
-direction between
+
-
<math>\left( 3 \right.,\left. 3 \right)</math>
+
-
and the line
+
-
<math>x=1</math>, i.e.
+
-
height
+
{{Displayed math||<math>\text{height} = 3-1 = 2\,\textrm{.}</math>}}
-
<math>=3-1=2</math>.
+
-
{{NAVCONTENT_START}}
 
[[Image:2_2_9_a-2(2).gif|center]]
[[Image:2_2_9_a-2(2).gif|center]]
-
{{NAVCONTENT_STOP}}
 
Thus, the triangle's area is
Thus, the triangle's area is
-
Area=
+
{{Displayed math||<math>\text{Area} = \tfrac{1}{2}\cdot\textrm{(base)}\cdot\textrm{(height)} = \tfrac{1}{2}\cdot 4\cdot 2 = 4\,\text{u.a.}</math>}}
-
<math>\frac{1}{2}</math>
+
-
(base)∙(height)
+
-
<math>=\frac{1}{2}\centerdot 4\centerdot 2=4</math>
+
-
= area units.
+

Current revision

We can start by drawing the points (1,4), (3,3) and (1,0) in a coordinate system and draw lines between them, so that we get a picture of how the triangle looks like.



If we now think of how we should use the fact that the area of a triangle is given by the formula

\displaystyle \text{Area} = \frac{1}{2}\cdot\text{(base)}\cdot\text{(height),}

it is clear that it is most appropriate to use the edge from (1,0) to (1,4) as the base of the triangle. The base is then parallel with the y-axis and we can read off its length as the difference in the y-coordinate between the corner points (1,0) and (1,4), i.e.

\displaystyle \text{base} = 4-0 = 4\,\textrm{.}

In addition, the triangle's height is the horizontal distance from the third corner point (3,3) to the base and we can read that off as the difference in the x-direction between (3,3) and the line \displaystyle x=1, i.e.

\displaystyle \text{height} = 3-1 = 2\,\textrm{.}



Thus, the triangle's area is

\displaystyle \text{Area} = \tfrac{1}{2}\cdot\textrm{(base)}\cdot\textrm{(height)} = \tfrac{1}{2}\cdot 4\cdot 2 = 4\,\text{u.a.}
Retrieved from "http://wiki.math.se/wikis/2008/bridgecourse1-ImperialCollege/index.php/Solution_2.2:9a"