Processing Math: Done

From Förberedande kurs i matematik 1

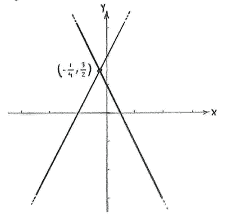
The lines have a point of intersection at that point which simultaneously satisfies the equations of both lines:

2x+y−1=0 and y−2x−2=0.

If we make y the subject of the second equation y−2x−2=0 and substitute it into the first equation, we obtain an equation which only contains x,

2x+2x+2−1=0  4x+1=0 

which gives that x=−14. Then, from the relation y=2x+2, we obtain y=2−14+2=32 .

The point of intersection is −4123 .

We check for safety's sake that −4123  really satisfies both equations:

We check for safety's sake that −4123  really satisfies both equations:

2x+y−1=0: LHS = 2−41+23−1=−21+23−22=0  =RHS

y−2x−2=0: LHS = 23−2−41−2=23+21−24=0  =RHS