Loading http://wiki.math.se/jsMath/fonts/msam10/def.js

From Förberedande kurs i matematik 1

The idea is first to find the general solution to the equation and then to see which angles lie between 0 and 360.

If we start by considering the expression 2v+10 as an unknown, then we have a usual basic trigonometric equation. One solution which we can see directly is

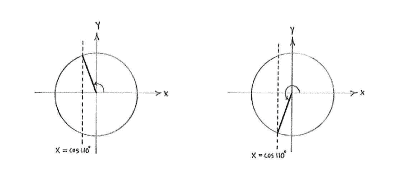
2v+10=110

There is then a further solution which satisfies 02v+10360, where 2v+10 lies in the third quadrant and makes the same angle with the negative y-axis as 100 makes with the positive y -axis, i.e. 2v+10 makes an angle 110−90=20  with the negative y -axis and consequently

2v+10=270−20=250

There is then a further solution which satisfies 02v+10360, where 2v+10 lies in the third quadrant and makes the same angle with the negative y-axis as 100 makes with the positive y -axis, i.e. 2v+10 makes an angle 110−90=20  with the negative y -axis and consequently

2v+10=270−20=250

FIGURE1 FIGURE2

Now it is easy to write down the general solution,

\displaystyle \text{2}v+\text{1}0^{\circ }=110^{\circ }+n\centerdot 360^{\circ } and

\displaystyle \text{2}v+\text{1}0^{\circ }=250^{\circ }+n\centerdot 360^{\circ }

and if we make \displaystyle v the subject, we get

\displaystyle v=50^{\circ }+n\centerdot 180^{\circ } and

\displaystyle v=120^{\circ }+n\centerdot 180^{\circ } EQ6

For different values of the integers \displaystyle n, we see that the corresponding solutions are:

\displaystyle \begin{array}{*{35}l} \cdots \cdots & \cdots \cdots & \cdots \cdots \\ n=-2 & v=50^{\circ }-2\centerdot 180^{\circ }=-310^{\circ } & v=120^{\circ }-2\centerdot 180^{\circ }=-240^{\circ } \\ n=-1 & v=50^{\circ }-1\centerdot 180^{\circ }=-130^{\circ } & v=120^{\circ }-1\centerdot 180^{\circ }=-60^{\circ } \\ n=0 & v=50^{\circ }+0\centerdot 180^{\circ }=50^{\circ } & v=120^{\circ }+0\centerdot 180^{\circ }=120^{\circ } \\ n=1 & v=50^{\circ }+1\centerdot 180^{\circ }=230^{\circ } & v=120^{\circ }+1\centerdot 180^{\circ }=300^{\circ } \\ n=2 & v=50^{\circ }+2\centerdot 180^{\circ }=410^{\circ } & v=120^{\circ }+2\centerdot 180^{\circ }=480^{\circ } \\ n=3 & v=50^{\circ }+3\centerdot 180^{\circ }=590^{\circ } & v=120^{\circ }+3\centerdot 180^{\circ }=660^{\circ } \\ \cdots \cdots & \cdots \cdots & \cdots \cdots \\ \end{array}

From the table, we see that the solutions that are between \displaystyle 0^{\circ } and \displaystyle 360^{\circ } are

\displaystyle v=50,\quad v=120^{\circ },\quad v=230^{\circ } and \displaystyle v=300^{\circ }