Solution 4.4:5a

From Förberedande kurs i matematik 1

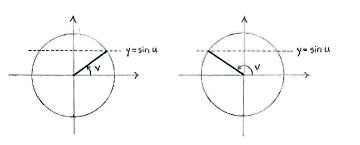
If we consider for a moment the equality

where u has a fixed value, there are usually two angles v in the unit circle which ensure that the equality holds,

v=uandv=−u.

(The only exception is when u=2 or u=32, in which case u and −u correspond to the same direction and there is only one angle v which satisfies the equality.)

We obtain all the angles v which satisfy (*) by adding multiples of 2,

v=u+2nandv=−u+2n

where n is an arbitrary integer.

If we now go back to our equation

the reasoning above shows that the equation is only satisfied when

3x=x+2nor3x=−x+2n.

If we make x the subject of each equation, we obtain the full solution to the equation,

xx=0+n=4+2n.