From Förberedande kurs i matematik 1

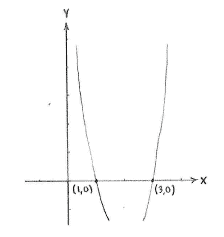
To determine all the points on the curve \displaystyle y=3x^{2}-12x+9 which also lie on the x-axis we substitute the equation of the x-axis i.e. \displaystyle y=0 in the equation of the curve and obtain that x must satisfy

\displaystyle 0 = 3x^{2}-12x+9\,\textrm{.}

After dividing by 3 and completing the square the right-hand side is

\displaystyle x^{2}-4x+3 = (x-2)^{2} - 2^{2} + 3 = (x-2)^{2} - 1

and thus the equation has solutions \displaystyle x=2\pm 1, i.e. \displaystyle x=2-1=1 and \displaystyle x=2+1=3\,.

The points where the curve cut the x-axis are (1,0) and (3,0).