Processing Math: Done
Solution 4.1:6a
From Förberedande kurs i matematik 1
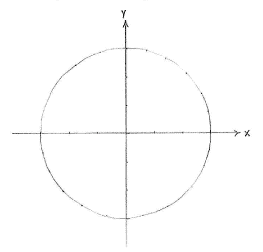
If we write the equation as
we can interpret the left-hand side as the square of the distance between the points (x,y) and (0,0). The whole equation says that the distance from a point (x,y) to the origin should be constant and equal to  9=3
9=3