Processing Math: Done
To print higher-resolution math symbols, click the
Hi-Res Fonts for Printing button on the jsMath control panel.
jsMath

Solution to Test Paper 3

From Mechanics

(Difference between revisions)
Jump to: navigation, search
Current revision (09:36, 8 April 2012) (edit) (undo)
 
(6 intermediate revisions not shown.)
Line 3: Line 3:
| 1 (a)
| 1 (a)
|<math>\begin{align}
|<math>\begin{align}
-
& 0=24.5-9.8t \\
+
& 0=24\textrm{.}5-9\textrm{.}8t \\
-
& t=\frac{24.5}{9.8}=2.5\text{ seconds} \\
+
& t=\frac{24\textrm{.}5}{9\textrm{.}8}=2\textrm{.}5\text{ seconds} \\
\end{align}</math>
\end{align}</math>
Line 13: Line 13:
| 1 (b)
| 1 (b)
| <math>\begin{align}
| <math>\begin{align}
-
& {{0}^{2}}={{24.5}^{2}}+2\times (-9.8)\times s \\
+
& {{0}^{2}}={{24\textrm{.}5}^{2}}+2\times (-9\textrm{.}8)\times s \\
-
& s=\frac{{{24.5}^{2}}}{2\times 9.8}=30.625\text{ m} \\
+
& s=\frac{{{24\textrm{.}5}^{2}}}{2\times 9\textrm{.}8}=30\textrm{.}625\text{ m} \\
 +
& \\
 +
& \text{OR} \\
 +
& \\
 +
& s=\frac{1}{2}(24\textrm{.}5+0)\times 2\textrm{.}5=30\textrm{.}625\text{ m} \\
\end{align}</math>
\end{align}</math>
- 
-
OR
 
- 
-
<p>
 
- 
-
<math>s=\frac{1}{2}(24.5+0)\times 2.5=30.625\text{ m}</math>
 
- 
-
</p>
 
| (3 marks)
| (3 marks)
Line 42: Line 38:
|-
|-
| 2 (a)
| 2 (a)
-
| <math>\begin{align}
+
| [[Image:test3ans1.gif]]
-
& T-800\times 9\textrm{.}8=800\times 0\textrm{.}2 \\
+
-
& T=\text{7840}+\text{160}=\text{8000 N} \\
+
-
\end{align}</math>
+
-
| (3 marks)
+
| (1 marks)
|-
|-
| 2 (b)
| 2 (b)
-
| <math>\begin{align}
+
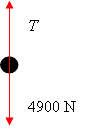
| T = 4900 N
-
& T-800\times 9\textrm{.}8=800\times (-0\textrm{.}2) \\
+
-
& T=\text{7840}-\text{160}=\text{7680 N} \\
+
-
\end{align}</math>
+
-
| (3 marks)
+
| (1 marks)
|-
|-
| 2 (c)
| 2 (c)
-
| <math>T=800\times 9\textrm{.}8=7840\text{ N}</math>
+
| <math>\begin{align}
-
| (1 mark)
+
& T-4900=500\times 0\textrm{.}6 \\
 +
& T=300+4900=5200\text{ N} \\
 +
\end{align}</math>
 +
| (3 mark)
 +
 +
|-
 +
| 2 (d)
 +
| <math>\begin{align}
 +
& 4800-4900=500a \\
 +
& a=\frac{-100}{500}=-0\textrm{.}2\text{ m}{{\text{s}}^{\text{-2}}} \\
 +
& 0\textrm{.}\text{2 m}{{\text{s}}^{-\text{2}}}\quad \text{downwards} \\
 +
\end{align}</math>
 +
 +
| (4 mark)
|-
|-
|
|
|
|
-
| '''(7 marks)'''
+
| '''(9 marks)'''
|-
|-
| 3 (a)
| 3 (a)
-
|[[Image:test1ans1.gif]]
+
|<math>R=5\times 9\textrm{.}8\cos 42{}^\circ =36\textrm{.}4\text{ N}</math>
-
| (1 mark)
+
| (2 mark)
|-
|-
| 3 (b)
| 3 (b)
-
| <math>F=2\times 1\textrm{.}8=3\textrm{.}6\text{ N}</math>
+
| <math>F=0\textrm{.}4R=14\textrm{.}6\text{ N}</math>
 +
 
| (2 marks)
| (2 marks)
Line 86: Line 90:
|-
|-
| 3 (c)
| 3 (c)
-
| <math>R=2\times 9\textrm{.}8=19\textrm{.}6\text{ N}</math>
+
| <math>\begin{align}
-
| (2 marks)
+
& 5\times 9\textrm{.}8\sin 42{}^\circ -14\textrm{.}6=5a \\
 +
& a=\frac{18\textrm{.}22}{5}=3\textrm{.}64\text{ m}{{\text{s}}^{\text{-2}}} \\
 +
\end{align}</math>
 +
 
 +
| (3 marks)
Line 93: Line 101:
| 3 (d)
| 3 (d)
| <math>\begin{align}
| <math>\begin{align}
-
& 3 \textrm{.}6=19 \textrm{.}6\mu \\
+
& 2=\frac{1}{2}\times 3\textrm{.}64{{t}^{2}} \\
-
& \mu =\frac{3 \textrm{.}6}{19 \textrm{.}6}=0 \textrm{.}184 \\
+
& t=\sqrt{\frac{4}{3\textrm{.}64}}=1\textrm{.}05\text{ seconds} \\
\end{align}</math>
\end{align}</math>
 +
 +
 +
 +
| (3 marks)
| (3 marks)
Line 102: Line 114:
|
|
|
|
-
| '''(8 marks)'''
+
| '''(10 marks)'''
|-
|-
| 4 (a)
| 4 (a)
-
| [[Image:test1ans2.gif]]
+
| <math>\begin{align}
-
| (1 mark)
+
& Q\cos 40{}^\circ =8\times 9\textrm{.}8\sin 40{}^\circ \\
 +
& Q=\frac{8\times 9\textrm{.}8\sin 40{}^\circ }{\cos 40{}^\circ }=65\textrm{.}8\text{ N} \\
 +
\end{align}</math>
 +
 
 +
| (3 mark)
|-
|-
| 4 (b)
| 4 (b)
-
| <math>\begin{align}
+
|<math>\begin{align}
-
& R+T\sin 30{}^\circ =200\times 9 \textrm{.}8 \\
+
& Q\cos 40{}^\circ +F=8\times 9\textrm{.}8\sin 40{}^\circ \\
-
& R+0 \textrm{.}5T=1960 \\
+
& Q\sin 40{}^\circ +8\times 9\textrm{.}8\cos 40{}^\circ =R \\
-
& R=1960-0 \textrm{.}5T \\
+
& Q\cos 40{}^\circ =8\times 9\textrm{.}8\sin 40{}^\circ \\
 +
& \text{ }-0\textrm{.}3(Q\sin 40{}^\circ +8\times 9\textrm{.}8\cos 40{}^\circ ) \\
 +
& Q=\frac{8\times 9\textrm{.}8\sin 40{}^\circ -0.\textrm{.}\times 8\times 9\textrm{.}8\cos 40{}^\circ }{\cos 40{}^\circ +0.\times \sin 40{}^\circ } \\
 +
& \text{ }=33\textrm{.}8\text{ N} \\
\end{align}</math>
\end{align}</math>
-
| (3 marks)
+
 
 +
| (5 marks)
-
|-
 
-
| 4 (c)
 
-
| <math></math>
 
-
<math>\begin{align}
 
-
& F=T\cos 30{}^\circ \\
 
-
& T\cos 30{}^\circ =0\textrm{.}6(1960-0\textrm{.}5T) \\
 
-
& T(\cos 30{}^\circ +0\textrm{.}3)=1176 \\
 
-
& T=\frac{1176}{(\cos 30{}^\circ +0\textrm{.}3)}=1010\text{ N} \\
 
-
\end{align}</math>
 
-
| (4 marks)
 
Line 141: Line 151:
|-
|-
| 5 (a)
| 5 (a)
-
| <math>\begin{align}
+
| <math>\mathbf{v}=(\mathbf{i}+\mathbf{j})+(0\textrm{.}05\mathbf{i}-0\textrm{.}1\mathbf{j})t</math>
-
& 5\mathbf{i}-2\mathbf{j}=4\mathbf{i}+3\mathbf{j}+10\mathbf{a} \\
+
 
-
& \mathbf{a}=\frac{1}{10}(\mathbf{i}-5\mathbf{j})=\left( 0\textrm{.}1\mathbf{i}-0\textrm{.}5\mathbf{j} \right)\text{ m}{{\text{s}}^{\text{-2}}} \\
+
-
\end{align}</math>
+
-
AG
+
| (2 marks)
-
| (3 marks)
+
|-
|-
| 5 (b)
| 5 (b)
-
| <math>\mathbf{r}=(4\mathbf{i}+3\mathbf{j})t+0\textrm{.}5(0\textrm{.}1\mathbf{i}-0\textrm{.}5\mathbf{j}){{t}^{2}}</math>
 
-
| (2 marks)
 
- 
- 
-
|-
 
-
| 5 (c)
 
| <math>\begin{align}
| <math>\begin{align}
-
& \mathbf{r}=(4t+0\textrm{.}05{{t}^{2}})\mathbf{i}+(3t-0\textrm{.}25{{t}^{2}})\mathbf{j} \\
+
& -(1+0\textrm{.}05t)=1-0\textrm{.}1t \\
-
& 3t-0\textrm{.}25{{t}^{2}}=0 \\
+
& 0\textrm{.}05t=2 \\
-
& t(3-0\textrm{.}25t)=0 \\
+
& t=40 \\
-
& t=0\text{ or }t=\frac{3}{0\textrm{.}25}=12\text{ s} \\
+
-
& t=12\text{ s} \\
+
\end{align}</math>
\end{align}</math>
-
| (3 marks)
+
 
 +
| (4 marks)
-
|-
 
-
| 5 (d)
 
-
| <math>\begin{align}
 
-
& \mathbf{v}=(4+0\textrm{.}1t)\mathbf{i}+(3-0\textrm{.}5t)\mathbf{j} \\
 
-
& 3-0\textrm{.}5t=0 \\
 
-
& t=6 \text{ s} \\
 
-
\end{align}</math>
 
-
| (3 marks)
 
Line 181: Line 173:
|
|
|
|
-
| '''(11 marks)'''
+
| '''(6 marks)'''
|}
|}
- 
-
AG: Answer Given in Question – Working must justify answer.
 

Current revision

Solutions
1 (a) 0=24.5−9.8tt=9.824.5=2.5 seconds (3 marks)


1 (b) 02=24.52+2(−9.8)ss=24.5229.8=30.625 mORs=21(24.5+0)2.5=30.625 m (3 marks)
1 (c) 61.25 m


(1 marks)
(7 marks)


2 (a) Image:test3ans1.gif (1 marks)


2 (b) T = 4900 N (1 marks)


2 (c) T−4900=5000.6T=300+4900=5200 N (3 mark)
2 (d) 4800−4900=500aa=500−100=−0.2 ms-20.2 ms−2downwards (4 mark)
(9 marks)


3 (a) R=59.8cos42=36.4 N (2 mark)


3 (b) F=0.4R=14.6 N (2 marks)


3 (c) 59.8sin42−14.6=5aa=518.22=3.64 ms-2 (3 marks)


3 (d) 2=213.64t2t=43.64=1.05 seconds  (3 marks)


(10 marks)


4 (a) Qcos40=89.8sin40Q=cos4089.8sin40=65.8 N (3 mark)


4 (b) Qcos40+F=89.8sin40Qsin40+89.8cos40=RQcos40=89.8sin40 −0.3(Qsin40+89.8cos40)Q=cos40+0sin4089.8sin40−0.89.8cos40 =33.8 N (5 marks)



(8 marks)


5 (a) v=(i+j)+(0.05i−0.1j)t


(2 marks)


5 (b) −(1+0.05t)=1−0.1t0.05t=2t=40 (4 marks)



(6 marks)


Retrieved from "http://wiki.math.se/wikis/2009/bridgecoursemechanics/index.php/Solution_to_Test_Paper_3"