Processing Math: Done
To print higher-resolution math symbols, click the
Hi-Res Fonts for Printing button on the jsMath control panel.
jsMath

13. Moments

From Mechanics

(Difference between revisions)
Jump to: navigation, search
Line 15: Line 15:
-
 
+
[[Image:T13.GIF]]
-
 
+
-
 
+
<math>\text{Moment }=Fd</math> <math>\ \ \ \ \ \ \ \ \ \text{Moment }=Fd\sin \theta </math>
-
 
+
-
 
+
-
 
+
-
 
+
-
+
-
<math>\text{Moment }=Fd</math>
+
-
+
-
<math>\text{Moment }=Fd\sin \theta </math>
+

Revision as of 16:13, 17 September 2009

       Theory          Exercises      


Key Points

The moment of the force about the point O is the product of the force and the perpendicular distance to the line of action of the force from O.


Image:T13.GIF

Moment =Fd          Moment =Fdsin


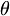
Clockwise moments are negative. Anti-clockwise moments are positive.


Example 11.1 Find the moment of each force shown below about the point O.

(a) (b)





Solution (a) (b)

2008=16

1212=144


Moment is -16 Nm Moment is 14.4 Nm

Example 13.2 Find the moment of each force shown below about the point O. (a) (b)




Solution (a) (b)






403sin60=104

1002sin20=684


Moment is 104 Nm (to 3 sf) Moment is 68.4 Nm (to 3 sf)

Example 13.3 For the rectangular lamina shown below, find the total moment of the forces acting, about the corner marked O.






Solution

Force Moment 5N at O 50=0

8 N −812=−96

7 N 70=0

6 N −605=−3

5 N 512=6

4 N 405=2

Total Moment 0−96+0−3+6+2=−46 Nm

Retrieved from "http://wiki.math.se/wikis/2009/bridgecoursemechanics/index.php/13._Moments"