4. Forces and Vectors
From Mechanics
(New page: 4. Forces and Vectors Key Points <math>\begin{align} & \mathbf{F}=F\cos \alpha \mathbf{i}+F\cos (90-\alpha )\mathbf{j} \\ & =F\cos \alpha \mathbf{i}+F\sin \alpha \mathbf{j} \end{al...) |
|||
| Line 1: | Line 1: | ||
| - | 4. Forces and Vectors | ||
| - | |||
| - | Key Points | ||
| - | |||
| - | |||
| - | |||
| - | |||
<math>\begin{align} | <math>\begin{align} | ||
& \mathbf{F}=F\cos \alpha \mathbf{i}+F\cos (90-\alpha )\mathbf{j} \\ | & \mathbf{F}=F\cos \alpha \mathbf{i}+F\cos (90-\alpha )\mathbf{j} \\ | ||
| Line 30: | Line 23: | ||
is called the vertical component of the force. | is called the vertical component of the force. | ||
| - | + | '''[[Example 4.1]]''' | |
| - | + | ||
| - | Example 4.1 | + | |
Express each of the forces given below in the form ai + bj. | Express each of the forces given below in the form ai + bj. | ||
| Line 40: | Line 31: | ||
| - | + | '''Solution''' | |
| - | Solution | + | |
(a) | (a) | ||
| Line 55: | Line 45: | ||
Note the negative sign here in the first term. | Note the negative sign here in the first term. | ||
| - | Example 4.2 | + | '''[[Example 4.2]]''' |
Express the force shown below as a vector in terms of i and j. | Express the force shown below as a vector in terms of i and j. | ||
| Line 63: | Line 53: | ||
| - | Solution | + | '''Solution''' |
| Line 71: | Line 61: | ||
Note the negative sign in the second term. | Note the negative sign in the second term. | ||
| - | Example 4.3 | + | '''[[Example 4.3]]''' |
| Line 77: | Line 67: | ||
| - | Solution | + | '''Solution''' |
| Line 85: | Line 75: | ||
Note that here both terms are negative. | Note that here both terms are negative. | ||
| - | Example 4.4 | + | '''[[Example 4.4]]''' |
Find the magnitude of the force (4i - 8j) N. Draw a diagram to show the direction of this force. | Find the magnitude of the force (4i - 8j) N. Draw a diagram to show the direction of this force. | ||
| - | Solution | + | '''Solution''' |
The magnitude, FN , of the force is given by, | The magnitude, FN , of the force is given by, | ||
| Line 96: | Line 86: | ||
| - | The angle, | + | The angle, <math>\theta </math>, is given by, |
<math>\theta ={{\tan }^{-1}}\left( \frac{8}{4} \right)=63.4{}^\circ </math> | <math>\theta ={{\tan }^{-1}}\left( \frac{8}{4} \right)=63.4{}^\circ </math> | ||
| - | Example 4.5 | + | '''[[Example 4.5]]''' |
Find the magnitude and direction of the resultant of the four forces shown in the diagram. | Find the magnitude and direction of the resultant of the four forces shown in the diagram. | ||
| Line 108: | Line 98: | ||
| - | Solution | + | '''Solution''' |
Force Vector Form | Force Vector Form | ||
| Line 138: | Line 128: | ||
| - | The angle | + | The angle <math>\theta </math> can be found using tan. |
Revision as of 15:48, 18 March 2009
 i+Fcos(90−
i+Fcos(90− )j=Fcos
)j=Fcos i+Fsin
i+Fsin j
j




Express each of the forces given below in the form ai + bj.
Solution
(a)
 i+20sin40
i+20sin40 j
j
(b)
 i+80sin30
i+80sin30 j
j
Note the negative sign here in the first term.
Express the force shown below as a vector in terms of i and j.
Solution
 i−28sin30
i−28sin30 j
j
Note the negative sign in the second term.
Solution
 i−50sin44
i−50sin44 j
j
Note that here both terms are negative.
Find the magnitude of the force (4i - 8j) N. Draw a diagram to show the direction of this force.
Solution
The magnitude, FN , of the force is given by,
 42+82=
42+82= 80=8
80=8 94 N (to 3sf)
94 N (to 3sf)
The angle, 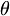
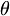 =tan−1
=tan−1 48
48 =63
=63 4
4
Find the magnitude and direction of the resultant of the four forces shown in the diagram.
Solution
Force Vector Form
20 N
 i+20sin50
i+20sin50 j
j
18 N
25 N
 i−25sin20
i−25sin20 j
j
15 N
 i+15sin30
i+15sin30 j
j
 20cos50
20cos50 −25cos20
−25cos20 −15cos30
−15cos30
 i+
i+ 20sin50
20sin50 −18−25sin20
−18−25sin20 +15sin30
+15sin30
 j=−23
j=−23 627i−3
627i−3 730j
730j
The magnitude is given by:
 23
23 6272+3
6272+3 7302=23
7302=23 9 N (to 3sf)
9 N (to 3sf)
The angle 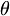
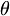 =3
=3 73023
73023 627
627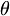 =9
=9 0
0
