Processing Math: Done
Lösung 1.2:2c
Aus Online Mathematik Brückenkurs 2
(Unterschied zwischen Versionen)
K (Lösning 1.2:2c moved to Solution 1.2:2c: Robot: moved page) |
|||
| Zeile 1: | Zeile 1: | ||
| - | {{ | + | When we see this expression, we should think "root of something", |
| - | < | + | |
| - | {{ | + | |
| + | <math>\sqrt{\left\{ \left. {} \right\} \right.}</math> | ||
| + | |||
| + | |||
| + | and in order to differentiate it, we should first differentiate the outer function , "the root of", with respect to its argument and, after that, multiply by the derivative of the inner functional expression | ||
| + | |||
| + | <math>\left\{ \left. {} \right\} \right.=\cos x</math>, | ||
| + | |||
| + | |||
| + | <math>\frac{d}{dx}\sqrt{\left\{ \left. \cos x \right\} \right.}=\frac{1}{2\sqrt{\left\{ \left. \cos x \right\} \right.}}\centerdot \left( \left\{ \left. \cos x \right\} \right. \right)^{\prime }</math>, | ||
| + | |||
| + | where we have used the differentiation rule | ||
| + | |||
| + | |||
| + | <math>\frac{d}{dx}\sqrt{x}=\frac{d}{dx}x^{{1}/{2}\;}=\frac{1}{2}x^{\frac{1}{2}-1}=\frac{1}{2}x^{-\frac{1}{2}}=\frac{1}{2\sqrt{x}}</math>. | ||
| + | |||
| + | Thus, we obtain | ||
| + | |||
| + | |||
| + | <math>\frac{d}{dx}\sqrt{\cos x}=\frac{1}{2\sqrt{\cos x}}\centerdot \left( -\sin x \right)=-\frac{\sin x}{2\sqrt{\cos x}}</math> | ||
Version vom 13:06, 11. Okt. 2008
When we see this expression, we should think "root of something",

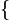
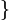
and in order to differentiate it, we should first differentiate the outer function , "the root of", with respect to its argument and, after that, multiply by the derivative of the inner functional expression
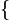
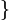 =cosx
=cosx

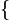 cosx
cosx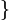 =12
=12
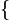 cosx
cosx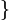


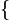 cosx
cosx


where we have used the differentiation rule
 x=ddxx1
x=ddxx1 2=21x21−1=21x−21=12
2=21x21−1=21x−21=12 x
x
Thus, we obtain
 cosx=12
cosx=12 cosx
cosx
 −sinx
−sinx =−sinx2
=−sinx2 cosx
cosx
