Processing Math: Done
To print higher-resolution math symbols, click the
Hi-Res Fonts for Printing button on the jsMath control panel.
jsMath

Lösung 1.2:2d

Aus Online Mathematik Brückenkurs 2

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
K (Lösning 1.2:2d moved to Solution 1.2:2d: Robot: moved page)
Zeile 1: Zeile 1:
-
{{NAVCONTENT_START}}
+
We can see the expression as "ln of something",
-
<center> [[Image:1_2_2d.gif]] </center>
+
 
-
{{NAVCONTENT_STOP}}
+
 
 +
<math>\ln \left\{ \left. {} \right\} \right.</math>,
 +
 
 +
where "something" is
 +
<math>\ln x</math>.
 +
 
 +
Because we have a compound expression, we use the chain rule and obtain, roughly speaking, the outer derivative multiplied by the inner derivative,
 +
 
 +
 
 +
<math>\frac{d}{dx}\ln \left\{ \left. \ln x \right\} \right.=\frac{1}{\ln x}\centerdot \left( \left\{ \left. \ln x \right\} \right. \right)^{\prime }</math>
 +
 
 +
 
 +
where the first factor on the right-hand side
 +
<math>\frac{1}{\ln x}</math>
 +
is the outer derivative of
 +
<math>\ln \left\{ \left. \ln x \right\} \right.</math>
 +
and the other factor
 +
<math>\left( \left\{ \left. \ln x \right\} \right. \right)^{\prime }</math>
 +
is the inner derivative. Thus, we get
 +
 
 +
 
 +
<math>\frac{d}{dx}\ln \left\{ \left. \ln x \right\} \right.=\frac{1}{\ln x}\centerdot \frac{1}{x}=\frac{1}{x\ln x}</math>

Version vom 13:13, 11. Okt. 2008

We can see the expression as "ln of something",


ln,

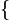
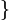
where "something" is lnx.

Because we have a compound expression, we use the chain rule and obtain, roughly speaking, the outer derivative multiplied by the inner derivative,


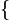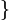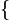ddxlnlnx=1lnxlnx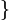 


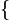where the first factor on the right-hand side 1lnx is the outer derivative of lnlnx and the other factor lnx  is the inner derivative. Thus, we get


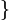
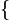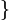
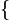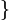ddxlnlnx=1lnxx1=1xlnx

Von „http://wiki.math.se/wikis/2009/bridgecourse2-TU-Berlin/index.php/L%C3%B6sung_1.2:2d“