Lösung 1.2:2e
Aus Online Mathematik Brückenkurs 2
K (Lösning 1.2:2e moved to Solution 1.2:2e: Robot: moved page) |
|||
| Zeile 1: | Zeile 1: | ||
| - | {{ | + | One way to differentiate the expression could be to expand |
| - | < | + | <math>\left( 2x+1 \right)^{4}</math> |
| - | {{ | + | multiply by |
| - | {{ | + | <math>x</math> |
| - | < | + | and differentiate term by term, but it is simpler instead to use the structure of the expression and differentiate step by step using the differentiation rules. |
| - | {{ | + | |
| + | To begin with, we have a product of | ||
| + | <math>x</math> | ||
| + | and | ||
| + | <math>\left( 2x+1 \right)^{4}</math> | ||
| + | so the product rule gives that | ||
| + | |||
| + | |||
| + | <math>\begin{align} | ||
| + | & \frac{d}{dx}x\left( 2x+1 \right)^{4}=\left( x \right)^{\prime }\centerdot \left( 2x+1 \right)^{4}+x\centerdot \left( \left( 2x+1 \right)^{4} \right)^{\prime } \\ | ||
| + | & =1\centerdot \left( 2x+1 \right)^{4}+x\centerdot \left( \left( 2x+1 \right)^{4} \right)^{\prime } \\ | ||
| + | \end{align}</math> | ||
| + | |||
| + | |||
| + | |||
| + | We can differentiate the expression | ||
| + | <math>\left( 2x+1 \right)^{4}</math> | ||
| + | by viewing it as "something raised to the | ||
| + | <math>\text{4}</math>", | ||
| + | |||
| + | |||
| + | <math>\left\{ \left. {} \right\} \right.^{4}</math>. | ||
| + | |||
| + | The chain rule then gives | ||
| + | |||
| + | |||
| + | <math>\begin{align} | ||
| + | & \frac{d}{dx}\left\{ \left. {} \right\} \right.^{4}=4\centerdot \left\{ \left. {} \right\} \right.^{3}\centerdot \left( \left\{ \left. {} \right\} \right. \right)^{\prime } \\ | ||
| + | & \frac{d}{dx}\left( 2x+1 \right)^{4}=4\centerdot \left( 2x+1 \right)^{3}\centerdot \left( 2x+1 \right)^{\prime } \\ | ||
| + | \end{align}</math> | ||
| + | |||
| + | |||
| + | We carry out the last differentiation directly, and obtain | ||
| + | |||
| + | |||
| + | <math>\left( 2x+1 \right)^{\prime }=2</math> | ||
| + | |||
| + | |||
| + | If we go through the whole calculation from the beginning, it is | ||
| + | |||
| + | |||
| + | <math>\begin{align} | ||
| + | & \frac{d}{dx}x\left( 2x+1 \right)^{4}=\left( x \right)^{\prime }\centerdot \left( 2x+1 \right)^{4}+x\centerdot \left( \left( 2x+1 \right)^{4} \right)^{\prime } \\ | ||
| + | & =1\centerdot \left( 2x+1 \right)^{4}+x\centerdot 4\left( 2x+1 \right)^{3}\centerdot \left( 2x+1 \right)^{\prime } \\ | ||
| + | & =\left( 2x+1 \right)^{4}+x\centerdot 4\left( 2x+1 \right)^{3}\centerdot 2 \\ | ||
| + | & =\left( 2x+1 \right)^{4}+8x\left( 2x+1 \right)^{3} \\ | ||
| + | \end{align}</math> | ||
| + | |||
| + | |||
| + | Both terms contain a common factor | ||
| + | <math>\left( 2x+1 \right)^{3}</math> | ||
| + | which we can take out to get an answer in factorized form: | ||
| + | |||
| + | |||
| + | <math>\begin{align} | ||
| + | & \frac{d}{dx}x\left( 2x+1 \right)^{4}=\left( 2x+1 \right)^{3}\left( \left( 2x+1 \right)+8x \right) \\ | ||
| + | & =\left( 2x+1 \right)^{3}\left( 10x+1 \right) \\ | ||
| + | \end{align}</math> | ||
Version vom 13:39, 11. Okt. 2008
One way to differentiate the expression could be to expand
 2x+1
2x+1 4
4
To begin with, we have a product of
 2x+1
2x+1 4
4
 2x+1
2x+1 4=
4= x
x


 2x+1
2x+1 4+x
4+x

 2x+1
2x+1 4
4
 =1
=1
 2x+1
2x+1 4+x
4+x

 2x+1
2x+1 4
4

We can differentiate the expression
 2x+1
2x+1 4
4
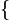
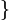 4
4
The chain rule then gives
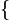
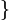 4=4
4=4
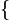
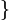 3
3

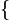
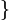

 ddx
ddx 2x+1
2x+1 4=4
4=4
 2x+1
2x+1 3
3
 2x+1
2x+1

We carry out the last differentiation directly, and obtain
 2x+1
2x+1
 =2
=2
If we go through the whole calculation from the beginning, it is
 2x+1
2x+1 4=
4= x
x


 2x+1
2x+1 4+x
4+x

 2x+1
2x+1 4
4
 =1
=1
 2x+1
2x+1 4+x
4+x 4
4 2x+1
2x+1 3
3
 2x+1
2x+1
 =
= 2x+1
2x+1 4+x
4+x 4
4 2x+1
2x+1 3
3 2=
2= 2x+1
2x+1 4+8x
4+8x 2x+1
2x+1 3
3
Both terms contain a common factor
 2x+1
2x+1 3
3
 2x+1
2x+1 4=
4= 2x+1
2x+1 3
3
 2x+1
2x+1 +8x
+8x =
= 2x+1
2x+1 3
3 10x+1
10x+1
