Lösung 3.3:1a

Aus Online Mathematik Brückenkurs 2

Version vom 10:42, 11. Mär. 2009

Powers are repeated multiplications and because multiplication is a relatively simple arithmetical operation when it is carried out in polar form, calculating powers also becomes fairly simple in polar form,

r(cos+isin)n=rn(cosn+isinn).

The equation above is called de Moivre's formula.

The plan is therefore to rewrite 1+i in polar form, raise the expression to the power 12 using de Moivre's formula and then to write the answer in the form a+ib.

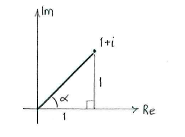
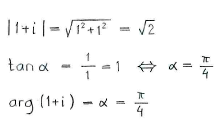
Using the calculations above, we see that

1+i=2cos4+isin4.

De Moivre's formula now gives

(1+i)12=212cos124+isin124=2(12)12cos3+isin3=26(−1+i0)=64(−1)=−64.