Processing Math: Done
To print higher-resolution math symbols, click the
Hi-Res Fonts for Printing button on the jsMath control panel.
jsMath

Lösung 1.3:2b

Aus Online Mathematik Brückenkurs 2

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
Zeile 2: Zeile 2:
# stationäre Punkte, wo <math>f^{\,\prime}(x)=0</math>,
# stationäre Punkte, wo <math>f^{\,\prime}(x)=0</math>,
-
# Singuläre Punkte, wodie Funktion nicht ableitbar ist, oder
+
# Singuläre Punkte, wo die Funktion nicht ableitbar ist, oder
# Endpunkte.
# Endpunkte.

Version vom 16:21, 26. Apr. 2009

Lokale Extrempunkte einer Funktion sind entweder:

  1. stationäre Punkte, wo f(x)=0,
  2. Singuläre Punkte, wo die Funktion nicht ableitbar ist, oder
  3. Endpunkte.

Wir untersuchen alle drei Fälle:

  1. Die Ableitung von f(x) ist
    f(x)=3−2x
    and becomes zero when x=32.


  2. Nachdem die Funktion ein Polynom ist, ist sie überall ableitbar.
  3. Die Funktion ist überall definiert, und also hat unser Intervall keine Endpunkte.

Also sind alle lokalen Extrempunkte auch stationäre Punkte, und also ist x=32 der einziger Punkt der ein Extrempunkt sein könnte. Wir untersuchen ob der Punkt ein Extrempunkt ist, mit einer Vorzeichentabelle.


x 23
f(x) + 0 −
f(x) 417

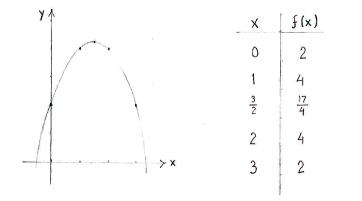
Nachdem die Funktion eine quadratische Funktion ist, ist deren Graph eine Parabel mit den Maxima (32174).

Von „http://wiki.math.se/wikis/2009/bridgecourse2-TU-Berlin/index.php/L%C3%B6sung_1.3:2b“