Processing Math: Done

Aus Online Mathematik Brückenkurs 2

Version vom 10:13, 11. Mär. 2009 (bearbeiten) Tekbot (Diskussion | Beiträge) K (Solution 1.3:6 moved to Lösung 1.3:6: Robot: moved page) ← Zum vorherigen Versionsunterschied		Version vom 12:19, 27. Apr. 2009 (bearbeiten) (rückgängig) Martin (Diskussion | Beiträge) Zum nächsten Versionsunterschied →
Zeile 1:		Zeile 1:
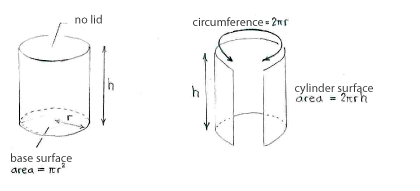
-	If we call the radius of the metal can ''r'' and its height ''h'', then we can determine the can's volume and area by using the figures below,	+	Wir benennen den Radius der Tasse ''r'' und die Höhe ''h''. Der Volumen ist
	{{Abgesetzte Formel||<math>\begin{align}		{{Abgesetzte Formel||<math>\begin{align}
-	\text{Volume} &= \text{(area of the base)}\cdot\text{(height)}\\[5pt]	+	\text{Volume} &= \text{(Fläche der Basis)}\cdot\text{(Höhe)}\\[5pt]
	&= \pi r^2\cdot h\,,\\[10pt]		&= \pi r^2\cdot h\,,\\[10pt]
-	\text{Area} &= \text{(area of the base)} + \text{(area of the cylindrical surface)}\\[5pt]	+	\text{Fläche} &= \text{(Fläche der Basis)} + \text{(Fläche des Zylinders)}\\[5pt]
	&= \pi r^2 + 2\pi rh\,\textrm{.}		&= \pi r^2 + 2\pi rh\,\textrm{.}
	\end{align}</math>}}		\end{align}</math>}}
Zeile 10:		Zeile 10:
	[[Image:1_3_6_1.gif|center]]		[[Image:1_3_6_1.gif|center]]
-	The problem can then be formulated as: minimise the can's area, <math>A = \pi r^2 + 2\pi h</math>, whilst at the same time keeping the volume, <math>V = \pi r^2h\,</math>, constant.	+	Das Problem ist also; Minimiere die Fläche <math>A = \pi r^2 + 2\pi h</math>, während der Volumen <math>V = \pi r^2h\,</math>, konstant ist.
-	From the formula for the volume, we can make ''h'' the subject,	+	Wir schreiben ''h'' als Funktion des Volumen,
	{{Abgesetzte Formel||<math>h=\frac{V}{\pi r^2}</math>}}		{{Abgesetzte Formel||<math>h=\frac{V}{\pi r^2}</math>}}
-	and express the area solely in terms of the radius, ''r'',	+	und können dadurch die Fläche als Funktion von ''r'' schreiben,
	{{Abgesetzte Formel||<math>A = \pi r^2 + 2\pi r\cdot\frac{V}{\pi r^2} = \pi r^2 + \frac{2V}{r}\,\textrm{.}</math>}}		{{Abgesetzte Formel||<math>A = \pi r^2 + 2\pi r\cdot\frac{V}{\pi r^2} = \pi r^2 + \frac{2V}{r}\,\textrm{.}</math>}}
-	The minimisation problem is then:	+	Unser Problem ist dann
-	::Minimise the area <math>A(r) = \pi r^2 + \frac{2V}{r}</math>, when <math>r>0\,</math>.	+	::Minimiere die Flächea <math>A(r) = \pi r^2 + \frac{2V}{r}</math>, wenn <math>r>0\,</math>.
-	The area function <math>A(r)</math> is differentiable for all <math>r>0</math> and the region of definition <math>r>0</math> has no endpoints (<math>r=0</math> does not satisfy <math>r>0</math>), so the function can only assume extreme values at critical points.	+	Die Funktion <math>A(r)</math> ist für alle <math>r>0</math> differenzierbar, und der Bereich <math>r>0</math> hat keine Endpunkte (nachdem <math>r=0</math> nicht <math>r>0</math> erfüllt), und also erscheinen Extrempunkte nur in stationären Punkten.
-	The derivative is given by	+	Die Ableitung ist
	{{Abgesetzte Formel||<math>A'(r) = 2\pi r - \frac{2V}{r^2}\,,</math>}}		{{Abgesetzte Formel||<math>A'(r) = 2\pi r - \frac{2V}{r^2}\,,</math>}}
-	and if we set the derivative equal to zero, so as to obtain the critical points, we get	+	Und die Ableitung gleich null ergibt folgende Gleichung
	{{Abgesetzte Formel||<math>\begin{align}		{{Abgesetzte Formel||<math>\begin{align}
Zeile 37:		Zeile 37:
	\end{align}</math>}}		\end{align}</math>}}
-	For this value of ''r'', the second derivative,	+	Die zweite Ableitung ist
	{{Abgesetzte Formel||<math>A''(r) = 2\pi + \frac{4V}{r^3}\,,</math>}}		{{Abgesetzte Formel||<math>A''(r) = 2\pi + \frac{4V}{r^3}\,,</math>}}
-	has the value	+	und hat den Wert
	{{Abgesetzte Formel||<math>A''\bigl(\sqrt[3]{V/\pi}\bigr) = 2\pi + \frac{4V}{V/\pi } = 6\pi > 0\,,</math>}}		{{Abgesetzte Formel||<math>A''\bigl(\sqrt[3]{V/\pi}\bigr) = 2\pi + \frac{4V}{V/\pi } = 6\pi > 0\,,</math>}}
-	which shows that <math>r=\sqrt[3]{V/\pi}</math> is a local minimum.	+	im stationären Punkt.
-	Because the region of definition, <math>r>0</math>, is open (the endpoint	+	Also ist <math>r=\sqrt[3]{V/\pi}</math> ein lokales Minima.
-	<math>r=0\text{ }</math> is not included) and unlimited, we cannot directly say that the area is least when <math>r = \sqrt[3]{V/\pi}\,</math>; it could be the case that area becomes smaller when <math>r\to 0</math> or <math>r\to \infty </math>. In this case, however, the area increases without bound as	+
-	<math>r\to 0</math> or <math>r\to \infty </math>, so <math>r=\sqrt[3]{V/\pi}</math>	+
-	really is a global minimum.	+
-	The metal can has the least area for a given volume <math>V</math> when	+	Nachdem wir kein begrenztes Intervall haben, können wir nicht direkt ausschließen dass die Fläche kleiner wird wenn <math>r\to 0</math> oder wenn <math>r\to \infty </math>. In unseren Fall wächst die Fläche aber unbegrenzt in den beiden Richtungen <math>r\to 0</math> und <math>r\to \infty </math>, und also ist
		+	<math>r=\sqrt[3]{V/\pi}</math> ein globales Minima.
		+
		+	Also ist die Fläche minimal wenn
	{{Abgesetzte Formel||<math>\begin{align}		{{Abgesetzte Formel||<math>\begin{align}

---

Version vom 12:19, 27. Apr. 2009

Wir benennen den Radius der Tasse r und die Höhe h. Der Volumen ist

VolumeFläche=(Fläche der Basis)(Höhe)=r2h=(Fläche der Basis)+(Fläche des Zylinders)=r2+2rh.

Das Problem ist also; Minimiere die Fläche A=r2+2h, während der Volumen V=r2h, konstant ist.

Wir schreiben h als Funktion des Volumen,

h=Vr2

und können dadurch die Fläche als Funktion von r schreiben,

A=r2+2rVr2=r2+r2V.

Unser Problem ist dann

Minimiere die Flächea A(r)=r2+r2V, wenn r0.

Die Funktion A(r) ist für alle r0 differenzierbar, und der Bereich r0 hat keine Endpunkte (nachdem r=0 nicht r0 erfüllt), und also erscheinen Extrempunkte nur in stationären Punkten.

Die Ableitung ist

A(r)=2r−r22V

Und die Ableitung gleich null ergibt folgende Gleichung

2r−r22V=02r=r22Vr3=Vr=3V.

Die zweite Ableitung ist

A(r)=2+r34V

und hat den Wert

A3V=2+4VV=60

im stationären Punkt.

Also ist r=3V  ein lokales Minima.

Nachdem wir kein begrenztes Intervall haben, können wir nicht direkt ausschließen dass die Fläche kleiner wird wenn r0 oder wenn r. In unseren Fall wächst die Fläche aber unbegrenzt in den beiden Richtungen r0 und r, und also ist

r=3V  ein globales Minima.

Also ist die Fläche minimal wenn

rh=3Vand=Vr2=VV−23=V1−23=V13=3V.