Processing Math: Done
To print higher-resolution math symbols, click the
Hi-Res Fonts for Printing button on the jsMath control panel.
jsMath

Lösung 2.1:1d

Aus Online Mathematik Brückenkurs 2

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
K (Solution 2.1:1d moved to Lösung 2.1:1d: Robot: moved page)
Zeile 1: Zeile 1:
-
The modulus function, <math>|x|</math>, strips <math>x</math> of its sign, e.g.
+
Die Betragsfunktion <math>|x|</math>, returniert den Betrag von <math>x</math>, und nimmt also das Vorzeichen von <math>x</math> weg. Zum Beispiel ist
{{Abgesetzte Formel||<math>|-5|=5\,</math>, <math>\quad|3|=3\quad</math> and <math>\quad |-\pi|=\pi\,</math>.}}
{{Abgesetzte Formel||<math>|-5|=5\,</math>, <math>\quad|3|=3\quad</math> and <math>\quad |-\pi|=\pi\,</math>.}}
-
For positive values of <math>x</math>, the modulus function has no effect, since
+
Für positive <math>x</math>, ändert die Betragsfunktion nichts, nachdem
-
<math>|x|=x</math>, but for negative <math>x</math> the modulus function changes the sign of <math>x</math>, i.e. <math>|x|=-x</math> (remember that <math>x</math>
+
<math>|x|=x</math>, während die Betragsfunktion für negative <math>x</math> das Vorzeichen von <math>x</math>, i.e. <math>|x|=-x</math> ändert.
-
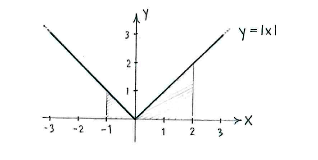
is negative and therefore <math>-x</math> is positive).
+
-
If we draw a graph of <math>y=|x|</math> it will consist of two parts. For
+
Zeichnen wir den Graph von <math>y=|x|</math> besteht er aus zwei Teilen. Für
-
<math>x\ge 0</math> we have <math>y=x</math>, and for <math>x\le 0</math> we have
+
<math>x\ge 0</math>ist <math>y=x</math>, und für <math>x\le 0</math> ist
<math>y=-x\,</math>.
<math>y=-x\,</math>.
[[Image:2_1_1_d1.gif|center]]
[[Image:2_1_1_d1.gif|center]]
-
The value of the integral is the area of the region under the graph <math>y=|x|</math> and between <math>x=-1</math> and <math>x=2</math>.
+
Daher ist das Integral die Fläche unter der Funktion <math>y=|x|</math> zwischen <math>x=-1</math> und <math>x=2</math>.
[[Image:2_1_1_d2.gif|center]]
[[Image:2_1_1_d2.gif|center]]
-
This region consists of two triangles and we therefore obtain
+
Nachdem das Gebiet aus zwei Dreiecken besteht erhalten wir
{{Abgesetzte Formel||<math>\int\limits_{-1}^{2} |x|\,dx = \frac{1}{2}\cdot 1\cdot 1 + \frac{1}{2}\cdot 2\cdot 2 = \frac{5}{2}\,\textrm{.}</math>}}
{{Abgesetzte Formel||<math>\int\limits_{-1}^{2} |x|\,dx = \frac{1}{2}\cdot 1\cdot 1 + \frac{1}{2}\cdot 2\cdot 2 = \frac{5}{2}\,\textrm{.}</math>}}

Version vom 16:30, 28. Apr. 2009

Die Betragsfunktion x, returniert den Betrag von x, und nimmt also das Vorzeichen von x weg. Zum Beispiel ist

−5=5, 3=3 and −=.

Für positive x, ändert die Betragsfunktion nichts, nachdem x=x, während die Betragsfunktion für negative x das Vorzeichen von x, i.e. x=−x ändert.

Zeichnen wir den Graph von y=x besteht er aus zwei Teilen. Für x0ist y=x, und für x0 ist y=−x.

Daher ist das Integral die Fläche unter der Funktion y=x zwischen x=−1 und x=2.

Nachdem das Gebiet aus zwei Dreiecken besteht erhalten wir

2−1xdx=2111+2122=25. 
Von „http://wiki.math.se/wikis/2009/bridgecourse2-TU-Berlin/index.php/L%C3%B6sung_2.1:1d“