Processing Math: 53%
To print higher-resolution math symbols, click the
Hi-Res Fonts for Printing button on the jsMath control panel.
No jsMath TeX fonts found -- using image fonts instead.
These may be slow and might not print well.
Use the jsMath control panel to get additional information.
jsMath Control PanelHide this Message

jsMath

Lösung 3.3:6

Aus Online Mathematik Brückenkurs 2

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
Aktuelle Version (12:52, 3. Sep. 2009) (bearbeiten) (rückgängig)
 
(Der Versionsvergleich bezieht 9 dazwischen liegende Versionen mit ein.)
Zeile 1: Zeile 1:
-
{{NAVCONTENT_START}}
+
'''Polarform'''
-
<center> [[Image:3_3_6-1(5).gif]] </center>
+
-
{{NAVCONTENT_STOP}}
+
-
{{NAVCONTENT_START}}
+
-
<center> [[Image:3_3_6-2(5).gif]] </center>
+
-
{{NAVCONTENT_STOP}}
+
-
{{NAVCONTENT_START}}
+
-
<center> [[Image:3_3_6-3(5).gif]] </center>
+
-
{{NAVCONTENT_STOP}}
+
-
{{NAVCONTENT_START}}
+
-
<center> [[Image:3_3_6-4(5).gif]] </center>
+
-
{{NAVCONTENT_STOP}}
+
-
{{NAVCONTENT_START}}
+
-
<center> [[Image:3_3_6-5(5).gif]] </center>
+
-
{{NAVCONTENT_STOP}}
+
 +
Wir schreiben die Gleichung zuerst in Polarform
 +
 +
{{Abgesetzte Formel||<math>\begin{align}
 +
z &= r\,(\cos\alpha + i\sin\alpha)\,,\\[5pt]
 +
1+i &= \sqrt{2}\Bigl(\cos\frac{\pi}{4} + i\sin\frac{\pi}{4}\Bigr)\,
 +
\end{align}</math>}}
 +
 +
und durch den Moivrischen Satz erhalten wir die Gleichung
 +
 +
{{Abgesetzte Formel||<math>r^2(\cos 2\alpha + i\sin 2\alpha) = \sqrt{2}\Bigl(\cos \frac{\pi}{4} + i\sin\frac{\pi}{4}\Bigr)\,\textrm{.}</math>}}
 +
 +
Damit die beiden Seiten gleich sind, müssen die Beträge der beiden Seiten gleich sein und die Argumente der beiden Seiten dürfen sich nur um ein Vielfaches von <math>2\pi</math> unterscheiden
 +
 +
{{Abgesetzte Formel||<math>\left\{\begin{align}
 +
r^2 &= \sqrt{2}\,,\\[5pt]
 +
2\alpha &= \frac{\pi}{4}+2n\pi\,.\quad
 +
\end{align}\right.</math>}}
 +
 +
Das ergibt
 +
 +
{{Abgesetzte Formel||<math>\left\{\begin{align}
 +
r &= \sqrt{\sqrt{2}} = \bigl(2^{1/2}\bigr)^{1/2} = 2^{1/4} = \sqrt[4]{2}\,,\\[5pt]
 +
\alpha &= \frac{\pi}{8}+n\pi\,.\quad
 +
\end{align}\right.</math>}}
 +
 +
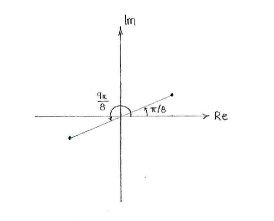
Das entspricht zwei Lösungen, da alle geraden Zahlen dem Argument <math>\pi/8</math> entsprechen, plus ein Vielfaches von <math>2\pi</math> und alle ungerade Zahlen dem Argument <math>9\pi/8</math> entsprechen, plus ein Vielfaches von <math>2\pi</math>.
 +
 +
In Polarform lauten die Lösungen also
 +
 +
{{Abgesetzte Formel||<math>z = \left\{\begin{align}
 +
&\sqrt[4]{2}\Bigl(\cos\frac{\pi}{8} + i\sin\frac{\pi}{8}\Bigr)\,,\\[5pt]
 +
&\sqrt[4]{2}\Bigl(\cos\frac{9\pi}{8} + i\sin\frac{9\pi}{8}\Bigr)\,\textrm{.}
 +
\end{align}\right.</math>}}
 +
 +
 +
Eine Lösung, <math>z=\sqrt[4]{2}(\cos (\pi/8) + i\sin (\pi/8)</math>, liegt im ersten Quadrant und die zweite Lösung, <math>z=\sqrt[4]{2}(\cos (9\pi/8) + i\sin (9\pi/8))</math>, liegt im dritten Quadrant.
[[Image:3_3_6.gif|center]]
[[Image:3_3_6.gif|center]]
 +
 +
 +
''' In der Form ''a'' + ''bi'' '''
 +
 +
Wir schreiben hier <math>z=x+iy</math> und versuchen die Konstanten <math>x</math> und <math>y</math> zu bestimmen.
 +
 +
Mit <math>z=x+iy</math> erhalten wir die Gleichung
 +
 +
{{Abgesetzte Formel||<math>\begin{align}
 +
(x+iy)^2 &= 1+i\,,\\[5pt]
 +
x^2-y^2+2xyi &= 1+i\,\textrm{.}
 +
\end{align}</math>}}
 +
 +
Da der Real- und Imaginärteil der beiden Seiten gleich sein muss, erhalten wir
 +
 +
{{Abgesetzte Formel||<math>\left\{\begin{align}
 +
x^2 - y^2 &= 1\,,\\[5pt]
 +
2xy &= 1\,\textrm{.}
 +
\end{align}\right.</math>}}
 +
 +
Wir können hier <math>x</math> und <math>y</math> direkt bestimmen, aber um es einfacher zu machen, berechnen wir den Betrag von beiden Seiten
 +
 +
{{Abgesetzte Formel||<math>x^2 + y^2 = \sqrt{1^2+1^2} = \sqrt{2}\,\textrm{.}</math>}}
 +
 +
Wir erhalten insgesamt drei Gleichungen
 +
 +
{{Abgesetzte Formel||<math>\left\{\begin{align}
 +
x^2 -y^2 &= 1\,,\\[5pt]
 +
2xy &= 1\,,\\[5pt]
 +
x^2 + y^2 &= \sqrt{2}\,\textrm{.}
 +
\end{align}\right.</math>}}
 +
 +
Addieren wir die erste Gleichung zur dritten erhalten wir
 +
{| align="center" style="padding:10px 0px 10px 0px;"
 +
||
 +
|align="right"|<math>x^2</math>
 +
||<math>{}-{}</math>
 +
|align="right"|<math>y^2</math>
 +
||<math>{}={}</math>
 +
|align="left"|<math>1</math>
 +
|-
 +
|align="left"|<math>+\ \ </math>
 +
|align="right"|<math>x^2</math>
 +
||<math>{}+{}</math>
 +
||<math>y^2</math>
 +
||<math>{}={}</math>
 +
|align="left"|<math>\sqrt{2}</math>
 +
|-
 +
|colspan="6"|<hr>
 +
|-
 +
||
 +
|align="right"|<math>2x^2</math>
 +
||
 +
||
 +
||<math>{}={}</math>
 +
|align="right"|<math>\sqrt{2}+1</math>
 +
|}
 +
 +
und wir erhalten
 +
 +
{{Abgesetzte Formel||<math>x=\pm \sqrt{\frac{\sqrt{2}+1}{2}}\,\textrm{.}</math>}}
 +
 +
Subtrahieren wir die erste Gleichung von der dritten, erhalten wir
 +
{| align="center" style="padding:10px 0px 10px 0px;"
 +
||
 +
|align="right"|<math>x^2</math>
 +
||<math>{}+{}</math>
 +
|align="right"|<math>y^2</math>
 +
||<math>{}={}</math>
 +
|align="left"|<math>\sqrt{2}</math>
 +
|-
 +
|align="left"|<math>-\ \ </math>
 +
|align="right"|<math>\bigl(x^2</math>
 +
||<math>{}-{}</math>
 +
|align="right"|<math>y^2</math>
 +
||<math>{}={}</math>
 +
|align="left"|<math>1\bigr)</math>
 +
|-
 +
|colspan="6"|<hr>
 +
|-
 +
||
 +
||
 +
||
 +
|align="right"|<math>2y^2</math>
 +
||<math>{}={}</math>
 +
|align="right"|<math>\sqrt{2}-1</math>
 +
|}
 +
 +
und das ergibt
 +
 +
{{Abgesetzte Formel||<math>y=\pm \sqrt{\frac{\sqrt{2}-1}{2}}\,\textrm{.}</math>}}
 +
 +
Insgesamt haben wir also vier mögliche Lösungen.
 +
 +
{{Abgesetzte Formel||<math>\left\{\begin{align}
 +
x &= \sqrt{\frac{\sqrt{2}+1}{2}}\\[5pt]
 +
y &= \sqrt{\frac{\sqrt{2}-1}{2}}
 +
\end{align}\right.
 +
\quad
 +
\left\{\begin{align}
 +
x &= \sqrt{\frac{\sqrt{2}+1}{2}}\\[5pt]
 +
y &= -\sqrt{\frac{\sqrt{2}-1}{2}}
 +
\end{align}\right.
 +
\quad
 +
\left\{\begin{align}
 +
x &= -\sqrt{\frac{\sqrt{2}+1}{2}}\\[5pt]
 +
y &= \sqrt{\frac{\sqrt{2}-1}{2}}
 +
\end{align}\right.
 +
\quad
 +
\left\{\begin{align}
 +
x &= -\sqrt{\frac{\sqrt{2}+1}{2}}\\[5pt]
 +
y &= -\sqrt{\frac{\sqrt{2}-1}{2}}
 +
\end{align} \right.</math>}}
 +
 +
Die zweite Gleichung sagt auch, dass <math>xy</math> positiv sein soll. Wir behalten daher nur die Gleichungen.
 +
 +
{{Abgesetzte Formel||<math>\left\{\begin{align}
 +
x &= \sqrt{\frac{\sqrt{2}+1}{2}}\\[5pt]
 +
y &= \sqrt{\frac{\sqrt{2}-1}{2}}
 +
\end{align}\right.
 +
\qquad\text{und}\qquad
 +
\left\{\begin{align}
 +
x &= -\sqrt{\frac{\sqrt{2}+1}{2}}\\[5pt]
 +
y &= -\sqrt{\frac{\sqrt{2}-1}{2}}
 +
\end{align}\right.</math>}}
 +
 +
Nachdem wir wissen, dass unsere Gleichung zwei Lösungen hat, müssen diese unsere Lösungen sein
 +
 +
{{Abgesetzte Formel||<math>z = \left\{\begin{align}
 +
\sqrt{\frac{\sqrt{2}+1}{2}} + i\sqrt{\frac{\sqrt{2}-1}{2}}\,,\\[5pt]
 +
-\sqrt{\frac{\sqrt{2}+1}{2}} - i\sqrt{\frac{\sqrt{2}-1}{2}}\,\textrm{.}
 +
\end{align}\right.</math>}}
 +
 +
Vergleichen wir diese Lösungen mit den Lösungen in Polarform, erhalten wir
 +
 +
{{Abgesetzte Formel||<math>\sqrt[4]{2}\Bigl(\cos\frac{\pi}{8} + i\sin\frac{\pi}{8} \Bigr) = \sqrt{\frac{\sqrt{2}+1}{2}} + i\sqrt{\frac{\sqrt{2}-1}{2}}</math>}}
 +
 +
und daraus folgt
 +
 +
{{Abgesetzte Formel||<math>\begin{align}
 +
\cos\frac{\pi}{8} &= \frac{1}{\sqrt[4]{2}}\sqrt{\frac{\sqrt{2}+1}{2}}\,,\\[5pt]
 +
\sin\frac{\pi}{8} &= \frac{1}{\sqrt[4]{2}}\sqrt{\frac{\sqrt{2}-1}{2}}\,\textrm{.}
 +
\end{align}</math>}}
 +
 +
Wir erhalten auch
 +
 +
{{Abgesetzte Formel||<math>\tan\frac{\pi}{8} = \frac{\sin\dfrac{\pi}{8}}{\cos\dfrac{\pi}{8}} = \frac{\dfrac{1}{\sqrt[4]{2}}\sqrt{\dfrac{\sqrt{2}-1}{2}}}{\dfrac{1}{\sqrt[4]{2}}\sqrt{\dfrac{\sqrt{2}+1}{2}}} = \sqrt {\dfrac{\sqrt{2}-1}{\sqrt{2}+1}}\,\textrm{.}</math>}}
 +
 +
Wir können diesen Ausdruck vereinfachen, indem wir den Ausdruck mit dem konjugierten Nenner erweitern
 +
 +
{{Abgesetzte Formel||<math>\begin{align}
 +
\tan\frac{\pi}{8}
 +
&= \sqrt {\frac{(\sqrt{2}-1)(\sqrt{2}-1)}{(\sqrt{2}+1)(\sqrt{2}-1)}}
 +
= \sqrt {\frac{(\sqrt{2}-1)^2}{(\sqrt{2})^2-1^2}}\\[5pt]
 +
&= \sqrt{\frac{(\sqrt{2}-1)^2}{2-1}}
 +
= \sqrt{(\sqrt{2}-1)^2}
 +
= \sqrt{2}-1\,\textrm{.}
 +
\end{align}</math>}}

Aktuelle Version

Polarform

Wir schreiben die Gleichung zuerst in Polarform

z1+i=r(cos+isin)=2cos4+isin4 

und durch den Moivrischen Satz erhalten wir die Gleichung

r2(cos2+isin2)=2cos4+isin4. 

Damit die beiden Seiten gleich sind, müssen die Beträge der beiden Seiten gleich sein und die Argumente der beiden Seiten dürfen sich nur um ein Vielfaches von 2 unterscheiden

r22=2=4+2n

Das ergibt

r=2=21212=214=42=8+n

Das entspricht zwei Lösungen, da alle geraden Zahlen dem Argument 8 entsprechen, plus ein Vielfaches von 2 und alle ungerade Zahlen dem Argument 98 entsprechen, plus ein Vielfaches von 2.

In Polarform lauten die Lösungen also

z=42cos8+isin842cos89+isin89.


Eine Lösung, z=42(cos(8)+isin(8) , liegt im ersten Quadrant und die zweite Lösung, z=42(cos(98)+isin(98)) , liegt im dritten Quadrant.


In der Form a + bi

Wir schreiben hier z=x+iy und versuchen die Konstanten x und y zu bestimmen.

Mit z=x+iy erhalten wir die Gleichung

(x+iy)2x2−y2+2xyi=1+i=1+i.

Da der Real- und Imaginärteil der beiden Seiten gleich sein muss, erhalten wir

x2−y22xy=1=1. 

Wir können hier x und y direkt bestimmen, aber um es einfacher zu machen, berechnen wir den Betrag von beiden Seiten

x2+y2=12+12=2. 

Wir erhalten insgesamt drei Gleichungen

x2−y22xyx2+y2=1=1=2.

Addieren wir die erste Gleichung zur dritten erhalten wir

x2 − y2 = 1
+   x2 + y2 = \displaystyle \sqrt{2}
\displaystyle 2x^2 \displaystyle {}={} \displaystyle \sqrt{2}+1

und wir erhalten

\displaystyle x=\pm \sqrt{\frac{\sqrt{2}+1}{2}}\,\textrm{.}

Subtrahieren wir die erste Gleichung von der dritten, erhalten wir

\displaystyle x^2 \displaystyle {}+{} \displaystyle y^2 \displaystyle {}={} \displaystyle \sqrt{2}
\displaystyle -\ \ \displaystyle \bigl(x^2 \displaystyle {}-{} \displaystyle y^2 \displaystyle {}={} \displaystyle 1\bigr)
\displaystyle 2y^2 \displaystyle {}={} \displaystyle \sqrt{2}-1

und das ergibt

\displaystyle y=\pm \sqrt{\frac{\sqrt{2}-1}{2}}\,\textrm{.}

Insgesamt haben wir also vier mögliche Lösungen.

\displaystyle \left\{\begin{align}

x &= \sqrt{\frac{\sqrt{2}+1}{2}}\\[5pt] y &= \sqrt{\frac{\sqrt{2}-1}{2}} \end{align}\right. \quad \left\{\begin{align} x &= \sqrt{\frac{\sqrt{2}+1}{2}}\\[5pt] y &= -\sqrt{\frac{\sqrt{2}-1}{2}} \end{align}\right. \quad \left\{\begin{align} x &= -\sqrt{\frac{\sqrt{2}+1}{2}}\\[5pt] y &= \sqrt{\frac{\sqrt{2}-1}{2}} \end{align}\right. \quad \left\{\begin{align} x &= -\sqrt{\frac{\sqrt{2}+1}{2}}\\[5pt] y &= -\sqrt{\frac{\sqrt{2}-1}{2}} \end{align} \right.

Die zweite Gleichung sagt auch, dass \displaystyle xy positiv sein soll. Wir behalten daher nur die Gleichungen.

\displaystyle \left\{\begin{align}

x &= \sqrt{\frac{\sqrt{2}+1}{2}}\\[5pt] y &= \sqrt{\frac{\sqrt{2}-1}{2}} \end{align}\right. \qquad\text{und}\qquad \left\{\begin{align} x &= -\sqrt{\frac{\sqrt{2}+1}{2}}\\[5pt] y &= -\sqrt{\frac{\sqrt{2}-1}{2}} \end{align}\right.

Nachdem wir wissen, dass unsere Gleichung zwei Lösungen hat, müssen diese unsere Lösungen sein

\displaystyle z = \left\{\begin{align}

\sqrt{\frac{\sqrt{2}+1}{2}} + i\sqrt{\frac{\sqrt{2}-1}{2}}\,,\\[5pt] -\sqrt{\frac{\sqrt{2}+1}{2}} - i\sqrt{\frac{\sqrt{2}-1}{2}}\,\textrm{.} \end{align}\right.

Vergleichen wir diese Lösungen mit den Lösungen in Polarform, erhalten wir

\displaystyle \sqrt[4]{2}\Bigl(\cos\frac{\pi}{8} + i\sin\frac{\pi}{8} \Bigr) = \sqrt{\frac{\sqrt{2}+1}{2}} + i\sqrt{\frac{\sqrt{2}-1}{2}}

und daraus folgt

\displaystyle \begin{align}

\cos\frac{\pi}{8} &= \frac{1}{\sqrt[4]{2}}\sqrt{\frac{\sqrt{2}+1}{2}}\,,\\[5pt] \sin\frac{\pi}{8} &= \frac{1}{\sqrt[4]{2}}\sqrt{\frac{\sqrt{2}-1}{2}}\,\textrm{.} \end{align}

Wir erhalten auch

\displaystyle \tan\frac{\pi}{8} = \frac{\sin\dfrac{\pi}{8}}{\cos\dfrac{\pi}{8}} = \frac{\dfrac{1}{\sqrt[4]{2}}\sqrt{\dfrac{\sqrt{2}-1}{2}}}{\dfrac{1}{\sqrt[4]{2}}\sqrt{\dfrac{\sqrt{2}+1}{2}}} = \sqrt {\dfrac{\sqrt{2}-1}{\sqrt{2}+1}}\,\textrm{.}

Wir können diesen Ausdruck vereinfachen, indem wir den Ausdruck mit dem konjugierten Nenner erweitern

\displaystyle \begin{align}

\tan\frac{\pi}{8} &= \sqrt {\frac{(\sqrt{2}-1)(\sqrt{2}-1)}{(\sqrt{2}+1)(\sqrt{2}-1)}} = \sqrt {\frac{(\sqrt{2}-1)^2}{(\sqrt{2})^2-1^2}}\\[5pt] &= \sqrt{\frac{(\sqrt{2}-1)^2}{2-1}} = \sqrt{(\sqrt{2}-1)^2} = \sqrt{2}-1\,\textrm{.} \end{align}

Von „http://wiki.math.se/wikis/2009/bridgecourse2-TU-Berlin/index.php/L%C3%B6sung_3.3:6“