Processing Math: Done

Aus Online Mathematik Brückenkurs 2

Version vom 12:51, 10. Mär. 2009 (bearbeiten) Tekbot (Diskussion | Beiträge) K (Robot: Automated text replacement (-{{Displayed math +{{Abgesetzte Formel)) ← Zum vorherigen Versionsunterschied		Aktuelle Version (10:59, 9. Sep. 2009) (bearbeiten) (rückgängig) Ombtut4 (Diskussion | Beiträge)
(Der Versionsvergleich bezieht 8 dazwischen liegende Versionen mit ein.)
Zeile 1:		Zeile 1:
-	Suppose that the tangent touches the curve at the point <math>(x_0,y_0)</math>. That point must, first and foremost, lie on the curve and therefore satisfy the equation of the curve, i.e.	+	Wir nehmen an, dass die Tangente(n) die Kurve im Punkt <math>(x_0,y_0)</math> berührt. Dieser Punkt liegt natürlich auf der Kurve, erfüllt also
	{{Abgesetzte Formel||<math>y_0 = -x_0^2\,\textrm{.}</math>|(1)}}		{{Abgesetzte Formel||<math>y_0 = -x_0^2\,\textrm{.}</math>|(1)}}
-	If we now write the equation of the tangent as <math>y=kx+m</math>, the slope of the tangent, ''k'', is given by the value of the curve's derivative, <math>y^{\,\prime} = -2x</math>, at <math>x=x_0</math>,	+	Schreiben wir die Tangente als <math>y=kx+m</math>, ist die Steigung ''k'' dasselbe wie die Ableitung <math>y^{\,\prime} = -2x</math> an der Stelle <math>x=x_0</math>.
-	{{Abgesetzte Formel||<math>k = -2x_0\,\textrm{.}</math>|(2)}}	+	{{Abgesetzte Formel||<math>k = -2x_0\,\textrm{}</math>|(2)}}
-	The condition that the tangent goes through the point <math>(x_0,y_0)</math> gives us that	+	Die Bedingung, dass die Tangente durch den Punkt <math>(x_0,y_0)</math> geht, gibt
	{{Abgesetzte Formel||<math>y_{0} = k\cdot x_0 + m\,\textrm{.}</math>|(3)}}		{{Abgesetzte Formel||<math>y_{0} = k\cdot x_0 + m\,\textrm{.}</math>|(3)}}
-	In addition to this, the tangent should also pass through the point (1,1),	+	Die Bedingung dass die Tangente durch den Punkt (1,1) geht, gibt
	{{Abgesetzte Formel||<math>1 = k\cdot 1 + m\,\textrm{.}</math>|(4)}}		{{Abgesetzte Formel||<math>1 = k\cdot 1 + m\,\textrm{.}</math>|(4)}}
-	Equations (1)-(4) constitute a system of equations in the unknowns <math>x_0</math>, <math>y_{0}</math>, <math>k</math> and <math>m</math>.	+	Die Gleichungen (1)-(4) sind ein System von Gleichungen mit den unbekannten <math>x_0</math>, <math>y_{0}</math>, <math>k</math> und <math>m</math>.
-	Because we are looking for <math>x_0</math> and <math>y_0</math>, the first step is to try and eliminate ''k'' and ''m'' from the equations.	+	Da wir <math>x_0</math> und <math>y_0</math> suchen, eliminieren wir zuerst ''k'' und ''m''.
-	Equation (2) gives that <math>k = -2 x_0</math> and substituting this into equation (4) gives	+	Aus der Gleichung (2) folgt, dass <math>k = -2 x_0</math>. Das in Gleichung (4) eingesetzt, liefert
	{{Abgesetzte Formel||<math>1 = -2x_0 + m\quad\Leftrightarrow\quad m = 2x_0+1\,\textrm{.}</math>}}		{{Abgesetzte Formel||<math>1 = -2x_0 + m\quad\Leftrightarrow\quad m = 2x_0+1\,\textrm{.}</math>}}
-	With ''k'' and ''m'' expressed in terms of <math>x_0</math> and <math>y_0</math>, (3) becomes an equation that is expressed completely in terms of <math>x_0</math>	+	Jetzt haben wir ''k'' und ''m'' in Termen von <math>x_0</math> und <math>y_0</math> ausgedrückt und Gleichung (3) hat nun nur <math>x_0</math>-
-	and <math>y_0</math>,	+	und <math>y_0</math>-Terme.
-	{{Abgesetzte Formel||<math>y_0 = -2x_0^2 + 2x_0 + 1\,\textrm{.}</math>|(3')}}	+	{{Abgesetzte Formel||<math>y_0 = -2x_0^2 + 2x_0 + 1\,\textrm{}</math>|(3')}}
-	This equation, together with (1), is a system of equations in <math>x_0</math> and <math>y_0</math>,	+	Diese Gleichung und die Gleichung (1) bilden ein Gleichungssystem für <math>x_0</math> und <math>y_0</math>.
	{{Abgesetzte Formel||<math>\left\{\begin{align}		{{Abgesetzte Formel||<math>\left\{\begin{align}
	y_{0} &= -x_0^{2}\,,\\[5pt]		y_{0} &= -x_0^{2}\,,\\[5pt]
-	y_{0} &= -2x_0^2 + 2x_0 + 1\,\textrm{.}	+	y_{0} &= -2x_0^2 + 2x_0 + 1\,\textrm{}
	\end{align}\right.</math>}}		\end{align}\right.</math>}}
-	Substituting equation (1) into (3') gives us an equation in <math>x_0</math>,	+
		+	Substituieren wir (1) von (3), erhalten wir eine Gleichung mit nur <math>x_0</math>.
	{{Abgesetzte Formel||<math>-x_0^2 = -2x_0^2 + 2x_0 + 1\,,</math>}}		{{Abgesetzte Formel||<math>-x_0^2 = -2x_0^2 + 2x_0 + 1\,,</math>}}
-	i.e.	+	also
	{{Abgesetzte Formel||<math>x_0^2 - 2x_0 - 1 = 0\,\textrm{.}</math>}}		{{Abgesetzte Formel||<math>x_0^2 - 2x_0 - 1 = 0\,\textrm{.}</math>}}
-	This quadratic equation has solutions	+	Diese Quadratische Gleichung hat die Lösungen
-	{{Abgesetzte Formel||<math>x_0 = 1-\sqrt{2}\qquad\text{and}\qquad x_0 = 1+\sqrt{2}\,\textrm{.}</math>}}	+	{{Abgesetzte Formel||<math>x_0 = 1-\sqrt{2}\qquad\text{und}\qquad x_0 = 1+\sqrt{2}\,\textrm{.}</math>}}
-	Equation (1) gives the corresponding y-values,	+	Durch die Gleichung (1) erhalten wir den entsprechenden ''y''-Wert
-	{{Abgesetzte Formel||<math>y_0 = -3+2\sqrt{2}\qquad\text{and}\qquad y_0 = -3-2\sqrt{2}\,\textrm{.}</math>}}	+	{{Abgesetzte Formel||<math>y_0 = -3+2\sqrt{2}\qquad\text{und}\qquad y_0 = -3-2\sqrt{2}\,\textrm{.}</math>}}
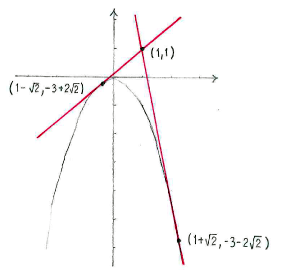
-	Thus, the answers are the points <math>(1-\sqrt{2},-3+2\sqrt{2})</math> and	+	Also erhalten wir die Punkte <math>(1-\sqrt{2},-3+2\sqrt{2})</math> und
	<math>(1+\sqrt{2},-3-2\sqrt{2})\,</math>.		<math>(1+\sqrt{2},-3-2\sqrt{2})\,</math>.
	[[Image:1_1_5_3.gif|center]]		[[Image:1_1_5_3.gif|center]]

---

Aktuelle Version

Wir nehmen an, dass die Tangente(n) die Kurve im Punkt (x0y0) berührt. Dieser Punkt liegt natürlich auf der Kurve, erfüllt also

y0=−x20.

(1)

Schreiben wir die Tangente als y=kx+m, ist die Steigung k dasselbe wie die Ableitung y=−2x an der Stelle x=x0.

k=−2x0

(2)

Die Bedingung, dass die Tangente durch den Punkt (x0y0) geht, gibt

y0=kx0+m.

(3)

Die Bedingung dass die Tangente durch den Punkt (1,1) geht, gibt

1=k1+m.

(4)

Die Gleichungen (1)-(4) sind ein System von Gleichungen mit den unbekannten x0, y0, k und m.

Da wir x0 und y0 suchen, eliminieren wir zuerst k und m.

Aus der Gleichung (2) folgt, dass k=−2x0. Das in Gleichung (4) eingesetzt, liefert

1=−2x0+mm=2x0+1.

Jetzt haben wir k und m in Termen von x0 und y0 ausgedrückt und Gleichung (3) hat nun nur x0- und y0-Terme.

y0=−2x20+2x0+1

(3')

Diese Gleichung und die Gleichung (1) bilden ein Gleichungssystem für x0 und y0.

y0y0=−x20=−2x20+2x0+1

Substituieren wir (1) von (3), erhalten wir eine Gleichung mit nur x0.

−x20=−2x20+2x0+1

also

x20−2x0−1=0.

Diese Quadratische Gleichung hat die Lösungen

x0=1−2undx0=1+2.

Durch die Gleichung (1) erhalten wir den entsprechenden y-Wert

y0=−3+22undy0=−3−22.

Also erhalten wir die Punkte (1−2−3+22)  und (1+2−3−22) .