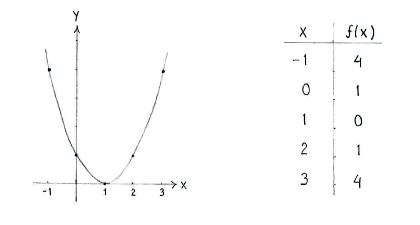Processing Math: Done
To print higher-resolution math symbols, click the
Hi-Res Fonts for Printing button on the jsMath control panel.
No jsMath TeX fonts found -- using image fonts instead.
These may be slow and might not print well.
Use the jsMath control panel to get additional information.
jsMath Control PanelHide this Message

jsMath

Lösung 1.3:2a

Aus Online Mathematik Brückenkurs 2

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
Aktuelle Version (17:44, 9. Sep. 2009) (bearbeiten) (rückgängig)
 
(Der Versionsvergleich bezieht 8 dazwischen liegende Versionen mit ein.)
Zeile 1: Zeile 1:
-
A function has its local extreme points at the following types of points:
+
Lokale Extremstellen einer Funktion sind entweder:
-
1. critical points, i.e. where
+
# stationäre Stellen mit <math>f^{\,\prime}(x)=0</math>,
-
<math>{f}'\left( x \right)=0</math>;
+
# singuläre Stellen (Theorie 1.3 E), in denen die Funktion nicht ableitbar ist, oder
-
 
+
# Randstellen.
-
2. points where the function is not differentiable;
+
-
 
+
-
3. endpoints of the interval of definition.
+
-
We investigate these cases for our function:
+
Wir untersuchen alle drei Fälle.
-
 
+
-
1. The derivative of
+
-
<math>f\left( x \right)</math>
+
-
is given by
+
-
+
-
<math>{f}'\left( x \right)=2x-2</math>
+
-
 
+
-
and becomes zero when
+
-
<math>2x-2=0</math>, i.e
+
-
<math>x=1</math>.
+
-
 
+
-
2. Because the function is a polynomial, it is differentiable everywhere.
+
-
 
+
-
3. The function is defined regardless of the value of
+
-
<math>x</math>, which means that the interval of definition is the whole real axis, and there are therefore no endpoints.
+
-
The only point where the function could possibly have a local extreme point is thus x=1. In order to determine whether the point is a local extreme point, we investigate the derivative using a sign table.
+
<ol>
 +
<li>Die Ableitung von <math>f(x)</math>
 +
{{Abgesetzte Formel||<math>f^{\,\prime}(x) = 2x-2</math>}}
 +
ist null, wenn <math>2x-2=0</math>, also für <math>x=1\,</math>.</li>
-
TABELL1
+
<li>Da die Funktion ein Polynom ist, ist sie überall differenzierbar.</li>
-
Because the derivative is negative to the left of
+
<li>Die Funktion ist überall definiert, also hat unser Intervall keinen Rand und es gibt keine Randstellen.</li>
-
<math>x=1</math>
+
</ol>
-
and positive to the right of
+
-
<math>x=1</math>, then
+
-
<math>x=1</math>
+
-
is a local minimum.
+
-
The sign table also gives information about the graph's approximate appearance and by calculating the value of the function at a couple of points, we can make a sketch of the graph.
+
Also sind alle lokalen Extremstellen auch stationäre Stellen und so ist <math>x=1\,</math> die einzige Stelle, die eine Extremstelle sein kann. Mit einer Vorzeichentabelle untersuchen wir, ob die Stelle eine Extremstelle ist.
-
PICTURE TABELL2
+
{| border="1" cellpadding="5" cellspacing="0" align="center"
 +
|-
 +
|width="50px" align="center" style="background:#efefef;"| <math>x</math>
 +
|style="background:#efefef;"|
 +
|width="50px" align="center" style="background:#efefef;"| <math>1</math>
 +
|style="background:#efefef;"|
 +
|-
 +
|width="50px" align="center"| <math>f^{\,\prime}(x)</math>
 +
|width="50px" align="center"| <math>-</math>
 +
|width="50px" align="center"| <math>0</math>
 +
|width="50px" align="center"| <math>+</math>
 +
|-
 +
|width="50px" align="center"| <math>f(x)</math>
 +
|width="50px" align="center"| <math>\searrow</math>
 +
|width="50px" align="center"| <math>0</math>
 +
|width="50px" align="center"| <math>\nearrow</math>
 +
|}
 +
Da die Ableitung links von <math>x=1</math> negativ ist und rechts von <math>x=1</math> positiv, ist <math>x=1</math> eine lokale Minimalstelle.
 +
Berechnen wir zusätzlich den Funktionswert in einigen Punkten, können wir die Funktion zeichnen.
[[Image:1_3_2a-3(3).gif|center]]
[[Image:1_3_2a-3(3).gif|center]]

Aktuelle Version

Lokale Extremstellen einer Funktion sind entweder:

  1. stationäre Stellen mit f(x)=0,
  2. singuläre Stellen (Theorie 1.3 E), in denen die Funktion nicht ableitbar ist, oder
  3. Randstellen.

Wir untersuchen alle drei Fälle.

  1. Die Ableitung von f(x)
    f(x)=2x−2
    ist null, wenn 2x−2=0, also für x=1.
  2. Da die Funktion ein Polynom ist, ist sie überall differenzierbar.
  3. Die Funktion ist überall definiert, also hat unser Intervall keinen Rand und es gibt keine Randstellen.

Also sind alle lokalen Extremstellen auch stationäre Stellen und so ist x=1 die einzige Stelle, die eine Extremstelle sein kann. Mit einer Vorzeichentabelle untersuchen wir, ob die Stelle eine Extremstelle ist.

x 1
f(x) − 0 +
f(x) 0

Da die Ableitung links von x=1 negativ ist und rechts von x=1 positiv, ist x=1 eine lokale Minimalstelle.

Berechnen wir zusätzlich den Funktionswert in einigen Punkten, können wir die Funktion zeichnen.

Von „http://wiki.math.se/wikis/2009/bridgecourse2-TU-Berlin/index.php/L%C3%B6sung_1.3:2a“