3.3 Potenzen und Wurzeln
Aus Online Mathematik Brückenkurs 2
K (Robot: Automated text replacement (-{{Vald flik +{{Selected tab)) |
|||
| (Der Versionsvergleich bezieht 47 dazwischen liegende Versionen mit ein.) | |||
| Zeile 2: | Zeile 2: | ||
{| border="0" cellspacing="0" cellpadding="0" height="30" width="100%" | {| border="0" cellspacing="0" cellpadding="0" height="30" width="100%" | ||
| style="border-bottom:1px solid #797979" width="5px" | | | style="border-bottom:1px solid #797979" width="5px" | | ||
| - | {{ | + | {{Gewählter Tab|[[3.3 Potenzen und Wurzeln|Theorie]]}} |
| - | {{ | + | {{Nicht gewählter Tab|[[3.3 Übungen|Übungen]]}} |
| style="border-bottom:1px solid #797979" width="100%"| | | style="border-bottom:1px solid #797979" width="100%"| | ||
|} | |} | ||
{{Info| | {{Info| | ||
| - | ''' | + | '''Inhalt:''' |
| - | * | + | * Der Moivresche Satz |
| - | * | + | * Quadratische Gleichungen |
| - | + | * Exponentialfunktionen | |
| - | + | * Quadratische Ergänzung | |
| - | * | + | |
| - | * | + | |
}} | }} | ||
{{Info| | {{Info| | ||
| - | ''' | + | '''Lernziele:''' |
| - | + | Nach diesem Abschnitt solltest Du folgendes wissen: | |
| - | * | + | * Wie man Potenzen von komplexen Zahlen mit dem Moivreschen Satz löst. |
| - | * | + | * Wie man Wurzeln von komplexen Zahlen berechnet, indem man die Zahl in Polarform bringt. |
| - | * | + | * Wie man komplexe quadratische Ausdrücke quadratisch ergänzt. |
| - | * | + | * Wie man komplexe quadratische Gleichungen löst. |
| - | + | ||
}} | }} | ||
| - | + | Die Lernziele sind Dir aus der Schule noch bestens vertraut und Du weißt ganz genau, wie man die zugehörigen Rechnungen ausführt? Dann kannst Du auch gleich mit den <b>Prüfungen</b> beginnen (Du findest den Link in der Student Lounge). | |
| - | + | == A - Moivrescher Satz== | |
| - | + | Die Rechenregeln <math>\ \arg (zw) = \arg z + \arg w\ </math> und <math>\ |\,zw\,| = |\,z\,|\,|\,w\,|\ </math> bedeuten, dass | |
| - | + | {{Abgesetzte Formel||<math>\biggl\{\begin{align*}&\arg (z\cdot z) = \arg z + \arg z \\ &|\,z\cdot z\,| = |\,z\,|\cdot|\,z\,|\end{align*}\qquad\biggl\{\begin{align*}&\arg z^3 = 3 \arg z \cr &|\,z^3\,| = |\,z\,|^3\end{align*}\qquad\text{etc.}</math>}} | |
| - | {{ | + | |
| + | Für eine beliebige komplexe Zahl <math>z=r\,(\cos \alpha +i\,\sin \alpha)</math> gilt daher, dass | ||
| + | |||
| + | {{Abgesetzte Formel||<math>z^n = \bigl(r\,(\cos \alpha +i\sin \alpha)\bigr)^n = r^n\,(\cos n\alpha +i\,\sin n\alpha)\,\mbox{.}</math>}} | ||
| - | + | Falls <math>|\,z\,|=1</math> (also, dass <math>z</math> am Einheitskreis liegt), erhalten wir den Sonderfall | |
<div class="regel"> | <div class="regel"> | ||
| - | {{ | + | {{Abgesetzte Formel||<math>(\cos \alpha +i\,\sin \alpha)^n = \cos n\alpha +i\,\sin n\alpha\,\mbox{.}</math>}} |
</div> | </div> | ||
| - | + | Diese Regel nennt man den ''Moivreschen Satz''. Wie wir sehen werden, ist diese Regel sehr wichtig, wenn man Potenzen und Wurzeln von komplexen Zahlen berechnet. | |
<div class="exempel"> | <div class="exempel"> | ||
| - | ''' | + | ''' Beispiel 1''' |
| - | + | Bestimme <math>z^3</math> und <math>z^{100}</math> für <math>z = \frac{1+i}{\sqrt2}</math> . | |
| - | + | Wir schreiben <math>z</math> in Polarform <math>\ \ z= \frac{1}{\sqrt2} + \frac{i}{\sqrt2} = 1\cdot \Bigl(\cos \frac{\pi}{4} + i\sin \frac{\pi}{4}\Bigr)\ \ </math> und verwenden den Moivreschen Satz | |
| - | {{ | + | {{Abgesetzte Formel||<math>\begin{align*}z^3 &= \Bigl( \cos\frac{\pi}{4} + i\,\sin\frac{\pi}{4}\,\Bigr)^3 = \cos\frac{3\pi}{4} + i\,\sin\frac{3\pi}{4} = -\frac{1}{\sqrt2} + \frac{1}{\sqrt2}\,i = \frac{-1+i}{\sqrt2}\,\mbox{}\\[6pt] z^{100} &= \Bigl( \cos\frac{\pi}{4} + i\,\sin\frac{\pi}{4}\,\Bigr)^{100} = \cos\frac{100\pi}{4} + i\,\sin\frac{100\pi}{4}\\[4pt] &= \cos 25\pi + i\,\sin 25\pi = \cos \pi + i\,\sin \pi = -1\,\mbox{.}\end{align*}</math>}} |
</div> | </div> | ||
<div class="exempel"> | <div class="exempel"> | ||
| - | ''' | + | ''' Beispiel 2''' |
| + | Mit der binomischen Formel können wir den Ausdruck wie folgt erläutern: | ||
| - | + | {{Abgesetzte Formel||<math>\begin{align*} (\cos v + i\,\sin v)^2 &= \cos^2\!v + i^2 \sin^2\!v + 2i \sin v \cos v\\ &= \cos^2\!v - \sin^2\!v + 2i \sin v \cos v\,\mbox{,}\end{align*}</math>}} | |
| - | + | aber wir können auch den Moivreschen Satz benutzen. Dann erhalten wir: | |
| - | + | {{Abgesetzte Formel||<math>(\cos v + i \sin v)^2 = \cos 2v + i \sin 2v\,\mbox{.}</math>}} | |
| - | + | Da die beiden Ausdrücke gleich sind, erhalten wir, indem wir die Real- und Imaginärteile gleichsetzen, die bekannten trigonometrischen Identitäten | |
| - | If one equates the real and imaginary parts of the two expressions one gets the well-known trigonometric formulas | ||
| - | + | {{Abgesetzte Formel||<math>\biggl\{\begin{align*}\cos 2v &= \cos^2\!v - \sin^2\!v\,\mbox{,}\\[2pt] \sin 2v&= 2 \sin v \cos v\,\mbox{.}\end{align*}</math>}} | |
| - | {{ | + | |
</div> | </div> | ||
<div class="exempel"> | <div class="exempel"> | ||
| - | ''' | + | ''' Beispiel 3''' |
| - | + | ||
| - | Simplify <math>\ \ \frac{(\sqrt3 + i)^{14}}{(1+i\sqrt3\,)^7(1+i)^{10}}\,</math>. | ||
| + | Vereinfache <math>\ \ \frac{(\sqrt3 + i)^{14}}{(1+i\sqrt3\,)^7(1+i)^{10}}\,</math>. | ||
| - | + | Wir schreiben die Zahlen <math>\sqrt{3}+i</math>, <math>1+i\sqrt{3}</math> und <math>1+i</math> in Polarform | |
*<math>\quad\sqrt{3} + i = 2\Bigl(\cos\frac{\pi}{6} + i\,\sin\frac{\pi}{6}\,\Bigr)\vphantom{\biggl(}</math>, | *<math>\quad\sqrt{3} + i = 2\Bigl(\cos\frac{\pi}{6} + i\,\sin\frac{\pi}{6}\,\Bigr)\vphantom{\biggl(}</math>, | ||
*<math>\quad 1+i\sqrt{3} = 2\Bigl(\cos\frac{\pi}{3} + i\,\sin\frac{\pi}{3}\,\Bigr)\vphantom{\biggl(}</math>, | *<math>\quad 1+i\sqrt{3} = 2\Bigl(\cos\frac{\pi}{3} + i\,\sin\frac{\pi}{3}\,\Bigr)\vphantom{\biggl(}</math>, | ||
*<math>\quad 1+i = \sqrt2\,\Bigl(\cos\frac{\pi}{4} + i\,\sin\frac{\pi}{4}\,\Bigr)\vphantom{\biggl(}</math>. | *<math>\quad 1+i = \sqrt2\,\Bigl(\cos\frac{\pi}{4} + i\,\sin\frac{\pi}{4}\,\Bigr)\vphantom{\biggl(}</math>. | ||
| - | + | Nach dem Moivreschen Satz erhalten wir | |
| - | {{ | + | {{Abgesetzte Formel||<math>\frac{(\sqrt3 + i)^{14}}{(1+i\sqrt3\,)^7(1+i)^{10}} = \frac{\displaystyle 2^{14}\Bigl(\cos\frac{14\pi}{6} + i\,\sin \frac{14\pi}{6}\,\Bigr)\vphantom{\biggl(}}{\displaystyle 2^7\Bigl(\cos \frac{7\pi}{3} + i\,\sin\frac{7\pi}{3}\,\Bigr) \, (\sqrt{2}\,)^{10}\Bigl(\cos\frac{10\pi}{4} + i\,\sin\frac{10\pi}{4}\,\Bigr)\vphantom{\biggl(}}\:</math>.}} |
| - | + | Diesen Ausdruck können wir weiter vereinfachen, indem wir die Multiplikations- und Divisionsregeln für komplexe Zahlen in Polarform verwenden | |
| - | {{ | + | {{Abgesetzte Formel||<math>\begin{align*}\frac{\displaystyle 2^{14}\Bigl(\cos\frac{14\pi}{6} + i\,\sin\frac{14\pi}{6}\,\Bigr)\vphantom{\biggl(}} {\displaystyle 2^{12}\Bigl(\cos\frac{29\pi}{6} + i\,\sin\frac{29\pi}{6}\,\Bigr)\vphantom{\biggl(}} &= 2^2 \Bigl(\cos\Bigl( -\frac{15\pi}{6}\,\Bigr) + i\,\sin\Bigl( -\frac{15\pi}{6}\,\Bigr)\,\Bigr)\\[8pt] &= 4\Bigl(\cos \Bigl( -\frac{\pi}{2}\,\Bigr) + i\,\sin\Bigl( -\frac{\pi}{2}\,\Bigr)\,\Bigr) = -4i\,\mbox{.}\end{align*}</math>}} |
</div> | </div> | ||
| - | == | + | == B - ''n''te Wurzeln von komplexen Zahlen == |
| - | + | Eine komplexe Zahl <math>z</math> wird ''n''te Wurzel von <math>w</math> genannt, falls | |
<div class="regel"> | <div class="regel"> | ||
| - | {{ | + | {{Abgesetzte Formel||<math>z^n= w \mbox{.}</math>}} |
</div> | </div> | ||
| - | + | ||
| - | + | Lösungen dieser Wurzelgleichung erhält man, indem man beide Zahlen in Polarform bringt und deren Betrag und Argument vergleicht. | |
| + | |||
| + | Ist eine Zahl <math> w=|\,w\,|\,(\cos \theta + i\,\sin \theta) </math> gegeben, nimmt man an, dass <math>z=r\,(\cos \alpha + i\, \sin \alpha)</math> und erhält so die Gleichung | ||
| + | {{Abgesetzte Formel||<math>r^{\,n}\,(\cos n\alpha + i \sin n\alpha) =|w|\,(\cos \theta + i \sin \theta)\,\mbox{,}</math>}} | ||
| - | + | wo wir den Moivreschen Satz auf der linken Seite angewendet haben. Vergleichen wir das Argument und den Betrag der beiden Seiten, erhalten wir | |
| - | + | ||
| - | + | {{Abgesetzte Formel||<math>\biggl\{\begin{align*} r^{\,n} &= |w|\,\mbox{,}\\ n\alpha &= \theta + k\cdot 2\pi\,\mbox{.}\end{align*}</math>}} | |
| - | + | Beachte hier, dass wir ein Vielfaches von <math>2\pi</math> zum Argument addiert haben, um alle Lösungen zu erhalten. | |
| - | + | {{Abgesetzte Formel||<math>\biggl\{\begin{align*} r &={\textstyle\sqrt[\scriptstyle n]{|w|}},\\ \alpha &= (\theta + 2k\pi)/n\,, \quad k=0, \pm 1, \pm 2, \ldots\end{align*}</math>}} | |
| - | + | Wir erhalten also ''einen'' Wert für <math>r</math>, aber unendlich viele Werte für <math>\alpha</math>. Trotzdem gibt es nicht unendlich viele Lösungen dieser Gleichung. Für Werte von <math>k</math> zwischen <math>k = 0</math> und <math>k = n - 1</math> erhalten wir verschiedene Argumente für <math>z</math> und daher verschiedene Zahlen <math>z</math>. Für andere Werte von <math>k</math> wiederholen wir nur die schon bekannten Lösungen, da die Funktionen <math>\cos \theta</math> und <math>\sin \theta</math> periodisch sind und die Periodenlänge <math>2 \pi</math> haben. Also hat eine Gleichung mit der Form <math>z^n=w</math> genau <math>n</math> Wurzeln. | |
| - | + | Hinweis: Beachte, dass die Argumente der Lösungen sich immer um <math>2\pi/n</math> unterscheiden. Also sind die Lösungen gleichförmig auf dem Kreis mit dem Radius <math>\sqrt[\scriptstyle n]{|w|}</math> verteilt und bilden ein ''n''-seitiges Polygon. | |
| - | ''Comment''. Note that the arguments of the roots differ from each other by <math>2\pi/n</math> so that the roots are evenly distributed on a circle with radius <math>\sqrt[\scriptstyle n]{|w|}</math> and form corners in a regular ''n-gon'' (an ''n'' sided polygon). | ||
<div class="exempel"> | <div class="exempel"> | ||
| - | ''' | + | '''Beispiel 4''' |
| - | + | Löse die Gleichung <math>\ z^4= 16\,i\,</math>. | |
| - | + | Wir schreiben <math>z</math> und <math>16\,i</math> in Polarform | |
*<math>\quad z=r\,(\cos \alpha + i\,\sin \alpha)\,</math>, | *<math>\quad z=r\,(\cos \alpha + i\,\sin \alpha)\,</math>, | ||
*<math>\quad 16\,i= 16\Bigl(\cos\frac{\pi}{2} + i\,\sin\frac{\pi}{2}\,\Bigr)\vphantom{\biggl(}</math>. | *<math>\quad 16\,i= 16\Bigl(\cos\frac{\pi}{2} + i\,\sin\frac{\pi}{2}\,\Bigr)\vphantom{\biggl(}</math>. | ||
| - | + | Die Gleichung <math>\ z^4=16\,i\ </math> wird also | |
| - | {{ | + | {{Abgesetzte Formel||<math>r^4\,(\cos 4\alpha + i\,\sin 4\alpha) = 16\Bigl(\cos\frac{\pi}{2} + i\sin\frac{\pi}{2}\,\Bigr)\,\mbox{.}</math>}} |
| - | + | Vergleichen wir das Argument und den Betrag der beiden Seiten, erhalten wir | |
| - | {{ | + | {{Abgesetzte Formel||<math>\biggl\{\begin{align*} r^4 &= 16,\\ 4\alpha &= \pi/2 + k\cdot 2\pi,\end{align*}\qquad\text{d.h.}\qquad\biggl\{\begin{align*} r &= \sqrt[\scriptstyle 4]{16}= 2, \\ \alpha &= \pi/8 + k\pi/2\,,\quad k=0,1,2,3.\end{align*}</math>}} |
{| width="100%" | {| width="100%" | ||
| width="95%"| | | width="95%"| | ||
| - | + | Die Wurzeln der Gleichung sind daher | |
| - | {{ | + | {{Abgesetzte Formel||<math>\left\{\begin{align*}\displaystyle z_1&= 2\Bigl(\cos \frac{\pi}{8} + i\,\sin\frac{\pi}{8}\,\Bigr),\\[4pt] |
\displaystyle z_2 &= 2\Bigl(\cos\frac{5\pi}{8} + i\,\sin\frac{5\pi}{8}\,\Bigr),\vphantom{\biggl(}\\[4pt] | \displaystyle z_2 &= 2\Bigl(\cos\frac{5\pi}{8} + i\,\sin\frac{5\pi}{8}\,\Bigr),\vphantom{\biggl(}\\[4pt] | ||
\displaystyle z_3 &= 2\Bigl(\cos\frac{9\pi}{8} + i\,\sin\frac{9\pi}{8}\,\Bigr),\vphantom{\biggl(}\\[4pt] | \displaystyle z_3 &= 2\Bigl(\cos\frac{9\pi}{8} + i\,\sin\frac{9\pi}{8}\,\Bigr),\vphantom{\biggl(}\\[4pt] | ||
\displaystyle z_4 &= 2\Bigl(\cos\frac{13\pi}{8} + i\,\sin\frac{13\pi}{8}\,\Bigr).\end{align*}\right.</math>}} | \displaystyle z_4 &= 2\Bigl(\cos\frac{13\pi}{8} + i\,\sin\frac{13\pi}{8}\,\Bigr).\end{align*}\right.</math>}} | ||
| width="5%" | | | width="5%" | | ||
| - | ||{{:3.3 - | + | ||{{:3.3 - Bild - Die komplexen Zahlen z₁, z₂, z₃ und z₄}} |
|} | |} | ||
</div> | </div> | ||
| - | == | + | == C - Exponentialform der komplexen Zahlen== |
| - | If we manipulate <math>i</math> as if it were a real number and treat a complex number <math>z</math> as a function of just <math>\alpha</math> ( where <math>r</math> is a constant), | ||
| - | + | Wenn wir <math>i</math> als eine normale Zahl betrachten und die komplexe Zahl <math>z</math> wie eine Funktion von nur <math>\alpha</math> betrachten (in der <math>r</math> also konstant ist), ergibt sich | |
| - | + | {{Abgesetzte Formel||<math>f(\alpha) = r\,(\cos \alpha + i\,\sin \alpha)</math>}} | |
| - | + | und wir erhalten durch wiederholte Ableitung | |
| - | + | {{Abgesetzte Formel||<math>\begin{align*} f^{\,\prime}(\alpha) &= -r\sin \alpha + r\,i\,\cos \alpha = r\,i^2 \sin \alpha + r\,i\,\cos \alpha = i\,r\,(\cos \alpha + i\,\sin \alpha) = i\,f(\alpha)\\ f^{\,\prime\prime} (\alpha) &= - r\,\cos \alpha - r\,i\,\sin \alpha = i^2\,r\,(\cos \alpha + i\,\sin \alpha) = i^2\, f(\alpha)\cr &\text{etc.}\end{align*}</math>}} | |
| - | + | Die einzigen reellen Funktionen, die dies erfüllen, sind Funktionen in der Form <math>f(x)= e^{\,kx}</math>. Daher stammt folgende Definition: | |
| - | + | ||
| - | + | ||
| - | {{ | + | {{Abgesetzte Formel||<math>e^{\,i\alpha} = \cos \alpha + i\,\sin \alpha\,\mbox{.}</math>}} |
| + | |||
| + | Dies ist auch eine Verallgemeinerung der reellen Exponentialfunktion für komplexe Zahlen. Ersetzen wir <math>z=a+ib</math> erhalten wir | ||
| + | |||
| + | {{Abgesetzte Formel||<math>e^{\,z} = e^{\,a+ib} = e^{\,a} \, e^{\,ib} = e^{\,a}(\cos b + i\,\sin b)\,\mbox{.}</math>}} | ||
| - | + | Die Definition von <math>e^{\,z}</math> kann wie eine Kurzform der Polarform verwendet werden, da <math>z=r\,(\cos \alpha + i\,\sin \alpha) = r\,e^{\,i\alpha}\,</math>. | |
<div class="exempel"> | <div class="exempel"> | ||
| - | ''' | + | ''' Beispiel 5''' |
| + | Für eine reelle Zahl <math>z</math> ist die Definition dieselbe wie für die reelle Exponentialfunktion. Da <math>z=a+0\cdot i</math> erhalten wir | ||
| - | + | {{Abgesetzte Formel||<math>e^{\,z} = e^{\,a+0\cdot i} = e^a (\cos 0 + i \sin 0) = e^a \cdot 1 = e^a\,\mbox{.}</math>}} | |
| - | + | ||
| - | {{ | + | |
</div> | </div> | ||
<div class="exempel"> | <div class="exempel"> | ||
| - | ''' | + | ''' Beispiel 6''' |
| - | + | ||
| - | + | Eine weitere Folgerung aus dieser Definition erhalten wir durch den Moivreschen Satz. | |
| - | {{ | + | {{Abgesetzte Formel||<math>\bigl(e^{\,i\alpha}\bigr)^n = (\cos \alpha + i \sin \alpha)^n = \cos n\alpha + i \sin n \alpha = e^{\,in\alpha}</math>}} |
| - | + | Das erinnert uns an die wohlbekannte Rechenregel für Potenzen. | |
| - | {{ | + | {{Abgesetzte Formel||<math>\left(a^x\right)^y = a^{x\,y}</math>}} |
</div> | </div> | ||
<div class="exempel"> | <div class="exempel"> | ||
| - | ''' | + | ''' Beispiel 7''' |
| - | + | Mit den Definitionen oben erhalten wir die Formel | |
| - | {{ | + | {{Abgesetzte Formel||<math>e^{\pi\,i} = \cos \pi + i \sin \pi = -1</math>}} |
| - | + | Diese berühmte Formel wurde von Euler zu Beginn des 18. Jahrhunderts entdeckt. | |
| - | + | ||
</div> | </div> | ||
<div class="exempel"> | <div class="exempel"> | ||
| - | ''' | + | ''' Beispiel 8''' |
| + | Löse die Gleichung <math>\ (z+i)^3 = -8i</math>. | ||
| - | Solve the equation <math>\ (z+i)^3 = -8i</math>. | ||
| - | + | Wir lassen <math>w = z + i</math> sein. Wir erhalten so die Gleichung <math>\ w^3=-8i\,</math>. Wir bringen als ersten Schritt <math>w</math> und <math>-8i</math> in Polarform | |
| - | + | ||
*<math>\quad w=r\,(\cos \alpha + i\,\sin \alpha) = r\,e^{i\alpha}\,\mbox{,}</math> | *<math>\quad w=r\,(\cos \alpha + i\,\sin \alpha) = r\,e^{i\alpha}\,\mbox{,}</math> | ||
*<math>\quad -8i = 8\Bigl(\cos \frac{3\pi}{2} + i\,\sin\frac{3\pi}{2}\,\Bigr) = 8\,e^{3\pi i/2}\vphantom{\biggl(}\,\mbox{.}</math> | *<math>\quad -8i = 8\Bigl(\cos \frac{3\pi}{2} + i\,\sin\frac{3\pi}{2}\,\Bigr) = 8\,e^{3\pi i/2}\vphantom{\biggl(}\,\mbox{.}</math> | ||
| - | The equation in polar form is <math>\ r^3e^{3\alpha i}=8\,e^{3\pi i/2}\ </math> and matching the moduli and arguments on both sides gives, | ||
| - | {{ | + | In Polarform lautet die Gleichung <math>\ r^3e^{3\alpha i}=8\,e^{3\pi i/2}\ </math>. Vergleichen wir das Argument und den Betrag der rechten und linken Seite, erhalten wir |
| + | |||
| + | {{Abgesetzte Formel||<math>\biggl\{\begin{align*} r^3 &= 8\,\mbox{,}\\ 3\alpha &= 3\pi/2+2k\pi\,\mbox{,}\end{align*}\qquad\Leftrightarrow\qquad\biggl\{\begin{align*} r&=\sqrt[\scriptstyle 3]{8}\,\mbox{,}\\ \alpha&= \pi/2+2k\pi/3\,,\quad k=0,1,2\,\mbox{.}\end{align*}</math>}} | ||
| - | + | Die Wurzeln der Gleichung sind daher | |
*<math>\quad w_1 = 2\,e^{\pi i/2} = 2\Bigl(\cos \frac{\pi}{2} + i\,\sin\frac{\pi}{2}\,\Bigr) = 2i\,\mbox{,}\quad\vphantom{\biggl(}</math> | *<math>\quad w_1 = 2\,e^{\pi i/2} = 2\Bigl(\cos \frac{\pi}{2} + i\,\sin\frac{\pi}{2}\,\Bigr) = 2i\,\mbox{,}\quad\vphantom{\biggl(}</math> | ||
*<math>\quad w_2 = 2\,e^{7\pi i/6} = 2\Bigl(\cos\frac{7\pi}{6} + i\,\sin\frac{7\pi}{6}\,\Bigr) = -\sqrt{3}-i\,\mbox{,}\quad\vphantom{\Biggl(}</math> | *<math>\quad w_2 = 2\,e^{7\pi i/6} = 2\Bigl(\cos\frac{7\pi}{6} + i\,\sin\frac{7\pi}{6}\,\Bigr) = -\sqrt{3}-i\,\mbox{,}\quad\vphantom{\Biggl(}</math> | ||
*<math>\quad w_3 = 2\,e^{11\pi i/6} = 2\Bigl(\cos\frac{11\pi}{6} + i\,\sin\frac{11\pi}{6}\,\Bigr) = \sqrt{3}-i\,\mbox{,}\quad\vphantom{\biggl(}</math> | *<math>\quad w_3 = 2\,e^{11\pi i/6} = 2\Bigl(\cos\frac{11\pi}{6} + i\,\sin\frac{11\pi}{6}\,\Bigr) = \sqrt{3}-i\,\mbox{,}\quad\vphantom{\biggl(}</math> | ||
| - | + | also sind <math>z_1 = 2i-i=i</math>, <math>z_2 = - \sqrt{3}-2i</math> und <math>z_3 = \sqrt{3}-2i</math>. | |
</div> | </div> | ||
<div class="exempel"> | <div class="exempel"> | ||
| - | ''' | + | ''' Beispiel 9''' |
| - | + | Löse die Gleichung <math>\ z^2 = \overline{z}\,</math>. | |
| - | + | Wenn für <math>z=a+ib</math>, <math>|\,z\,|=r</math> und <math>\arg z = \alpha</math> ist, ist für <math>\overline{z}= a-ib</math> <math>|\,\overline{z}\,|=r</math> und <math>\arg \overline{z} = - \alpha</math>. Also ist <math>z=r\,e^{i\alpha}</math> und <math>\overline{z} = r\,e^{-i\alpha}</math>. Die Gleichung lautet also | |
| - | {{ | + | {{Abgesetzte Formel||<math>(r\,e^{i\alpha})^2 = r\,e^{-i\alpha}\qquad\text{oder}\qquad r^2 e^{2i\alpha}= r\,e^{-i\alpha}\,\mbox{.}</math>}} |
| - | + | Wir sehen direkt, dass <math>r=0</math> eine der Lösungen ist und daher die Lösung <math>z=0</math> ergibt. Nehmen wir an, dass <math>r\not=0</math> erhalten wir die Gleichung <math>\ r\,e^{3i\alpha} = 1\,</math>. Vergleichen wir hier Betrag und Argument, erhalten wir | |
| - | {{ | + | {{Abgesetzte Formel||<math>\biggl\{\begin{align*} r &= 1\,\mbox{,}\\ 3\alpha &= 0 + 2k\pi\,\mbox{,}\end{align*}\qquad\Leftrightarrow\qquad\biggl\{\begin{align*} r &= 1\,\mbox{,}\\ \alpha &= 2k\pi/3\,\mbox{,}\quad k=0,1,2\,\mbox{.}\end{align*}</math>}} |
| - | + | Die Wurzeln sind also | |
*<math>\quad z_1 = e^0 = 1\,\mbox{,}</math> | *<math>\quad z_1 = e^0 = 1\,\mbox{,}</math> | ||
*<math>\quad z_2 = e^{2\pi i/ 3} = \cos\frac{2\pi}{3} + i\,\sin\frac{2\pi}{3} = -\frac{1}{2} + \frac{\sqrt3}{2}\,i\,\mbox{,}\vphantom{\Biggl(}</math> | *<math>\quad z_2 = e^{2\pi i/ 3} = \cos\frac{2\pi}{3} + i\,\sin\frac{2\pi}{3} = -\frac{1}{2} + \frac{\sqrt3}{2}\,i\,\mbox{,}\vphantom{\Biggl(}</math> | ||
| Zeile 262: | Zeile 259: | ||
| - | == | + | == D - Quadratische Ergänzung == |
| - | + | Die wohlbekannten Regeln | |
| - | {{ | + | {{Abgesetzte Formel||<math>\left\{\begin{align*} (a+b)^2 &= a^2+2ab+b^2\\ (a-b)^2 &= a^2-2ab+b^2\end{align*}\right.</math>}} |
| - | + | können auch verwendet werden, um quadratische Ausdrücke zu vereinfachen, zum Beispiel | |
| - | {{ | + | {{Abgesetzte Formel||<math>\begin{align*} x^2+4x+4 &= (x+2)^2\,\mbox{,}\\ x^2-10x+25 &= (x-5)^2\,\mbox{.}\end{align*}</math>}} |
| - | + | Dies kann verwendet werden, um quadratische Gleichungen zu lösen, zum Beispiel | |
| - | {{ | + | {{Abgesetzte Formel||<math>\begin{align*} x^2+4x+4 &= 9\,\mbox{,}\\ (x+2)^2 &= 9\,\mbox{.}\end{align*}</math>}} |
| - | + | Indem wir die Wurzeln berechnen, erhalten wir, dass <math>x+2=\pm\sqrt{9}</math> und, dass <math>x=-2\pm 3</math> und daher <math>x=1</math> oder <math>x=-5</math>. | |
| - | + | Manchmal muss man eine Konstante addieren oder subtrahieren, um eine der binomischen Formeln umgekehrt verwenden zu können. Zum Beispiel betrachten wir die Gleichung | |
| - | {{ | + | {{Abgesetzte Formel||<math>x^2+4x-5=0\,\mbox{.}</math>}} |
| - | + | Addieren wir 9 zu beiden Seiten, erhalten wir eine passende quadratische Form | |
| - | {{ | + | {{Abgesetzte Formel||<math>\begin{align*} x^2+4x-5+9 &= 0+9\,\mbox{}\\ x^2+4x+4\phantom{{}+9} &= 9\,\mbox{.}\end{align*}</math>}} |
| - | + | Diese Methode, quadratische Gleichungen zu lösen, nennt man ''quadratische Ergänzung''. | |
<div class="exempel"> | <div class="exempel"> | ||
| - | ''' | + | ''' Beispiel 10''' |
<ol type="a"> | <ol type="a"> | ||
| - | <li> | + | <li> Löse die Gleichung <math>\ x^2-6x+7=2\,</math>. |
<br> | <br> | ||
<br> | <br> | ||
| - | + | Der Koeffizient von <math>x</math> ist <math>-6</math> und daher müssen wir die Zahl <math>(-3)^2=9</math> als Konstante haben, um die quadratische Ergänzung verwenden zu können. Indem wir 2 auf beiden Seiten addieren, erhalten wir | |
| - | {{ | + | {{Abgesetzte Formel||<math>\begin{align*} x^2-6x+7+2 &= 2+2\\ x^2-6x+9\phantom{{}+2} &= 4\\ \rlap{(x-3)^2}\phantom{x^2-6x+7+2}{} &= 4\,\mbox{.}\end{align*}</math>}} |
| - | + | Wir erhalten also <math>x-3=\pm 2</math>. Daher ist <math>x=1</math> oder <math>x=5</math>. | |
</li> | </li> | ||
| - | <li> | + | <li> Löse die Gleichung <math>\ z^2+21=4-8z\,</math>. |
<br> | <br> | ||
<br> | <br> | ||
| - | + | Die Gleichung kann wie <math>z^2+8z+17=0</math> geschrieben werden. Indem wir 1 von beiden Seiten subtrahieren, erhalten wir | |
| - | {{ | + | {{Abgesetzte Formel||<math>\begin{align*} z^2+8z+17-1 &= 0-1\\ z^2+8z+16\phantom{{}-1} &= -1\\\ \rlap{(z+4)^2}\phantom{z^2+8z+17-1}{} &= -1\end{align*}</math>}} |
| - | + | und daher ist <math>z+4=\pm\sqrt{-1}</math>. Also sind die Wurzeln <math>z=-4-i</math> und <math>z=-4+i</math>. | |
| - | + | ||
</li> | </li> | ||
</ol> | </ol> | ||
| Zeile 317: | Zeile 313: | ||
</div> | </div> | ||
| - | + | Im Allgemeinen addiert oder subtrahiert man eine Konstante, sodass die Konstante auf der linken Seite der Gleichung das Quadrat des halben Koeffizienten des ''x''-Terms ist. Diese Methode funktioniert auch für komplexe Gleichungen. | |
<div class="exempel"> | <div class="exempel"> | ||
| - | ''' | + | ''' Beispiel 11''' |
| - | + | Löse die Gleichung <math>\ x^2-\frac{8}{3}x+1=2\,</math>. | |
| - | + | Der halbe Koeffizient von <math>x</math> ist <math>-\tfrac{4}{3}</math>. Also müssen wir <math>\bigl(-\tfrac{4}{3}\bigr)^2=\tfrac{16}{9}</math> auf beiden Seiten addieren | |
| - | {{ | + | {{Abgesetzte Formel||<math>\begin{align*} x^2-\tfrac{8}{3}x+\tfrac{16}{9}+1 &= 2+\tfrac{16}{9}\\ \rlap{\bigl(x-\tfrac{4}{3}\bigr)^2}\phantom{x^2-\tfrac{8}{3}x+\tfrac{16}{9}}{}+1 &= \tfrac{34}{9}\\ \rlap{\bigl(x-\tfrac{4}{3}\bigr)^2}\phantom{x^2-\tfrac{8}{3}x+\tfrac{16}{9}+1} &= \tfrac{25}{9}\,\mbox{.}\end{align*}</math>}} |
| - | + | Wir sehen, dass <math>x-\tfrac{4}{3}=\pm\tfrac{5}{3}</math> und erhalten dadurch, dass <math>x=\tfrac{4}{3}\pm\tfrac{5}{3}</math>, also <math>x=-\tfrac{1}{3}</math> oder <math>x=3</math>. | |
</div> | </div> | ||
<div class="exempel"> | <div class="exempel"> | ||
| - | ''' | + | ''' Beispiel 12''' |
| - | + | Löse die Gleichung <math>\ x^2+px+q=0\,</math>. | |
| - | + | Durch quadratische Ergänzung erhalten wir | |
| - | {{ | + | {{Abgesetzte Formel||<math>\begin{align*} x^2+px+\Bigl(\frac{p}{2}\Bigr)^2+q &= \Bigl(\frac{p}{2}\Bigr)^2\\ \rlap{\Bigl(x+\frac{p}{2}\Bigr)^2}\phantom{x^2+px+\Bigl(\frac{p}{2}\Bigr)^2+q}{} &= \Bigl(\frac{p}{2}\Bigr)^2-q\\ \rlap{x+\frac{p}{2}}\phantom{x^2+px+\Bigl(\frac{p}{2}\Bigr)^2+q}{} &= \pm \sqrt{\Bigl(\frac{p}{2}\Bigr)^2-q}\ \mbox{.}\end{align*}</math>}} |
| - | + | Dadurch erhalten wir eine allgemeine Lösungsformel für quadratische Gleichungen | |
| - | {{ | + | {{Abgesetzte Formel||<math>x=-\frac{p}{2}\pm \sqrt{\Bigl(\frac{p}{2}\Bigr)^2-q}\,\mbox{.}</math>}} |
</div> | </div> | ||
<div class="exempel"> | <div class="exempel"> | ||
| - | ''' | + | ''' Beispiel 13''' |
| - | + | Löse die Gleichung <math>\ z^2-(12+4i)z-4+24i=0\,</math>. | |
| - | Half the coefficient of <math>z</math> is <math>-(6+2i)</math> so we add the square of this expression to both sides | ||
| - | + | Der halbe Koeffizient von <math>z</math> ist <math>-(6+2i)</math>. Daher addieren wir das Quadrat des Koeffizienten auf beiden Seiten der Gleichung | |
| - | + | {{Abgesetzte Formel||<math>z^2-(12+4i)z+(-(6+2i))^2-4+24i=(-(6+2i))^2\,\mbox{.}</math>}} | |
| - | + | Erweitern wir die rechte Seite <math>\ (-(6+2i))^2=36+24i+4i^2=32+24i\ </math> und ergänzen die linke Seite quadratisch, erhalten wir | |
| - | + | {{Abgesetzte Formel||<math>\begin{align*} (z-(6+2i))^2-4+24i &= 32+24i\\ \rlap{(z-(6+2i))^2}\phantom{(z-(6+2i))^2-4+24i}{} &= 36\,\mbox{.}\end{align*}</math>}} | |
| + | |||
| + | Wir erhalten <math>\ z-(6+2i)=\pm 6\ </math> und daher die Wurzeln <math>z=12+2i</math> und <math>z=2i</math>. | ||
</div> | </div> | ||
| - | + | Man kann auch einen Ausdruck quadratisch ergänzen, indem man dieselbe Konstante vom Ausdruck subtrahiert und addiert. Das Ziel dabei ist, dass die Variable nur noch in der quadrierten Klammer steht, und nicht mehr außerhalb. Zum Beispiel | |
| - | {{ | + | {{Abgesetzte Formel||<math>\begin{align*} x^2+10x+3 &= x^2+10x+25+3-25\\ &= (x+5)^2-22\,\mbox{.}\end{align*}</math>}} |
<div class="exempel"> | <div class="exempel"> | ||
| - | ''' | + | ''' Beispiel 14''' |
| - | + | Ergänze <math>\ z^2+(2-4i)z+1-3i\,</math> quadratisch. | |
| - | + | Wir subtrahieren und addieren <math>\bigl(\frac{1}{2}(2-4i)\bigr)^2=(1-2i)^2=-3-4i\,</math> vom Ausdruck, | |
| - | {{ | + | {{Abgesetzte Formel||<math>\begin{align*} z^2+(2-4i)z+1-3i &= z^2+(2-4i)z+(1-2i)^2-(1-2i)^2+1-3i\\ &= \bigl(z+(1-2i)\bigr)^2-(1-2i)^2+1-3i\\ &= \bigl(z+(1-2i)\bigr)^2-(-3-4i)+1-3i\\ &= \bigl(z+(1-2i)\bigr)^2+4+i\,\mbox{.}\end{align*}</math>}} |
</div> | </div> | ||
| - | == | + | == E - Lösungen mit der allgemeinen Lösungsformel== |
| - | + | Manchmal ist es am einfachsten, quadratische Gleichungen mit der allgemeinen Lösungsformel zu lösen. Bei komplexen Gleichungen können dann aber Terme wie <math>\sqrt{a+ib}</math> entstehen. Man kann dann annehmen, dass | |
| - | {{ | + | {{Abgesetzte Formel||<math>z=x+iy=\sqrt{a+ib}\,\mbox{.}</math>}} |
| - | + | Quadrieren wir beide Seiten, erhalten wir | |
| - | {{ | + | {{Abgesetzte Formel||<math>\begin{align*} (x+iy)^2 &= a+ib\\ x^2 - y^2 + 2xy\,i &= a+ib\,\mbox{.}\end{align*}</math>}} |
| - | + | Indem wir den Real- und Imaginärteil vergleichen, erhalten wir | |
| - | {{ | + | {{Abgesetzte Formel||<math>\left\{\begin{align*} &x^2 - y^2 = a\,\mbox{,}\\ &2xy=b\,\mbox{.}\end{align*}\right.</math>}} |
| - | + | Diese Gleichungen löst man zum Beispiel, indem man <math>y= b/(2x)</math> in der ersten Gleichung ersetzt. | |
<div class="exempel"> | <div class="exempel"> | ||
| - | ''' | + | ''' Beispiel 15''' |
| - | + | Berechne <math>\ \sqrt{-3-4i}\,</math>. | |
| - | + | Wir nehmen an, dass <math>\ x+iy=\sqrt{-3-4i}\ </math>, wobei <math>x</math> und <math>y</math> reelle Zahlen sind. Quadrieren wir beide Seiten, erhalten wir | |
| - | {{ | + | {{Abgesetzte Formel||<math>\begin{align*} (x+iy)^2 &= -3-4i\\ x^2 - y^2 + 2xyi &= -3-4i\end{align*}</math>}} |
| - | + | und wir erhalten die beiden Gleichungen | |
| - | {{ | + | {{Abgesetzte Formel||<math>\Bigl\{\begin{align*} x^2 - y^2 &= -3\,\mbox{,}\\ 2xy&= -4\,\mbox{.}\end{align*}</math>}} |
| - | + | Von der zweiten Gleichung erhalten wir <math>\ y=-4/(2x) = -2/x\ </math>. Das in der ersten Gleichung substituiert, ergibt | |
| - | {{ | + | {{Abgesetzte Formel||<math>x^2-\frac{4}{x^2} = -3 \quad \Leftrightarrow \quad x^4 +3x^2 - 4=0\,\mbox{.}</math>}} |
| - | + | Dies ist eine quadratische Gleichung für <math>x^2</math>, die wir am einfachsten lösen, indem wir <math>t=x^2</math> substituieren | |
| - | {{ | + | {{Abgesetzte Formel||<math>t^2 +3t -4=0\,\mbox{.}</math>}} |
| - | + | Die Lösungen sind <math>t = 1</math> und <math>t = -4</math>. Die letzte Lösung ist nicht gültig, da <math>x</math> und <math>y</math> reell sein müssen (nach unserer Annahme). Wir erhalten also die Lösungen <math>x=\pm\sqrt{1}</math> und dadurch | |
| - | * <math>\ x=-1\ </math> | + | * <math>\ x=-1\ </math> ergibt, dass <math>\ y=-2/(-1)=2\,</math>, |
| - | * <math>\ x=1\ </math> | + | * <math>\ x=1\ </math> ergibt, dass <math>\ y=-2/1=-2\,</math>. |
| - | + | Also ist | |
| - | {{ | + | {{Abgesetzte Formel||<math>\sqrt{-3-4i} = \biggl\{\begin{align*} &\phantom{-}1-2i\,\mbox{,}\\ &-1+2i\,\mbox{.}\end{align*}</math>}} |
</div> | </div> | ||
<div class="exempel"> | <div class="exempel"> | ||
| - | ''' | + | ''' Beispiel 16''' |
<ol type="a"> | <ol type="a"> | ||
| - | <li> | + | <li> Löse die Gleichung <math>\ z^2-2z+10=0\,</math>. |
<br> | <br> | ||
<br> | <br> | ||
| - | + | Wir erhalten durch die allgemeine Lösungsformel (siehe Beispiel 12) | |
| - | {{ | + | {{Abgesetzte Formel||<math>z= 1\pm \sqrt{1-10} = 1\pm \sqrt{-9}= 1\pm 3i\,\mbox{.}</math>}} |
</li> | </li> | ||
| - | <li> | + | <li> Löse die Gleichung <math>\ z^2 + (4-2i)z -4i=0\,\mbox{.}</math> |
<br> | <br> | ||
<br> | <br> | ||
| - | + | Wir verwenden wieder die Lösungsformel und erhalten | |
| - | {{ | + | {{Abgesetzte Formel||<math>\begin{align*} z &= -2+i\pm\sqrt{\smash{(-2+i)^2+4i}\vphantom{i^2}} = -2+i\pm\sqrt{4-4i+i^{\,2}+4i}\\ &=-2+i\pm\sqrt{3} = -2\pm\sqrt{3}+i\,\mbox{.}\end{align*}</math>}} |
</li> | </li> | ||
| - | <li> | + | <li> Löse die Gleichung <math>\ iz^2+(2+6i)z+2+11i=0\,\mbox{.}</math> |
<br> | <br> | ||
<br> | <br> | ||
| - | Division | + | Division auf beiden Seiten durch <math>i</math> ergibt |
| - | {{ | + | {{Abgesetzte Formel||<math>\begin{align*} z^2 + \frac{2+6i}{i}z +\frac{2+11i}{i} &= 0\\ z^2+ (6-2i)z + 11-2i &= 0\,\mbox{.}\end{align*}</math>}} |
| - | + | Durch die Lösungsformel erhalten wir | |
| - | {{ | + | {{Abgesetzte Formel||<math>\begin{align*} z &= -3+i \pm \sqrt{\smash{(-3+i)^2 -(11-2i)}\vphantom{i^2}}\\ &= -3+i \pm \sqrt{-3-4i}\\ &= -3+i\pm(1-2i)\,\mbox{.}\end{align*}</math>}} |
| - | + | indem wir das Beispiel 15 verwenden, um <math>\ \sqrt{-3-4i}\ </math> zu erhalten. Die Lösungen sind daher | |
| - | {{ | + | {{Abgesetzte Formel||<math>z=\biggl\{\begin{align*} &-2-i\,\mbox{,}\\ &-4+3i\,\mbox{.}\end{align*}</math>}} |
</li> | </li> | ||
</ol> | </ol> | ||
</div> | </div> | ||
| + | <br><br> | ||
| + | |||
| + | Noch Fragen zu diesem Kapitel? Dann schau nach im Kursforum (Du findest den Link in der Student Lounge) oder frag nach per Skype bei ombTutor <skype style="call" action="call">ombTutor</skype> <skype style="chat" action="chat">ombTutor</skype> | ||
| + | |||
| + | Keine Fragen mehr? Dann mache weiter mit den '''[[3.3 Übungen|Übungen]]''' . | ||
Aktuelle Version
| Theorie | Übungen |
Inhalt:
- Der Moivresche Satz
- Quadratische Gleichungen
- Exponentialfunktionen
- Quadratische Ergänzung
Lernziele:
Nach diesem Abschnitt solltest Du folgendes wissen:
- Wie man Potenzen von komplexen Zahlen mit dem Moivreschen Satz löst.
- Wie man Wurzeln von komplexen Zahlen berechnet, indem man die Zahl in Polarform bringt.
- Wie man komplexe quadratische Ausdrücke quadratisch ergänzt.
- Wie man komplexe quadratische Gleichungen löst.
Die Lernziele sind Dir aus der Schule noch bestens vertraut und Du weißt ganz genau, wie man die zugehörigen Rechnungen ausführt? Dann kannst Du auch gleich mit den Prüfungen beginnen (Du findest den Link in der Student Lounge).
A - Moivrescher Satz
Die Rechenregeln  zw
zw =
= z
z
 w
w
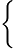 arg(z arg(z z)=argz+argz z)=argz+argz z z z z = = z z   z z 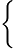 argz3=3argz argz3=3argz z3 z3 = = z z 3etc. 3etc. |
Für eine beliebige komplexe Zahl  +isin
+isin )
)
 r(cos r(cos +isin +isin ) ) n=rn(cosn n=rn(cosn +isinn +isinn ). ). |
Falls  z
z =1
=1
 +isin +isin )n=cosn )n=cosn +isinn +isinn . . |
Diese Regel nennt man den Moivreschen Satz. Wie wir sehen werden, ist diese Regel sehr wichtig, wenn man Potenzen und Wurzeln von komplexen Zahlen berechnet.
Beispiel 1
Bestimme  21+i
21+i
Wir schreiben  2+i
2+i 2=1
2=1
 cos
cos 4+isin
4+isin 4
4
 cos cos 4+isin 4+isin 4 4 3=cos43 3=cos43 +isin43 +isin43 =−1 =−1 2+1 2+1 2i= 2i= 2−1+i= 2−1+i= cos cos 4+isin 4+isin 4 4 100=cos4100 100=cos4100 +isin4100 +isin4100 =cos25 =cos25 +isin25 +isin25 =cos =cos +isin +isin =−1. =−1. |
Beispiel 2
Mit der binomischen Formel können wir den Ausdruck wie folgt erläutern:
aber wir können auch den Moivreschen Satz benutzen. Dann erhalten wir:
Da die beiden Ausdrücke gleich sind, erhalten wir, indem wir die Real- und Imaginärteile gleichsetzen, die bekannten trigonometrischen Identitäten
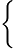 cos2vsin2v=cos2v−sin2v,=2sinvcosv. cos2vsin2v=cos2v−sin2v,=2sinvcosv. |
Beispiel 3
Vereinfache  3+i)14(1+i
3+i)14(1+i 3)7(1+i)10
3)7(1+i)10
Wir schreiben die Zahlen  3+i
3+i  3
3
 3+i=2
3+i=2 cos
cos 6+isin
6+isin 6
6
1+i , 3=2
3=2 cos
cos 3+isin
3+isin 3
3
1+i= . 2
2 cos
cos 4+isin
4+isin 4
4
Nach dem Moivreschen Satz erhalten wir
 3+i)14(1+i 3+i)14(1+i 3)7(1+i)10=214 3)7(1+i)10=214 cos614 cos614 +isin614 +isin614  27 27 cos37 cos37 +isin37 +isin37  ( ( 2)10 2)10 cos410 cos410 +isin410 +isin410  |
Diesen Ausdruck können wir weiter vereinfachen, indem wir die Multiplikations- und Divisionsregeln für komplexe Zahlen in Polarform verwenden
 cos629 cos629 +isin629 +isin629  214 214 cos614 cos614 +isin614 +isin614  =22 =22 cos cos −615 −615  +isin +isin −615 −615   =4 =4 cos cos − − 2 2 +isin +isin − − 2 2  =−4i. =−4i. |
B - nte Wurzeln von komplexen Zahlen
Eine komplexe Zahl
Lösungen dieser Wurzelgleichung erhält man, indem man beide Zahlen in Polarform bringt und deren Betrag und Argument vergleicht.
Ist eine Zahl  w
w (cos
(cos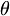 +isin
+isin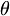 )
) +isin
+isin )
)
 +isinn +isinn )= )= w w (cos (cos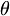 +isin +isin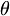 ), ), |
wo wir den Moivreschen Satz auf der linken Seite angewendet haben. Vergleichen wir das Argument und den Betrag der beiden Seiten, erhalten wir
| \displaystyle \biggl\{\begin{align*} r^{\,n} &= |w|\,\mbox{,}\\ n\alpha &= \theta + k\cdot 2\pi\,\mbox{.}\end{align*} |
Beachte hier, dass wir ein Vielfaches von \displaystyle 2\pi zum Argument addiert haben, um alle Lösungen zu erhalten.
| \displaystyle \biggl\{\begin{align*} r &={\textstyle\sqrt[\scriptstyle n]{|w|}},\\ \alpha &= (\theta + 2k\pi)/n\,, \quad k=0, \pm 1, \pm 2, \ldots\end{align*} |
Wir erhalten also einen Wert für \displaystyle r, aber unendlich viele Werte für \displaystyle \alpha. Trotzdem gibt es nicht unendlich viele Lösungen dieser Gleichung. Für Werte von \displaystyle k zwischen \displaystyle k = 0 und \displaystyle k = n - 1 erhalten wir verschiedene Argumente für \displaystyle z und daher verschiedene Zahlen \displaystyle z. Für andere Werte von \displaystyle k wiederholen wir nur die schon bekannten Lösungen, da die Funktionen \displaystyle \cos \theta und \displaystyle \sin \theta periodisch sind und die Periodenlänge \displaystyle 2 \pi haben. Also hat eine Gleichung mit der Form \displaystyle z^n=w genau \displaystyle n Wurzeln.
Hinweis: Beachte, dass die Argumente der Lösungen sich immer um \displaystyle 2\pi/n unterscheiden. Also sind die Lösungen gleichförmig auf dem Kreis mit dem Radius \displaystyle \sqrt[\scriptstyle n]{|w|} verteilt und bilden ein n-seitiges Polygon.
Beispiel 4
Löse die Gleichung \displaystyle \ z^4= 16\,i\,.
Wir schreiben \displaystyle z und \displaystyle 16\,i in Polarform
- \displaystyle \quad z=r\,(\cos \alpha + i\,\sin \alpha)\,,
- \displaystyle \quad 16\,i= 16\Bigl(\cos\frac{\pi}{2} + i\,\sin\frac{\pi}{2}\,\Bigr)\vphantom{\biggl(}.
Die Gleichung \displaystyle \ z^4=16\,i\ wird also
| \displaystyle r^4\,(\cos 4\alpha + i\,\sin 4\alpha) = 16\Bigl(\cos\frac{\pi}{2} + i\sin\frac{\pi}{2}\,\Bigr)\,\mbox{.} |
Vergleichen wir das Argument und den Betrag der beiden Seiten, erhalten wir
| \displaystyle \biggl\{\begin{align*} r^4 &= 16,\\ 4\alpha &= \pi/2 + k\cdot 2\pi,\end{align*}\qquad\text{d.h.}\qquad\biggl\{\begin{align*} r &= \sqrt[\scriptstyle 4]{16}= 2, \\ \alpha &= \pi/8 + k\pi/2\,,\quad k=0,1,2,3.\end{align*} |
|
Die Wurzeln der Gleichung sind daher
|
|
C - Exponentialform der komplexen Zahlen
Wenn wir \displaystyle i als eine normale Zahl betrachten und die komplexe Zahl \displaystyle z wie eine Funktion von nur \displaystyle \alpha betrachten (in der \displaystyle r also konstant ist), ergibt sich
| \displaystyle f(\alpha) = r\,(\cos \alpha + i\,\sin \alpha) |
und wir erhalten durch wiederholte Ableitung
| \displaystyle \begin{align*} f^{\,\prime}(\alpha) &= -r\sin \alpha + r\,i\,\cos \alpha = r\,i^2 \sin \alpha + r\,i\,\cos \alpha = i\,r\,(\cos \alpha + i\,\sin \alpha) = i\,f(\alpha)\\ f^{\,\prime\prime} (\alpha) &= - r\,\cos \alpha - r\,i\,\sin \alpha = i^2\,r\,(\cos \alpha + i\,\sin \alpha) = i^2\, f(\alpha)\cr &\text{etc.}\end{align*} |
Die einzigen reellen Funktionen, die dies erfüllen, sind Funktionen in der Form \displaystyle f(x)= e^{\,kx}. Daher stammt folgende Definition:
| \displaystyle e^{\,i\alpha} = \cos \alpha + i\,\sin \alpha\,\mbox{.} |
Dies ist auch eine Verallgemeinerung der reellen Exponentialfunktion für komplexe Zahlen. Ersetzen wir \displaystyle z=a+ib erhalten wir
| \displaystyle e^{\,z} = e^{\,a+ib} = e^{\,a} \, e^{\,ib} = e^{\,a}(\cos b + i\,\sin b)\,\mbox{.} |
Die Definition von \displaystyle e^{\,z} kann wie eine Kurzform der Polarform verwendet werden, da \displaystyle z=r\,(\cos \alpha + i\,\sin \alpha) = r\,e^{\,i\alpha}\,.
Beispiel 5
Für eine reelle Zahl \displaystyle z ist die Definition dieselbe wie für die reelle Exponentialfunktion. Da \displaystyle z=a+0\cdot i erhalten wir
| \displaystyle e^{\,z} = e^{\,a+0\cdot i} = e^a (\cos 0 + i \sin 0) = e^a \cdot 1 = e^a\,\mbox{.} |
Beispiel 6
Eine weitere Folgerung aus dieser Definition erhalten wir durch den Moivreschen Satz.
| \displaystyle \bigl(e^{\,i\alpha}\bigr)^n = (\cos \alpha + i \sin \alpha)^n = \cos n\alpha + i \sin n \alpha = e^{\,in\alpha} |
Das erinnert uns an die wohlbekannte Rechenregel für Potenzen.
| \displaystyle \left(a^x\right)^y = a^{x\,y} |
Beispiel 7
Mit den Definitionen oben erhalten wir die Formel
| \displaystyle e^{\pi\,i} = \cos \pi + i \sin \pi = -1 |
Diese berühmte Formel wurde von Euler zu Beginn des 18. Jahrhunderts entdeckt.
Beispiel 8
Löse die Gleichung \displaystyle \ (z+i)^3 = -8i.
Wir lassen \displaystyle w = z + i sein. Wir erhalten so die Gleichung \displaystyle \ w^3=-8i\,. Wir bringen als ersten Schritt \displaystyle w und \displaystyle -8i in Polarform
- \displaystyle \quad w=r\,(\cos \alpha + i\,\sin \alpha) = r\,e^{i\alpha}\,\mbox{,}
- \displaystyle \quad -8i = 8\Bigl(\cos \frac{3\pi}{2} + i\,\sin\frac{3\pi}{2}\,\Bigr) = 8\,e^{3\pi i/2}\vphantom{\biggl(}\,\mbox{.}
In Polarform lautet die Gleichung \displaystyle \ r^3e^{3\alpha i}=8\,e^{3\pi i/2}\ . Vergleichen wir das Argument und den Betrag der rechten und linken Seite, erhalten wir
| \displaystyle \biggl\{\begin{align*} r^3 &= 8\,\mbox{,}\\ 3\alpha &= 3\pi/2+2k\pi\,\mbox{,}\end{align*}\qquad\Leftrightarrow\qquad\biggl\{\begin{align*} r&=\sqrt[\scriptstyle 3]{8}\,\mbox{,}\\ \alpha&= \pi/2+2k\pi/3\,,\quad k=0,1,2\,\mbox{.}\end{align*} |
Die Wurzeln der Gleichung sind daher
- \displaystyle \quad w_1 = 2\,e^{\pi i/2} = 2\Bigl(\cos \frac{\pi}{2} + i\,\sin\frac{\pi}{2}\,\Bigr) = 2i\,\mbox{,}\quad\vphantom{\biggl(}
- \displaystyle \quad w_2 = 2\,e^{7\pi i/6} = 2\Bigl(\cos\frac{7\pi}{6} + i\,\sin\frac{7\pi}{6}\,\Bigr) = -\sqrt{3}-i\,\mbox{,}\quad\vphantom{\Biggl(}
- \displaystyle \quad w_3 = 2\,e^{11\pi i/6} = 2\Bigl(\cos\frac{11\pi}{6} + i\,\sin\frac{11\pi}{6}\,\Bigr) = \sqrt{3}-i\,\mbox{,}\quad\vphantom{\biggl(}
also sind \displaystyle z_1 = 2i-i=i, \displaystyle z_2 = - \sqrt{3}-2i und \displaystyle z_3 = \sqrt{3}-2i.
Beispiel 9
Löse die Gleichung \displaystyle \ z^2 = \overline{z}\,.
Wenn für \displaystyle z=a+ib, \displaystyle |\,z\,|=r und \displaystyle \arg z = \alpha ist, ist für \displaystyle \overline{z}= a-ib \displaystyle |\,\overline{z}\,|=r und \displaystyle \arg \overline{z} = - \alpha. Also ist \displaystyle z=r\,e^{i\alpha} und \displaystyle \overline{z} = r\,e^{-i\alpha}. Die Gleichung lautet also
| \displaystyle (r\,e^{i\alpha})^2 = r\,e^{-i\alpha}\qquad\text{oder}\qquad r^2 e^{2i\alpha}= r\,e^{-i\alpha}\,\mbox{.} |
Wir sehen direkt, dass \displaystyle r=0 eine der Lösungen ist und daher die Lösung \displaystyle z=0 ergibt. Nehmen wir an, dass \displaystyle r\not=0 erhalten wir die Gleichung \displaystyle \ r\,e^{3i\alpha} = 1\,. Vergleichen wir hier Betrag und Argument, erhalten wir
| \displaystyle \biggl\{\begin{align*} r &= 1\,\mbox{,}\\ 3\alpha &= 0 + 2k\pi\,\mbox{,}\end{align*}\qquad\Leftrightarrow\qquad\biggl\{\begin{align*} r &= 1\,\mbox{,}\\ \alpha &= 2k\pi/3\,\mbox{,}\quad k=0,1,2\,\mbox{.}\end{align*} |
Die Wurzeln sind also
- \displaystyle \quad z_1 = e^0 = 1\,\mbox{,}
- \displaystyle \quad z_2 = e^{2\pi i/ 3} = \cos\frac{2\pi}{3} + i\,\sin\frac{2\pi}{3} = -\frac{1}{2} + \frac{\sqrt3}{2}\,i\,\mbox{,}\vphantom{\Biggl(}
- \displaystyle \quad z_3 = e^{4\pi i/ 3} = \cos\frac{4\pi}{3} + i\,\sin\frac{4\pi}{3} = -\frac{1}{2} - \frac{\sqrt3}{2}\,i\,\mbox{,}
- \displaystyle \quad z_4 = 0\,\mbox{.}
D - Quadratische Ergänzung
Die wohlbekannten Regeln
| \displaystyle \left\{\begin{align*} (a+b)^2 &= a^2+2ab+b^2\\ (a-b)^2 &= a^2-2ab+b^2\end{align*}\right. |
können auch verwendet werden, um quadratische Ausdrücke zu vereinfachen, zum Beispiel
| \displaystyle \begin{align*} x^2+4x+4 &= (x+2)^2\,\mbox{,}\\ x^2-10x+25 &= (x-5)^2\,\mbox{.}\end{align*} |
Dies kann verwendet werden, um quadratische Gleichungen zu lösen, zum Beispiel
| \displaystyle \begin{align*} x^2+4x+4 &= 9\,\mbox{,}\\ (x+2)^2 &= 9\,\mbox{.}\end{align*} |
Indem wir die Wurzeln berechnen, erhalten wir, dass \displaystyle x+2=\pm\sqrt{9} und, dass \displaystyle x=-2\pm 3 und daher \displaystyle x=1 oder \displaystyle x=-5.
Manchmal muss man eine Konstante addieren oder subtrahieren, um eine der binomischen Formeln umgekehrt verwenden zu können. Zum Beispiel betrachten wir die Gleichung
| \displaystyle x^2+4x-5=0\,\mbox{.} |
Addieren wir 9 zu beiden Seiten, erhalten wir eine passende quadratische Form
| \displaystyle \begin{align*} x^2+4x-5+9 &= 0+9\,\mbox{}\\ x^2+4x+4\phantom{{}+9} &= 9\,\mbox{.}\end{align*} |
Diese Methode, quadratische Gleichungen zu lösen, nennt man quadratische Ergänzung.
Beispiel 10
- Löse die Gleichung \displaystyle \ x^2-6x+7=2\,.
Der Koeffizient von \displaystyle x ist \displaystyle -6 und daher müssen wir die Zahl \displaystyle (-3)^2=9 als Konstante haben, um die quadratische Ergänzung verwenden zu können. Indem wir 2 auf beiden Seiten addieren, erhalten wir\displaystyle \begin{align*} x^2-6x+7+2 &= 2+2\\ x^2-6x+9\phantom{{}+2} &= 4\\ \rlap{(x-3)^2}\phantom{x^2-6x+7+2}{} &= 4\,\mbox{.}\end{align*} Wir erhalten also \displaystyle x-3=\pm 2. Daher ist \displaystyle x=1 oder \displaystyle x=5.
- Löse die Gleichung \displaystyle \ z^2+21=4-8z\,.
Die Gleichung kann wie \displaystyle z^2+8z+17=0 geschrieben werden. Indem wir 1 von beiden Seiten subtrahieren, erhalten wir\displaystyle \begin{align*} z^2+8z+17-1 &= 0-1\\ z^2+8z+16\phantom{{}-1} &= -1\\\ \rlap{(z+4)^2}\phantom{z^2+8z+17-1}{} &= -1\end{align*} und daher ist \displaystyle z+4=\pm\sqrt{-1}. Also sind die Wurzeln \displaystyle z=-4-i und \displaystyle z=-4+i.
Im Allgemeinen addiert oder subtrahiert man eine Konstante, sodass die Konstante auf der linken Seite der Gleichung das Quadrat des halben Koeffizienten des x-Terms ist. Diese Methode funktioniert auch für komplexe Gleichungen.
Beispiel 11
Löse die Gleichung \displaystyle \ x^2-\frac{8}{3}x+1=2\,.
Der halbe Koeffizient von \displaystyle x ist \displaystyle -\tfrac{4}{3}. Also müssen wir \displaystyle \bigl(-\tfrac{4}{3}\bigr)^2=\tfrac{16}{9} auf beiden Seiten addieren
| \displaystyle \begin{align*} x^2-\tfrac{8}{3}x+\tfrac{16}{9}+1 &= 2+\tfrac{16}{9}\\ \rlap{\bigl(x-\tfrac{4}{3}\bigr)^2}\phantom{x^2-\tfrac{8}{3}x+\tfrac{16}{9}}{}+1 &= \tfrac{34}{9}\\ \rlap{\bigl(x-\tfrac{4}{3}\bigr)^2}\phantom{x^2-\tfrac{8}{3}x+\tfrac{16}{9}+1} &= \tfrac{25}{9}\,\mbox{.}\end{align*} |
Wir sehen, dass \displaystyle x-\tfrac{4}{3}=\pm\tfrac{5}{3} und erhalten dadurch, dass \displaystyle x=\tfrac{4}{3}\pm\tfrac{5}{3}, also \displaystyle x=-\tfrac{1}{3} oder \displaystyle x=3.
Beispiel 12
Löse die Gleichung \displaystyle \ x^2+px+q=0\,.
Durch quadratische Ergänzung erhalten wir
| \displaystyle \begin{align*} x^2+px+\Bigl(\frac{p}{2}\Bigr)^2+q &= \Bigl(\frac{p}{2}\Bigr)^2\\ \rlap{\Bigl(x+\frac{p}{2}\Bigr)^2}\phantom{x^2+px+\Bigl(\frac{p}{2}\Bigr)^2+q}{} &= \Bigl(\frac{p}{2}\Bigr)^2-q\\ \rlap{x+\frac{p}{2}}\phantom{x^2+px+\Bigl(\frac{p}{2}\Bigr)^2+q}{} &= \pm \sqrt{\Bigl(\frac{p}{2}\Bigr)^2-q}\ \mbox{.}\end{align*} |
Dadurch erhalten wir eine allgemeine Lösungsformel für quadratische Gleichungen
| \displaystyle x=-\frac{p}{2}\pm \sqrt{\Bigl(\frac{p}{2}\Bigr)^2-q}\,\mbox{.} |
Beispiel 13
Löse die Gleichung \displaystyle \ z^2-(12+4i)z-4+24i=0\,.
Der halbe Koeffizient von \displaystyle z ist \displaystyle -(6+2i). Daher addieren wir das Quadrat des Koeffizienten auf beiden Seiten der Gleichung
| \displaystyle z^2-(12+4i)z+(-(6+2i))^2-4+24i=(-(6+2i))^2\,\mbox{.} |
Erweitern wir die rechte Seite \displaystyle \ (-(6+2i))^2=36+24i+4i^2=32+24i\ und ergänzen die linke Seite quadratisch, erhalten wir
| \displaystyle \begin{align*} (z-(6+2i))^2-4+24i &= 32+24i\\ \rlap{(z-(6+2i))^2}\phantom{(z-(6+2i))^2-4+24i}{} &= 36\,\mbox{.}\end{align*} |
Wir erhalten \displaystyle \ z-(6+2i)=\pm 6\ und daher die Wurzeln \displaystyle z=12+2i und \displaystyle z=2i.
Man kann auch einen Ausdruck quadratisch ergänzen, indem man dieselbe Konstante vom Ausdruck subtrahiert und addiert. Das Ziel dabei ist, dass die Variable nur noch in der quadrierten Klammer steht, und nicht mehr außerhalb. Zum Beispiel
| \displaystyle \begin{align*} x^2+10x+3 &= x^2+10x+25+3-25\\ &= (x+5)^2-22\,\mbox{.}\end{align*} |
Beispiel 14
Ergänze \displaystyle \ z^2+(2-4i)z+1-3i\, quadratisch.
Wir subtrahieren und addieren \displaystyle \bigl(\frac{1}{2}(2-4i)\bigr)^2=(1-2i)^2=-3-4i\, vom Ausdruck,
| \displaystyle \begin{align*} z^2+(2-4i)z+1-3i &= z^2+(2-4i)z+(1-2i)^2-(1-2i)^2+1-3i\\ &= \bigl(z+(1-2i)\bigr)^2-(1-2i)^2+1-3i\\ &= \bigl(z+(1-2i)\bigr)^2-(-3-4i)+1-3i\\ &= \bigl(z+(1-2i)\bigr)^2+4+i\,\mbox{.}\end{align*} |
E - Lösungen mit der allgemeinen Lösungsformel
Manchmal ist es am einfachsten, quadratische Gleichungen mit der allgemeinen Lösungsformel zu lösen. Bei komplexen Gleichungen können dann aber Terme wie \displaystyle \sqrt{a+ib} entstehen. Man kann dann annehmen, dass
| \displaystyle z=x+iy=\sqrt{a+ib}\,\mbox{.} |
Quadrieren wir beide Seiten, erhalten wir
| \displaystyle \begin{align*} (x+iy)^2 &= a+ib\\ x^2 - y^2 + 2xy\,i &= a+ib\,\mbox{.}\end{align*} |
Indem wir den Real- und Imaginärteil vergleichen, erhalten wir
| \displaystyle \left\{\begin{align*} &x^2 - y^2 = a\,\mbox{,}\\ &2xy=b\,\mbox{.}\end{align*}\right. |
Diese Gleichungen löst man zum Beispiel, indem man \displaystyle y= b/(2x) in der ersten Gleichung ersetzt.
Beispiel 15
Berechne \displaystyle \ \sqrt{-3-4i}\,.
Wir nehmen an, dass \displaystyle \ x+iy=\sqrt{-3-4i}\ , wobei \displaystyle x und \displaystyle y reelle Zahlen sind. Quadrieren wir beide Seiten, erhalten wir
| \displaystyle \begin{align*} (x+iy)^2 &= -3-4i\\ x^2 - y^2 + 2xyi &= -3-4i\end{align*} |
und wir erhalten die beiden Gleichungen
| \displaystyle \Bigl\{\begin{align*} x^2 - y^2 &= -3\,\mbox{,}\\ 2xy&= -4\,\mbox{.}\end{align*} |
Von der zweiten Gleichung erhalten wir \displaystyle \ y=-4/(2x) = -2/x\ . Das in der ersten Gleichung substituiert, ergibt
| \displaystyle x^2-\frac{4}{x^2} = -3 \quad \Leftrightarrow \quad x^4 +3x^2 - 4=0\,\mbox{.} |
Dies ist eine quadratische Gleichung für \displaystyle x^2, die wir am einfachsten lösen, indem wir \displaystyle t=x^2 substituieren
| \displaystyle t^2 +3t -4=0\,\mbox{.} |
Die Lösungen sind \displaystyle t = 1 und \displaystyle t = -4. Die letzte Lösung ist nicht gültig, da \displaystyle x und \displaystyle y reell sein müssen (nach unserer Annahme). Wir erhalten also die Lösungen \displaystyle x=\pm\sqrt{1} und dadurch
- \displaystyle \ x=-1\ ergibt, dass \displaystyle \ y=-2/(-1)=2\,,
- \displaystyle \ x=1\ ergibt, dass \displaystyle \ y=-2/1=-2\,.
Also ist
| \displaystyle \sqrt{-3-4i} = \biggl\{\begin{align*} &\phantom{-}1-2i\,\mbox{,}\\ &-1+2i\,\mbox{.}\end{align*} |
Beispiel 16
- Löse die Gleichung \displaystyle \ z^2-2z+10=0\,.
Wir erhalten durch die allgemeine Lösungsformel (siehe Beispiel 12)\displaystyle z= 1\pm \sqrt{1-10} = 1\pm \sqrt{-9}= 1\pm 3i\,\mbox{.} - Löse die Gleichung \displaystyle \ z^2 + (4-2i)z -4i=0\,\mbox{.}
Wir verwenden wieder die Lösungsformel und erhalten\displaystyle \begin{align*} z &= -2+i\pm\sqrt{\smash{(-2+i)^2+4i}\vphantom{i^2}} = -2+i\pm\sqrt{4-4i+i^{\,2}+4i}\\ &=-2+i\pm\sqrt{3} = -2\pm\sqrt{3}+i\,\mbox{.}\end{align*} - Löse die Gleichung \displaystyle \ iz^2+(2+6i)z+2+11i=0\,\mbox{.}
Division auf beiden Seiten durch \displaystyle i ergibt\displaystyle \begin{align*} z^2 + \frac{2+6i}{i}z +\frac{2+11i}{i} &= 0\\ z^2+ (6-2i)z + 11-2i &= 0\,\mbox{.}\end{align*} Durch die Lösungsformel erhalten wir
\displaystyle \begin{align*} z &= -3+i \pm \sqrt{\smash{(-3+i)^2 -(11-2i)}\vphantom{i^2}}\\ &= -3+i \pm \sqrt{-3-4i}\\ &= -3+i\pm(1-2i)\,\mbox{.}\end{align*} indem wir das Beispiel 15 verwenden, um \displaystyle \ \sqrt{-3-4i}\ zu erhalten. Die Lösungen sind daher
\displaystyle z=\biggl\{\begin{align*} &-2-i\,\mbox{,}\\ &-4+3i\,\mbox{.}\end{align*}
Noch Fragen zu diesem Kapitel? Dann schau nach im Kursforum (Du findest den Link in der Student Lounge) oder frag nach per Skype bei ombTutor 

Keine Fragen mehr? Dann mache weiter mit den Übungen .
![[Image]](/wikis/2009/bridgecourse2-TU-Berlin/img_auth.php/metapost/6/b/9/6b9221f9f7b01fe26ab79402492dcd48.png)
