Processing Math: Done
Lösung 2.2:2d
Aus Online Mathematik Brückenkurs 2
(Unterschied zwischen Versionen)
K (Lösning 2.2:2d moved to Solution 2.2:2d: Robot: moved page) |
|||
| Zeile 1: | Zeile 1: | ||
| - | {{ | + | What makes the integral not entirely simple is the expression |
| - | < | + | <math>\text{1}-x</math> |
| - | {{ | + | under the root sign, so we try the substitution |
| + | <math>u=\text{1}-x</math>, | ||
| + | |||
| + | |||
| + | <math>\int\limits_{0}^{1}{\sqrt[3]{\text{1}-x}}\,dx=\left\{ \begin{matrix} | ||
| + | u=\text{1}-x \\ | ||
| + | du=\left( \text{1}-x \right)^{\prime }\,dx=-\,dx \\ | ||
| + | \end{matrix} \right\}=-\int\limits_{1}^{0}{\sqrt[3]{u}}\,du</math> | ||
| + | |||
| + | |||
| + | Note how the new limits of integration go from | ||
| + | <math>\text{1}</math> | ||
| + | to | ||
| + | <math>0</math> | ||
| + | (and not the other way around!). It is possible to change the order of the limits we change sign at the same time, i.e. | ||
| + | |||
| + | |||
| + | <math>-\int\limits_{1}^{0}{\sqrt[3]{u}}\,du=+\int\limits_{0}^{1}{\sqrt[3]{u}}\,du</math> | ||
| + | |||
| + | |||
| + | All that is now left is routine calculations: | ||
| + | |||
| + | |||
| + | <math>\begin{align} | ||
| + | & \int\limits_{0}^{1}{\sqrt[3]{u}}\,du=\int\limits_{0}^{1}{u^{\frac{1}{3}}}\,du=\left[ \frac{u^{\frac{1}{3}+1}}{\frac{1}{3}+1} \right]_{0}^{1} \\ | ||
| + | & =\frac{3}{4}\left[ u^{\frac{4}{3}} \right]_{0}^{1}=\frac{3}{4}\left( 1^{\frac{4}{3}}-0^{\frac{4}{3}} \right)=\frac{3}{4} \\ | ||
| + | \end{align}</math> | ||
Version vom 12:34, 20. Okt. 2008
What makes the integral not entirely simple is the expression
 10
10 31−xdx=
31−xdx=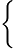 u=1−xdu=
u=1−xdu= 1−x
1−x
 dx=−dx
dx=−dx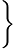 =−
=− 01
01 3udu
3udu
Note how the new limits of integration go from
 01
01 3udu=+
3udu=+ 10
10 3udu
3udu
All that is now left is routine calculations:
 10
10 3udu=
3udu= 10u31du=
10u31du=
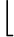 u31+131+1
u31+131+1
 10=43
10=43 u34
u34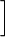 10=43
10=43 134−034
134−034 =43
=43
