Processing Math: Done
Lösung 1.2:4b
Aus Online Mathematik Brückenkurs 2
(Unterschied zwischen Versionen)
K (Solution 1.2:4b moved to Lösung 1.2:4b: Robot: moved page) |
|||
| Zeile 1: | Zeile 1: | ||
| - | + | Wir berechnen zuerst die erste Ableitung mit der Fakrorregel, | |
{{Abgesetzte Formel||<math>\begin{align} | {{Abgesetzte Formel||<math>\begin{align} | ||
| Zeile 7: | Zeile 7: | ||
\end{align}</math>}} | \end{align}</math>}} | ||
| - | + | Wir leiten leiten den zweiten Term, Term für Term mit der Kettenregel ab, | |
{{Abgesetzte Formel||<math>\begin{align} | {{Abgesetzte Formel||<math>\begin{align} | ||
| Zeile 16: | Zeile 16: | ||
\end{align}</math>}} | \end{align}</math>}} | ||
| - | + | Also haben wir | |
{{Abgesetzte Formel||<math>\begin{align} | {{Abgesetzte Formel||<math>\begin{align} | ||
| Zeile 24: | Zeile 24: | ||
\end{align}</math>}} | \end{align}</math>}} | ||
| - | + | Die zweite Ableitung ist | |
{{Abgesetzte Formel||<math>\begin{align} | {{Abgesetzte Formel||<math>\begin{align} | ||
Version vom 12:37, 19. Apr. 2009
Wir berechnen zuerst die erste Ableitung mit der Fakrorregel,
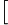 x(sinlnx+coslnx) x(sinlnx+coslnx) =(x) =(x)  (sinlnx+coslnx)+x (sinlnx+coslnx)+x (sinlnx+coslnx) (sinlnx+coslnx) =1 =1 (sinlnx+coslnx)+x (sinlnx+coslnx)+x (sinlnx+coslnx) (sinlnx+coslnx) . . |
Wir leiten leiten den zweiten Term, Term für Term mit der Kettenregel ab,
 =(sinlnx) =(sinlnx) +(coslnx) +(coslnx) =coslnx =coslnx (lnx) (lnx) −sinlnx −sinlnx (lnx) (lnx) =coslnx =coslnx x1−sinlnx x1−sinlnx x1. x1. |
Also haben wir
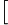 x(sinlnx+coslnx) x(sinlnx+coslnx) =sinlnx+coslnx+coslnx−sinlnx=2coslnx. =sinlnx+coslnx+coslnx−sinlnx=2coslnx. |
Die zweite Ableitung ist
 (lnx) (lnx) =−2sinlnx =−2sinlnx x1=−x2sinlnx. x1=−x2sinlnx. |
