Processing Math: Done
To print higher-resolution math symbols, click the
Hi-Res Fonts for Printing button on the jsMath control panel.
jsMath

Lösung 3.3:1a

Aus Online Mathematik Brückenkurs 2

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
K (Solution 3.3:1a moved to Lösung 3.3:1a: Robot: moved page)
Zeile 1: Zeile 1:
-
Powers are repeated multiplications and because multiplication is a relatively simple arithmetical operation when it is carried out in polar form, calculating powers also becomes fairly simple in polar form,
+
In Polarform haben wir den Moivreschen Satz,
{{Abgesetzte Formel||<math>\bigl(r(\cos\alpha + i\sin\alpha)\bigr)^n = r^n(\cos n\alpha + i\sin n\alpha)\,\textrm{.}</math>}}
{{Abgesetzte Formel||<math>\bigl(r(\cos\alpha + i\sin\alpha)\bigr)^n = r^n(\cos n\alpha + i\sin n\alpha)\,\textrm{.}</math>}}
-
The equation above is called de Moivre's formula.
+
Also bringen wir <math>1+i</math> auf Polarform, und verwenden den Moivreschen Satz, und schreiben die Potenz zuletzt wieder in der Form <math>a+ib</math>.
-
 
+
-
The plan is therefore to rewrite <math>1+i</math> in polar form, raise the expression to the power <math>12</math> using de Moivre's formula and then to write the answer in the form <math>a+ib</math>.
+
<center>[[Image:3_3_1_a1.gif]] [[Image:3_3_1_a_text.gif]]</center>
<center>[[Image:3_3_1_a1.gif]] [[Image:3_3_1_a_text.gif]]</center>
-
Using the calculations above, we see that
+
Durch die Rechnungen oben sehen wir dass
{{Abgesetzte Formel||<math>1+i = \sqrt{2}\Bigl(\cos\frac{\pi}{4} + i\sin\frac{\pi}{4} \Bigr)\,\textrm{.}</math>}}
{{Abgesetzte Formel||<math>1+i = \sqrt{2}\Bigl(\cos\frac{\pi}{4} + i\sin\frac{\pi}{4} \Bigr)\,\textrm{.}</math>}}
-
De Moivre's formula now gives
+
Und durch den Moivreschen Satz erhalten wir
{{Abgesetzte Formel||<math>\begin{align}
{{Abgesetzte Formel||<math>\begin{align}

Version vom 14:42, 18. Mai 2009

In Polarform haben wir den Moivreschen Satz,

r(cos+isin)n=rn(cosn+isinn). 

Also bringen wir 1+i auf Polarform, und verwenden den Moivreschen Satz, und schreiben die Potenz zuletzt wieder in der Form a+ib.

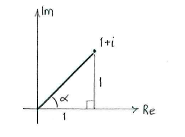
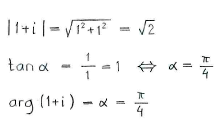
Image:3_3_1_a1.gif Image:3_3_1_a_text.gif

Durch die Rechnungen oben sehen wir dass

1+i=2cos4+isin4. 

Und durch den Moivreschen Satz erhalten wir

(1+i)12=212cos124+isin124=2(12)12cos3+isin3=26(−1+i0)=64(−1)=−64.
Von „http://wiki.math.se/wikis/2009/bridgecourse2-TU-Berlin/index.php/L%C3%B6sung_3.3:1a“