Lösung 3.3:1d

Aus Online Mathematik Brückenkurs 2

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
K (Solution 3.3:1d moved to Lösung 3.3:1d: Robot: moved page)
Zeile 1: Zeile 1:
-
Because we are going to raise something to the power 12, the base in the expression should be written in polar form. In turn, the base consists of a quotient which is advantageous to calculate in polar form. Thus, it seems appropriate to write
+
Wir bringen zuerst
-
<math>1+i\sqrt{3}</math> and <math>\text{1}+i</math> in polar form right from the beginning and to carry out all calculations in polar form.
+
<math>1+i\sqrt{3}</math> und <math>\text{1}+i</math> auf Polarform, und rechnen weiterhin in Polarform.
<center>[[Image:3_3_1_d.gif]] [[Image:3_3_1_d_text.gif]]</center>
<center>[[Image:3_3_1_d.gif]] [[Image:3_3_1_d_text.gif]]</center>
-
We obtain
+
Wir erhalten
{{Abgesetzte Formel||<math>\begin{align}
{{Abgesetzte Formel||<math>\begin{align}
Zeile 11: Zeile 11:
\end{align}</math>}}
\end{align}</math>}}
-
and
+
und also
{{Abgesetzte Formel||<math>\begin{align}
{{Abgesetzte Formel||<math>\begin{align}
Zeile 20: Zeile 20:
\end{align}</math>}}
\end{align}</math>}}
-
Finally, de Moivre's formula gives
+
Durch den Moivreschen Satz erhalten wir schließlich
{{Abgesetzte Formel||<math>\begin{align}
{{Abgesetzte Formel||<math>\begin{align}

Version vom 14:47, 18. Mai 2009

Wir bringen zuerst \displaystyle 1+i\sqrt{3} und \displaystyle \text{1}+i auf Polarform, und rechnen weiterhin in Polarform.

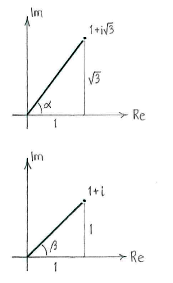
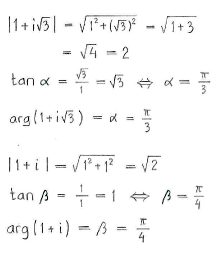
Image:3_3_1_d.gif Image:3_3_1_d_text.gif

Wir erhalten

\displaystyle \begin{align}

1+i\sqrt{3} &= 2\Bigl(\cos\frac{\pi}{3} + i\sin\frac{\pi}{3}\Bigr)\\[5pt] 1+i &= \sqrt{2}\Bigl(\cos\frac{\pi}{4} + i\sin\frac{\pi}{4}\Bigr) \end{align}

und also

\displaystyle \begin{align}

\frac{1+i\sqrt{3}}{1+i} &= \frac{2\Bigl(\cos\dfrac{\pi}{3} + i\sin\dfrac{\pi}{3}\Bigr)}{\sqrt{2}\Bigl(\cos\dfrac{\pi}{4} + i\sin\dfrac{\pi}{4}\Bigr)}\\[5pt] &= \frac{2}{\sqrt{2}}\Bigl(\cos\Bigl(\frac{\pi}{3}-\frac{\pi}{4}\Bigr) + i\sin\Bigl(\frac{\pi}{3}-\frac{\pi}{4}\Bigr)\Bigr)\\[5pt] &= \sqrt{2}\Bigl(\cos\frac{\pi}{12} + i\sin\frac{\pi}{12}\Bigr)\,\textrm{.} \end{align}

Durch den Moivreschen Satz erhalten wir schließlich

\displaystyle \begin{align}

\Bigl(\frac{1+i\sqrt{3}}{1+i}\Bigr)^{12} &= \bigl(\sqrt{2}\,\bigr)^{12}\Bigl(\cos\Bigl(12\cdot\frac{\pi}{12}\Bigr) + i\sin\Bigl(12\cdot\frac{\pi}{12}\Bigr)\Bigr)\\[5pt] &= 2^{(1/2)\cdot 12}(\cos\pi + i\sin\pi)\\[5pt] &= 2^6\cdot (-1+i\cdot 0)\\[5pt] &= -64\,\textrm{.} \end{align}

Von „http://wiki.math.se/wikis/2009/bridgecourse2-TU-Berlin/index.php/L%C3%B6sung_3.3:1d“