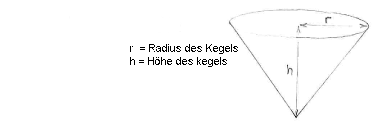Lösung 1.3:7
Aus Online Mathematik Brückenkurs 2
| Zeile 54: | Zeile 54: | ||
::Maximiere <math>V(\alpha) = \frac{1}{3}\pi R^3 \Bigl(\frac{2\pi-\alpha}{2\pi} \Bigr)^2\sqrt{1-\Bigl(\frac{2\pi-\alpha}{2\pi}\Bigr)^2}\,</math>, wo <math>0\le \alpha \le 2\pi\,</math>. | ::Maximiere <math>V(\alpha) = \frac{1}{3}\pi R^3 \Bigl(\frac{2\pi-\alpha}{2\pi} \Bigr)^2\sqrt{1-\Bigl(\frac{2\pi-\alpha}{2\pi}\Bigr)^2}\,</math>, wo <math>0\le \alpha \le 2\pi\,</math>. | ||
| - | Bevor wir anfangen die Funktion abzuleiten, sehen wir, dass | + | Bevor wir anfangen die Funktion abzuleiten, sehen wir, dass der Winkel |
<math>\alpha</math> nur in | <math>\alpha</math> nur in | ||
<math>(2\pi-\alpha)/2\pi</math>-Termen auftritt, um das Problem zu vereinfachen, können wir das Volumen genauso in Bezug auf die Variable <math>x=(2\pi-\alpha)/2\pi</math> maximieren. | <math>(2\pi-\alpha)/2\pi</math>-Termen auftritt, um das Problem zu vereinfachen, können wir das Volumen genauso in Bezug auf die Variable <math>x=(2\pi-\alpha)/2\pi</math> maximieren. | ||
| - | ::Maximiere <math>V(x) = \frac{1}{3}\pi R^3x^2\sqrt{1-x^2}\,</math>, | + | ::Maximiere <math>V(x) = \frac{1}{3}\pi R^3x^2\sqrt{1-x^2}\,</math>, für <math>0\le x\le 1\,</math>. |
| - | Wenn <math>x=0</math> oder <math>x=1</math>, ist | + | Wenn <math>x=0</math> oder <math>x=1</math> ist, ist das Volumen null, weil die Funktion überall (außer in <math>x=1</math>) differenzierbar ist, nimmt das Volumen sein Maximum in einem stationären Punkt an. |
Wir leiten die Funktion ab, | Wir leiten die Funktion ab, | ||
| Zeile 74: | Zeile 74: | ||
\end{align}</math>}} | \end{align}</math>}} | ||
| - | Die Ableitung ist null wenn <math>x=0</math> (dies ist | + | Die Ableitung ist null wenn <math>x=0</math> (dies ist ein Raandpunkt) oder wenn <math>2-3x^2=0</math> ist, also wenn <math>x=\sqrt{2/3}\,</math>. (Der Punkt <math>x=-\sqrt{2/3}</math> liegt außerhalb des Gebietes <math>0\le x\le 1</math>.) |
Durch einer Vorzeichentabelle erhalten wir die Vorzeichen der Faktoren, | Durch einer Vorzeichentabelle erhalten wir die Vorzeichen der Faktoren, | ||
| Zeile 137: | Zeile 137: | ||
|} | |} | ||
| - | Wir sehen hier dass <math>x=\sqrt{2/3}</math> ein globales | + | Wir sehen hier dass <math>x=\sqrt{2/3}</math> ein globales Maximum ist. <math>x = \sqrt{2/3}</math> entspricht den Winkel <math>\alpha</math>: |
| - | {{Abgesetzte Formel||<math>\sqrt{\frac{2}{3}}=\frac{2\pi-\alpha }{2\pi}\quad \Leftrightarrow\quad \alpha = 2\pi \bigl(1-\sqrt{2/3}\,\bigr)\ | + | {{Abgesetzte Formel||<math>\sqrt{\frac{2}{3}}=\frac{2\pi-\alpha }{2\pi}\quad \Leftrightarrow\quad \alpha = 2\pi \bigl(1-\sqrt{2/3}\,\bigr)\ </math>}} |
Version vom 10:50, 6. Aug. 2009
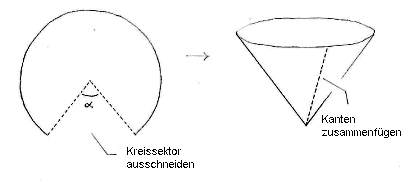
Wie der Kegel gebaut wird, ist hier illustriert:
Nachdem wir den Volumen des Kegels maximieren wollen, betrachten wir nun die Maße Kegels.
Mit diesen Maßen ist das Volumen des Kegels:
 (Höhe)=31 (Höhe)=31 r2h. r2h. |
Wir müssen jetzt den Radius 

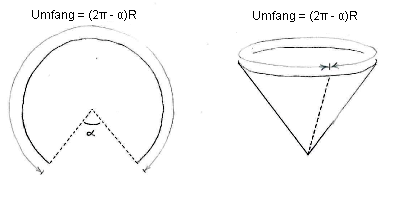
Schneiden wir einen Kreissektor mit dem Winkel 
 −
− )R
)R
Der Umfang des oberen Kreises ist aber auch
 r
r
 r=(2 r=(2 − − )R )R r=2 r=2 2 2 − − R. R. |
Jetzt haben wir den neuen Radius 
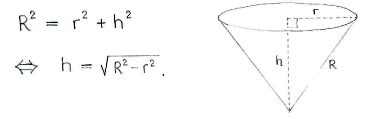
Um die Höhe zu bestimmen, benutzen wir den Satz des Pythagoras
Also haben wir
 R2− R2− 2 2 2 2 − − R R 2= 2= R2− R2− 2 2 2 2 − −  2R2=R 2R2=R 1− 1− 2 2 2 2 − −  2. 2. |
Jetzt haben wir den Radius 
 r2h=31 r2h=31  2 2 2 2 − − R R 2R 2R 1− 1− 2 2 2 2 − −  2=31 2=31 R3 R3 2 2 2 2 − −  2 2 1− 1− 2 2 2 2 − −  2. 2. |
Unser Problem ist jetzt:
- Maximiere
V( , wo )=31
)=31 R3
R3 2
2 2
2 −
−
 2
2 1−
1− 2
2 2
2 −
−
 2
2 0 .

 2
2
- Maximiere
Bevor wir anfangen die Funktion abzuleiten, sehen wir, dass der Winkel

 −
− )
) 2
2
 −
− )
) 2
2
- Maximiere
V(x)=31 , für R3x2
R3x2 1−x2
1−x2 0 . x
x 1
1
- Maximiere
Wenn
Wir leiten die Funktion ab,
 (x)=31 (x)=31 R3 R3 2x 2x  1−x2+31 1−x2+31 R3x2 R3x2 12 12 1−x2 1−x2 (−2x) (−2x) |
und vereinfachen den Ausdruck, indem wir so viele Faktoren wir möglich herausziehen,
 (x)=32 (x)=32 R3x R3x 1−x2−31 1−x2−31 R3x31 R3x31 1−x2=31 1−x2=31 R3x R3x 1−x2 1−x2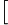 2(1−x2)−x2 2(1−x2)−x2 =31 =31 R3x R3x 1−x2(2−3x2). 1−x2(2−3x2). |
Die Ableitung ist null wenn  2
2 3
3  2
2 3
3  x
x 1
1
Durch einer Vorzeichentabelle erhalten wir die Vorzeichen der Faktoren,
| | |  32 32 | | ||
| | | | | | |
 1−x2 1−x2 | | | | | |
| | | | | | |
und erhalten dadurch das Vorzeichen der Ableitung selbst
| | |  32 32 | | ||
 (x) (x) | \displaystyle 0 | \displaystyle + | \displaystyle 0 | \displaystyle - | |
| \displaystyle V(x) | \displaystyle 0 | \displaystyle \nearrow | \displaystyle \tfrac{4}{9\sqrt{3}}\pi R^3 | \displaystyle \searrow | \displaystyle 0 |
Wir sehen hier dass \displaystyle x=\sqrt{2/3} ein globales Maximum ist. \displaystyle x = \sqrt{2/3} entspricht den Winkel \displaystyle \alpha:
| \displaystyle \sqrt{\frac{2}{3}}=\frac{2\pi-\alpha }{2\pi}\quad \Leftrightarrow\quad \alpha = 2\pi \bigl(1-\sqrt{2/3}\,\bigr)\ |