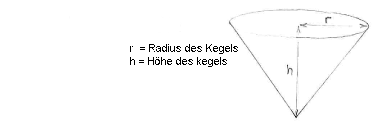Lösung 1.3:7
Aus Online Mathematik Brückenkurs 2
| Zeile 60: | Zeile 60: | ||
::Maximiere <math>V(x) = \frac{1}{3}\pi R^3x^2\sqrt{1-x^2}\ </math> , für <math>0\le x\le 1\,</math>. | ::Maximiere <math>V(x) = \frac{1}{3}\pi R^3x^2\sqrt{1-x^2}\ </math> , für <math>0\le x\le 1\,</math>. | ||
| - | Wenn <math>x=0</math> oder <math>x=1</math> ist, ist das Volumen null. Weil die Funktion überall (außer in <math>x=1</math>) differenzierbar ist, nimmt das Volumen sein Maximum | + | Wenn <math>x=0</math> oder <math>x=1</math> ist, ist das Volumen null. Weil die Funktion überall (außer in <math>x=1</math>) differenzierbar ist, nimmt das Volumen sein Maximum an einer stationären Stelle an. |
Wir leiten die Funktion ab | Wir leiten die Funktion ab | ||
| Zeile 74: | Zeile 74: | ||
\end{align}</math>}} | \end{align}</math>}} | ||
| - | Die Ableitung ist null, wenn <math>x=0</math> (dies ist ein Randpunkt) oder wenn <math>2-3x^2=0</math> ist, also wenn <math>x=\sqrt{2/3}\,</math>. ( | + | Die Ableitung ist null, wenn <math>x=0</math> (dies ist ein Randpunkt) oder wenn <math>2-3x^2=0</math> ist, also wenn <math>x=\sqrt{2/3}\,</math>. (Die Stelle <math>x=-\sqrt{2/3}</math> liegt außerhalb des Gebietes <math>0\le x\le 1</math>.) |
Durch eine Vorzeichentabelle erhalten wir die Vorzeichen der Faktoren | Durch eine Vorzeichentabelle erhalten wir die Vorzeichen der Faktoren | ||
| Zeile 137: | Zeile 137: | ||
|} | |} | ||
| - | Wir sehen hier, dass <math>x=\sqrt{2/3}</math> ein globales Maximum | + | Wir sehen hier, dass die Funktion an der Stelle <math>x=\sqrt{2/3}</math> ein globales Maximum hat. <math>x = \sqrt{2/3}</math> entspricht dem Winkel <math>\alpha</math>: |
{{Abgesetzte Formel||<math>\sqrt{\frac{2}{3}}=\frac{2\pi-\alpha }{2\pi}\quad \Leftrightarrow\quad \alpha = 2\pi \bigl(1-\sqrt{2/3}\,\bigr)\ </math>}} | {{Abgesetzte Formel||<math>\sqrt{\frac{2}{3}}=\frac{2\pi-\alpha }{2\pi}\quad \Leftrightarrow\quad \alpha = 2\pi \bigl(1-\sqrt{2/3}\,\bigr)\ </math>}} | ||
Aktuelle Version
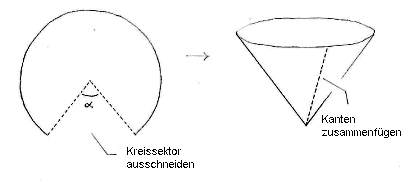
Wie der Kegel gebaut wird, ist hier illustriert.
Da wir das Volumen des Kegels maximieren wollen, betrachten wir nun die Abmessungen Kegels.
Mit diesen Abmessungen ist das Volumen des Kegels
 (Höhe)=31 (Höhe)=31 r2h. r2h. |
Wir müssen jetzt den Radius 

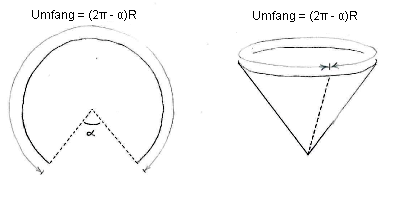
Schneiden wir einen Kreissektor mit dem Winkel 
 −
− )R
)R
Der Umfang des oberen Kreises ist aber auch
 r
r
 r=(2 r=(2 − − )R )R r=2 r=2 2 2 − − R. R. |
Jetzt haben wir den neuen Radius 
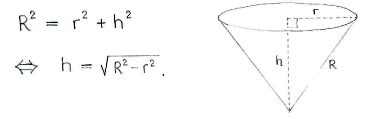
Um die Höhe zu bestimmen, benutzen wir den Satz des Pythagoras.
Also haben wir
 R2− R2− 2 2 2 2 − − R R 2= 2= R2− R2− 2 2 2 2 − −  2R2=R 2R2=R 1− 1− 2 2 2 2 − −  2. 2. |
Jetzt haben wir den Radius 
 r2h=31 r2h=31  2 2 2 2 − − R R 2R 2R 1− 1− 2 2 2 2 − −  2=31 2=31 R3 R3 2 2 2 2 − −  2 2 1− 1− 2 2 2 2 − −  2. 2. |
Unser Problem ist jetzt:
- Maximiere
V( , wo )=31
)=31 R3
R3 2
2 2
2 −
−
 2
2 1−
1− 2
2 2
2 −
−
 2
2 0 .

 2
2
- Maximiere
Bevor wir anfangen die Funktion abzuleiten, sehen wir, dass der Winkel

 −
− )
) 2
2
 −
− )
) 2
2
- Maximiere
V(x)=31 , für R3x2
R3x2 1−x2
1−x2 0 . x
x 1
1
- Maximiere
Wenn
Wir leiten die Funktion ab
 (x)=31 (x)=31 R3 R3 2x 2x  1−x2+31 1−x2+31 R3x2 R3x2 12 12 1−x2 1−x2 (−2x) (−2x) |
und vereinfachen den Ausdruck, indem wir so viele Faktoren wir möglich herausziehen.
 (x)=32 (x)=32 R3x R3x 1−x2−31 1−x2−31 R3x31 R3x31 1−x2=31 1−x2=31 R3x R3x 1−x2 1−x2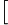 2(1−x2)−x2 2(1−x2)−x2 =31 =31 R3x R3x 1−x2(2−3x2) 1−x2(2−3x2) |
Die Ableitung ist null, wenn  2
2 3
3  2
2 3
3  x
x 1
1
Durch eine Vorzeichentabelle erhalten wir die Vorzeichen der Faktoren
| | |  32 32 | | ||
| | | | | | |
 1−x2 1−x2 | \displaystyle + | \displaystyle + | \displaystyle + | \displaystyle + | \displaystyle 0 |
| \displaystyle 2-3x^2 | \displaystyle + | \displaystyle + | \displaystyle 0 | \displaystyle - | \displaystyle - |
und erhalten dadurch das Vorzeichen der Ableitung selbst.
| \displaystyle x | \displaystyle 0 | \displaystyle \sqrt{\tfrac{2}{3}} | \displaystyle 1 | ||
| \displaystyle V'(x) | \displaystyle 0 | \displaystyle + | \displaystyle 0 | \displaystyle - | |
| \displaystyle V(x) | \displaystyle 0 | \displaystyle \nearrow | \displaystyle \tfrac{4}{9\sqrt{3}}\pi R^3 | \displaystyle \searrow | \displaystyle 0 |
Wir sehen hier, dass die Funktion an der Stelle \displaystyle x=\sqrt{2/3} ein globales Maximum hat. \displaystyle x = \sqrt{2/3} entspricht dem Winkel \displaystyle \alpha:
| \displaystyle \sqrt{\frac{2}{3}}=\frac{2\pi-\alpha }{2\pi}\quad \Leftrightarrow\quad \alpha = 2\pi \bigl(1-\sqrt{2/3}\,\bigr)\ |