Lösung 1.3:1a

Aus Online Mathematik Brückenkurs 2

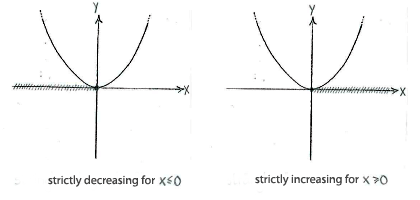
A critical point is a point where the derivative is equal to zero, i.e. the function has a horizontal tangent. For the function in the exercise, this occurs when x=0.

In addition, the point at the origin is a local and global minimum, because no other points give a smaller value for the function that the point at x=0. On the other hand, there are no inflexion points (points where the derivative is both zero and has the same sign on both sides of the point).

To the left of x=0, the derivative is negative (the tangent slopes downwards) and the function is strictly decreasing, and to the right of x=0 the derivative ) the tangent slopes upwards) and the function is strictly increasing.