Lösung 1.3:6
Aus Online Mathematik Brückenkurs 2
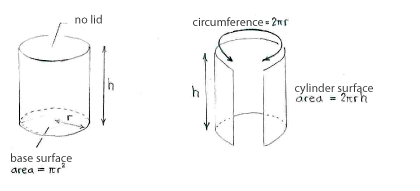
If we call the radius of the metal can
Volume = (area of the base). (height)
 r2h
r2h
Area= (area of the base)+(area of the cylindrical surface)
 r2+2
r2+2 h
h
The problem can then be formulated as: minimise the can's area,
 r2+2
r2+2 h
h r2h
r2h
From the formula for the volume, we can make
 r2
r2
and express the area solely in terms of the radius,
 r2+2
r2+2 r
r V
V r2=
r2= r2+r2V
r2+r2V
The minimisation problem is then:
to minimise the area
 r
r =
= r2+r2V
r2+r2V  0
0
The area function
 r
r
 0
0 0
0 0
0
The derivative is given by

 r
r =2
=2 r−r22V
r−r22V
and if we set the derivative equal to zero, so as to obtain the critical points, we get
 r−r22V=0
r−r22V=0 2
2 r=r22V
r=r22V r3=
r3= V
V r=
r= 3
3 V
V
For this value of


 r
r =2
=2 +r34V
+r34V


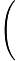
 3
3 V
V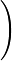 =2
=2 +
+ V4V=6
V4V=6
 0
0
which shows that
 3V
3V

Because the region of definition,
 0
0 3V
3V

 0
0
 0
0
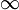
 3V
3V

The metal can has the least area for a given volume
\displaystyle r=\sqrt[3]{{V}/{\pi }\;}, and
\displaystyle h=\frac{V}{\pi r^{2}}=\frac{V}{\pi }\left( \frac{V}{\pi } \right)^{-{2}/{3}\;}=\left( \frac{V}{\pi } \right)^{1-{2}/{3}\;}=\left( \frac{V}{\pi } \right)^{{1}/{3}\;}=\sqrt[3]{\frac{V}{\pi }}