Processing Math: Done

Aus Online Mathematik Brückenkurs 2

If we use w=z−1 as a new unknown and move the term 4 over to the right-hand side, we have a binomial equation,

w4=−4

We can solve this equation in the usual way by using polar form and de Moivre's formula. We have

w=rcos+isin−4=4cos+isin 

and the equation becomes

r4cos4+isin4=4cos+isin 

The only way that both sides can be equal is if the magnitudes agree and the arguments do not differ by anything other than a multiple of 2,

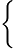r4=44=+2nn an arbitrary integer  

which gives us that

r=42=2=4+2nn an arbitrary integer  

for n=0 1 2 and 3, the argument assumes the four different values

4 43 45 and 47

and for different values of n we obtain values of which are equal to those above, apart from multiples of 2. Thus, we have four solutions,

w=2cos4+isin42cos34+isin342cos54+isin542cos74+isin74=1+i−1+i−1−i1−i

and the original variable z is

z=2+ii−i2−i