Processing Math: Done

Aus Online Mathematik Brückenkurs 2

Let us first divide both sides by 4+i, so that the coefficient in front of z2 becomes 1,

z2+4+i1−21iz=174+i

The two complex quotients become

4+i4−i1−21i4−i=42−i24−i−84i+21i2=16+1−17−85i=17−17−85i=−1−5i

174+i=174−i4+i4−i=42−i2174−i=17174−i=4−i

Thus, the equation becomes

z2−1+5iz=4−i 

Now, we complete the square of the left-hand side:

z−21+5i2−21+5i2=4−iz−21+5i2−41+25i+425i2=4−iz−21+5i2−41−25i+425=4−iz−21+5i2=−2+23i

If we set w=z−21+5i, we have a binomial equation in w,

w2=−2+23i

which we solve by putting w=x+iy ,

x+iy2=−2+23i 

or, if the left-hand side is expanded,

x2−y2+2xyi=−2+23i

If we identify the real and imaginary parts on both sides, we get

x2−y2=−22xy=23

and if we take the magnitude of both sides and put them equal to each other, we obtain a third relation:

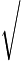
x2+y2=−22+232=25 

Strictly speaking, this last relation is contained within the first two and we include it because makes the calculations easier.

Together, the three relations constitute the following system of equations:

 x2−y2=2 2xy=−23 x2+y2=25

From the first and the third equations, we can relatively easily obtain the values that x and y can take.

Add the first and third equations,

EQ1

which gives that x=21.

Then, subtract the first equation from the third equation,

EQ13

i.e. y=23.

This gives four possible combinations,

x=21y=23x=21y=−23x=−21y=23x=−21y=−23 

of which only two also satisfy the second equation.

x=21y=23andx=−21y=−23 

This means that the binomial equation has the two solutions,

w=21+23i and w=1−2−23i

and that the original equation has the solutions

z=1+4i and z=i

according to the relation w=z−21+5i.

Finally, we check that the solutions really do satisfy the equation.

1+4i:4+iz2+1−21iz=4+i1+4i2+1−21i1+4i=4+i1+8i+16i2+1+4i−21i−84i2=4+i−15+8i+1−17i+84=−60+32i−15i+8i2+1−17i+84=−60+32i−15i−8+1−17i+84=17

z=i:4+iz2+1−21iz=4+ii2+1−21ii=4+i−1+i−21i2=−4−i+i+21=17