Processing Math: Done
To print higher-resolution math symbols, click the
Hi-Res Fonts for Printing button on the jsMath control panel.
No jsMath TeX fonts found -- using image fonts instead.
These may be slow and might not print well.
Use the jsMath control panel to get additional information.
jsMath Control PanelHide this Message

jsMath

Lösung 3.2:6f

Aus Online Mathematik Brückenkurs 2

Wechseln zu: Navigation, Suche

Schreiben wir den Zähler und Nenner in Polarform, koönnen wir die Divisions- und Multilpikationsregeln für komplexe Zahlen in Polarform verwenden:

  • r1(cos+isin)r2(cos+isin)=r1r2cos(+)+isin(+) 
  • r2(cos+isin)r1(cos+isin)=r2r1cos(−)+isin(−). 

Wir schreiben die Zahlen also in Polarform:

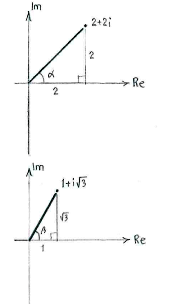
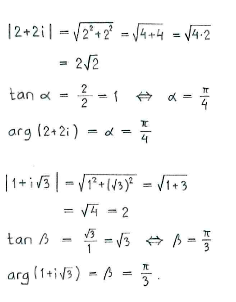
Image:3_2_6_f1_bild.gif Image:3_2_6_f1_bildtext.gif
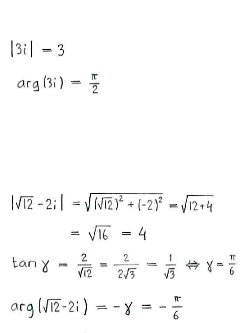
Image:3_2_6_f2_bild.gif Image:3_2_6_f2_bildtext.gif

und erhalten den Ausdruck

3i(12−2i)(2+2i)(1+i3)=22cos4+isin42cos3+isin33cos2+isin24cos−6+isin−6=12cos2−6+isin2−642cos4+3+isin4+3=12cos3+isin342cos127+isin127=1242cos127−3+isin127−3=32cos4+isin4.
Von „http://wiki.math.se/wikis/2009/bridgecourse2-TU-Berlin/index.php/L%C3%B6sung_3.2:6f“