Processing Math: Done
Lösung 2.1:2b
Aus Online Mathematik Brückenkurs 2
Erweitern wir zuerst den Ausdruck, erhalten wir
 2−1(x−2)(x+1)dx= 2−1(x−2)(x+1)dx= 2−1(x2+x−2x−2)dx= 2−1(x2+x−2x−2)dx= 2−1(x2−x−2)dx 2−1(x2−x−2)dx |
und wir erhalten das Integral
 2−1(x2−x1−2x0)dx. 2−1(x2−x1−2x0)dx. |
Wir sehen dass der Integrand aus Termen auf der Form
 2−1(x2−x1−2x0)dx= 2−1(x2−x1−2x0)dx= 3x3−2x2−2 3x3−2x2−2 x1 x1 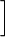 2−1=323−222−2 2−1=323−222−2 12− 12− 3(−1)3−2(−1)2−2 3(−1)3−2(−1)2−2 1(−1) 1(−1) =38−24−4− =38−24−4− −31−21+2 −31−21+2 =616−12−24+2+3−12=−627=−29. =616−12−24+2+3−12=−627=−29. |
