14. Exercises
From Mechanics
| Theory | Exercises | Video |
Exercise14.1
A uniform beam of mass 20 kg and length 3m rests on two supports as shown below.
(a) Find the reaction force exerted by each support.
(b) Find the greatest mass that can be placed at the left hand end of the beam.
Exercise 14.2
A plank has length 6 m. The plank is placed on a quayside with its mid-point at the edge of the quay, so that half of the plank sticks out over the water. A rock of mass m kg, which should be modeled as a particle is placed at the end of the plank which is on the ground. A person of mass 60 kg walks on the part of plank that is over the water. When the person is 1 m from the end the plank is on the point of tipping. Find m.
Exercise 14.3
A uniform beam has mass 20 kg and length 6 m. It rests on two supports that are 1.5 m from each end of the beam. A concrete block of mass 5 kg is placed at a point on the beam at a distance of 2.5 m from one end. Find the magnitude of the reaction forces exerted on the beam by the two supports.
Exercise 14.4
A uniform rod of mass 18 kg and length 6m rests on two supports.
a) Find the magnitude of each force exerted on the rod by the supports.
A particle is attached to the rod at the right hand end.
b) What is the greatest possible mass of the particle if the rod remains in equilibrium?
Exercise 14.5
A metal beam, of mass 6 kg and length 2m, rests in a horizontal position on two supports that are at a distance of 40 cm from each end of the beam. A 1.2 kg mass is placed at one end of the beam.
a) Find the magnitude of the reaction forces acting on the beam.
b) What is the greatest mass that could be placed at the other end of the beam, if it is to remain in equilibrium?
Exercise 14.6
A ladder, of length 5 m and mass 25 kg, leans against a smooth wall so that it is at an angle of 70
Exercise 14.7
A ladder, of mass 20 kg and length 5 m, has its base on rough, horizontal ground and rests against a smooth vertical wall. The coefficient of friction between the ground and the ladder is 0.6. The angle between the ladder and the ground is 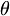
a) Find the magnitude of the friction force acting on the ladder in terms of 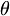
b) Find the smallest value of 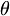
 Loading...
Loading...