20. Exercises
From Mechanics
| Theory | Exercises | Video |
Exercise 20.1
A particle, of mass 2 kg, is attached to a fixed point on a smooth horizontal table by a light inextensible string of length 50 cm. The particle travels in a circle on the table at 400 r.p.m. Find the tension in the string.
Exercise 20.2
A marble is made to rotate against the outside rim of a circular tray of radius 0.2 m. The mass of the marble is 100 grams and it moves at 2
Exercise 20.3
A car, of mass 1 tonne, takes a bend, of radius 50 m, on a level road, at 60 km/h, without sliding. Find the frictional force between the tyres and the ground. What is the least value of 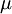
Exercise 20.4
A coin, of mass 20 grams, is placed on a horizontal turntable which rotates at 30 r.p.m. The coin is 5 cm from the centre of rotation. The coefficient of friction between the coin and the turntable is 0.6.
a) Does the coin remain in the same position on the turntable or does it slide?
b) What is the greatest distance from the centre of rotation that the coin can be placed, without slipping?
c) Would your answers to parts a) and b) change for a heavier coin? Explain why.
Answer a | Answer b | Answer c | Solution a | Solution b | Solution c
Exercise 20.5
A van, of mass 1500 kg travels around a bend of radius 80 m. The van travels at a constant speed of 15
a) Find the magnitude of the friction force on the van.
b) Find the least possible value of the coefficient of friction.
Answer a | Answer b | Solution a | Solution b
Exercise 20.6
A horizontal bend on the road has a radius of 140 m. The coefficient of friction between a road surface and the tyres of a car is 0.8. The mass of the car is 1200 kg.
a) Find the maximum speed at which the car can travel round the bend without sliding.
b) Find the magnitude of the friction force in this case
Answer a | Answer b | Solution a | Solution b
 Loading...
Loading...