From Mechanics

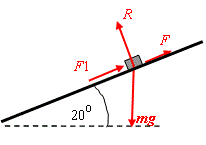
In the figure \displaystyle F1 is the air resistance.

Resolving up the plane

\displaystyle \begin{align} & F1+F-mg\sin {{20}^{\circ }}=0 \\ & \\ & F1=mg\sin {{20}^{\circ }}-F=60\times 9\textrm{.}81\times 0\textrm{.}342-F=201\textrm{.}3\ \text{N}-F \\ \end{align}

Resolving perpendicular to the plane upwards

\displaystyle \begin{align} & R-mg\cos {{20}^{\circ }}=0 \\ & \\ & R=mg\cos {{20}^{\circ }}=60\times 9\textrm{.}81\times 0\textrm{.}94=553\ \text{N} \\ \end{align}

Using the friction equation gives

\displaystyle F=\mu R=0\textrm{.}05\times 553=27\textrm{.}65\ \text{N}

Substituting in the above equation for \displaystyle R1 gives

\displaystyle F1=201\textrm{.}3-27\textrm{.}65\approx 173\ \text{N}